The distance travelled by a motor car in t second after the brakes are applied is s feet, where s = 22t . The distance travelled by the car before it stops, is
10.08 ft
10 ft
11 ft
11.5 ft
The correct Answer is:A
To solve the problem, we need to find the distance traveled by a motor car after the brakes are applied, given the equation for distance s=22t−12t2. The car stops when its velocity becomes zero.
1. Understand the Problem: We need to find the total distance traveled by the car before it stops. The distance s is given as a function of time t.
2. Differentiate the Distance Function: To find when the car stops, we need to find the velocity, which is the derivative of the distance with respect to time. Thus, we differentiate s:
dsdt=ddt(22t−12t2)
Using the power rule, we get:
dsdt=22−24t
3. Set the Velocity to Zero: The car stops when the velocity is zero:
22−24t=0
4. Solve for Time t: Rearranging the equation gives:
24t=22⟹t=2224=1112 seconds
5. Substitute t Back into the Distance Equation: Now we substitute t=1112 back into the distance equation to find the total distance traveled:
s=22(1112)−12(1112)2
Calculating each term:
s=22×1112−12×121144
Simplifying:
s=24212−12112
s=242−12112=12112
6. Convert to Decimal: To find the numerical value:
s=12112≈10.0833 feet
Thus, the distance traveled by the car before it stops is approximately 10.08 feet.
dsdt=ddt(22t−12t2)
Using the power rule, we get:
dsdt=22−24t
22−24t=0
24t=22⟹t=2224=1112 seconds
s=22(1112)−12(1112)2
Calculating each term:
s=22×1112−12×121144
Simplifying:
s=24212−12112
s=242−12112=12112
s=12112≈10.0833 feet
Topper's Solved these Questions
- DERIVATIVE AS A RATE MEASURER BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DERIVATIVE AS A RATE MEASURER EXERCISE - Section I - Solved Mcqs
27 Videos
- DERIVATIVE AS A RATE MEASURER BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DERIVATIVE AS A RATE MEASURER EXERCISE - Exercise
26 Videos
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DEFINITE INTEGRALSEXERCISE - Chapter Test 2
56 Videos
- DIFFERENTIAL EQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Chapter Test
30 Videos
Similar Questions
A car travels with a uniform velocity of 25 m s−1 for 5 s. The brakes are then applied and the car is uniformly retarded and comes to rest in further 10 s. Find : (i) the distance which the car travels before the brakes are applied, (ii) the retardation,and (iii) the distance travelled by the car after applying the brakes.
View SolutionA car travels for 20 min with a constant speed of 54 km h−1 Find the distance travelled by the car.
View Solution
Knowledge Check
In the given v-t graph the distance travelled by the body in 5 seconds will be
 A100 mB80 mC40 mD20 m
A100 mB80 mC40 mD20 m
A car travels with a uniform velocity of 20 m s−1 for 5 s. The brakes are then applied and the car is uniformly retarded. It comes to rest in further 8 s. Draw a graph of velocity against time. Use this graph to find :
(i) the distance travelled in first 5 s,
(ii) the distance travelled after the brakes are applied,
(iii) total distance travelled, and
(iv) acceleration during the first 5 s and last 8 s.
View SolutionThe position of a particle along x-axis at time t is given by x=1+t−t2. The distance travelled by the particle in first 2 seconds is
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
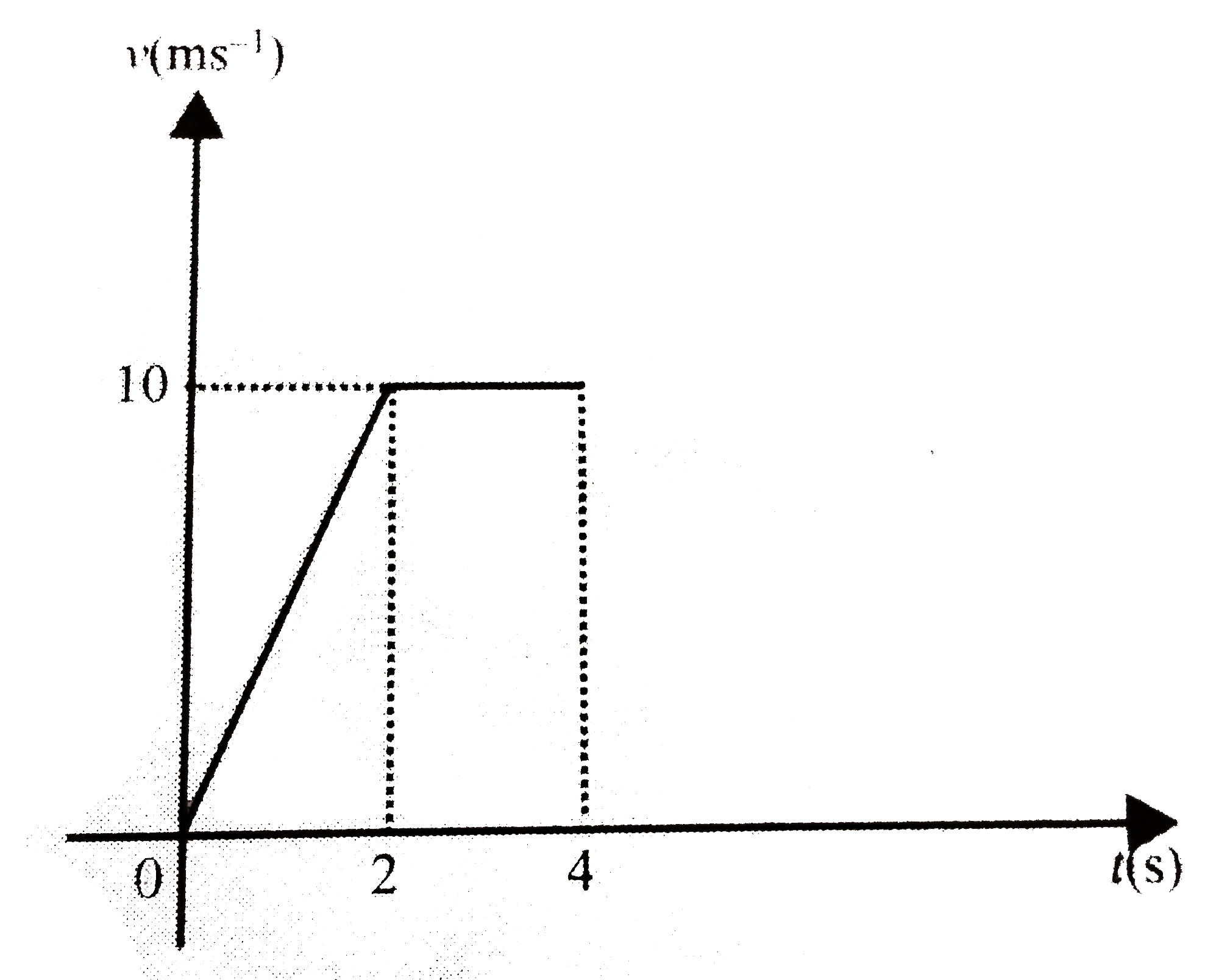
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionA particle moves on x-axis as per equation x=(t3−9t2+15+2)m. Distance travelled by the particle between t=0 and t=5s is
View SolutionA driver takes 0.20 s to apply the brakes after he sees a need for it. This is called the reaction time of the driver. If he ils driving a car at a speed of 54 km/h and the brakes cause a deceleration of 6.0ms2, find the distance travelled by the car after he sees the need to put the brakes on.
View SolutionA motor car is moving with a velocity of 108km/h and it takes 4second to stop after the brakes are applied. Calculate the force exerted by the brakes on the motorcar if its mass along with the passenger is 1000kg.
View SolutionThe distance travelled by a particle in a straight line motion is directly proportional to t1/2, where t is the time elapsed.
View Solution
