A vessel containing mercury has a hole at its bottom. The diameter of the hole is 60×10−6m. Find the maximum height of mercury in the vessel so that it does not flow out through the hole. Surface tension of mercury is 54×10−2N/m.
To find the maximum height of mercury in the vessel so that it does not flow out through the hole, we can use the relationship between surface tension, pressure, and height of the liquid column.
1. Identify the given values:
- Diameter of the hole, d=60×10−6m
- Surface tension of mercury, T=54×10−2N/m
- Density of mercury, ρ=13.6×103kg/m3
- Acceleration due to gravity, g=9.8m/s2
2. Calculate the radius of the hole:
R=d2=60×10−62=30×10−6m
3. Use the formula for the pressure due to surface tension:
The pressure difference created by the surface tension at the hole is given by:
P=2TR
4. Substitute the values into the pressure formula:
P=2×54×10−230×10−6=108×10−230×10−6=10830×104Pa
P=3.6×104Pa
5. Relate the pressure to the height of the mercury column:
The gauge pressure due to a column of liquid is given by:
P=ρgh
6. Set the two expressions for pressure equal to each other:
ρgh=2TR
7. Rearrange the equation to solve for height h:
h=2TρgR
8. Substitute the known values into the height formula:
h=2×54×10−2(13.6×103)×(9.8)×(30×10−6)
9. Calculate the height h:
h=108×10−2(13.6×103)×(9.8)×(30×10−6)
h=108×10−2(13.6×9.8×30)×10−3
h=10813.6×9.8×30×101
h≈0.27m
Final Answer:
The maximum height of mercury in the vessel so that it does not flow out through the hole is approximately 0.27m.
- Diameter of the hole, d=60×10−6m
- Surface tension of mercury, T=54×10−2N/m
- Density of mercury, ρ=13.6×103kg/m3
- Acceleration due to gravity, g=9.8m/s2
R=d2=60×10−62=30×10−6m
The pressure difference created by the surface tension at the hole is given by:
P=2TR
P=2×54×10−230×10−6=108×10−230×10−6=10830×104Pa
P=3.6×104Pa
The gauge pressure due to a column of liquid is given by:
P=ρgh
ρgh=2TR
h=2TρgR
h=2×54×10−2(13.6×103)×(9.8)×(30×10−6)
h=108×10−2(13.6×103)×(9.8)×(30×10−6)
h=108×10−2(13.6×9.8×30)×10−3
h=10813.6×9.8×30×101
h≈0.27m
The maximum height of mercury in the vessel so that it does not flow out through the hole is approximately 0.27m.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 3 (SOLVED EXAMPLES)31videos
PROPERTIES OF MATTER
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 (SOLVED EXAMPLES)11videos
PROPERTIES OF MATTER
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS12videos
PROPERTIES OF MATTER
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)2videos
OSCILLATIONS
- BOOK - ICSECHAPTER - SAMPLE QUESTION PAPER - 01 EXERCISE - SECTION - D12videos
SAMPLE QUESTION PAPER - 01
Similar Questions
In the bottom of a vessel with mercury of density ρ there is a round hole of radius r. At what maximum height of the mercury layer will the liquid still not flow out through this hole. (Surface tension = T)–
View SolutionThere is a circular hole of diameter d=140μm at the bottom of a vessel containing mercury . The minimum height of mercury layer so that the mercury will not flow out of this hole is
( Surface tension, σ=490×10−3Nm−1)View SolutionA mercury drop of radius 1.0cm is sprayed into 108 droplets of equal size. Calculate the energy expanded. (Surface tension of mercury =32×10−2N/m).
View SolutionA menisus drop of radius 1 cm sprayed into 105 droplets of equal size . Calculate the energy expended if surface tension of mercury is 435×10−3 N/m
View SolutionA mercury drop of radius 1 cm is sprayed into 106 droplets of equal size. Calculate the energy expended if surface tension of mercury is 35×10−3N/m .
View SolutionA small hollow sphere having a small hole in it is immersed in water to a depth of 50cm, before any water penetrates into it. Calculate the radius of the hole, if the surface tension of water is 7.2×10−2Nm−1.
View SolutionMercury in a capillary tube is depressed by 1.16cm. The diameter of the capillary tube is 1mm. Calculate the angle of contact of mercury with the glass if the surface tension of mercury is 54×10Nm−1 and its density is 13.6×103kgm−3.
View SolutionTwo communicating vessels contain mercury. The diameter of one vessel in n times larger then the diameter of the other. A column of water of height h is poured into the left vessel. The mercury level will rise in the right hand vessel (s = relative density of mercury and ρ = density of water) by
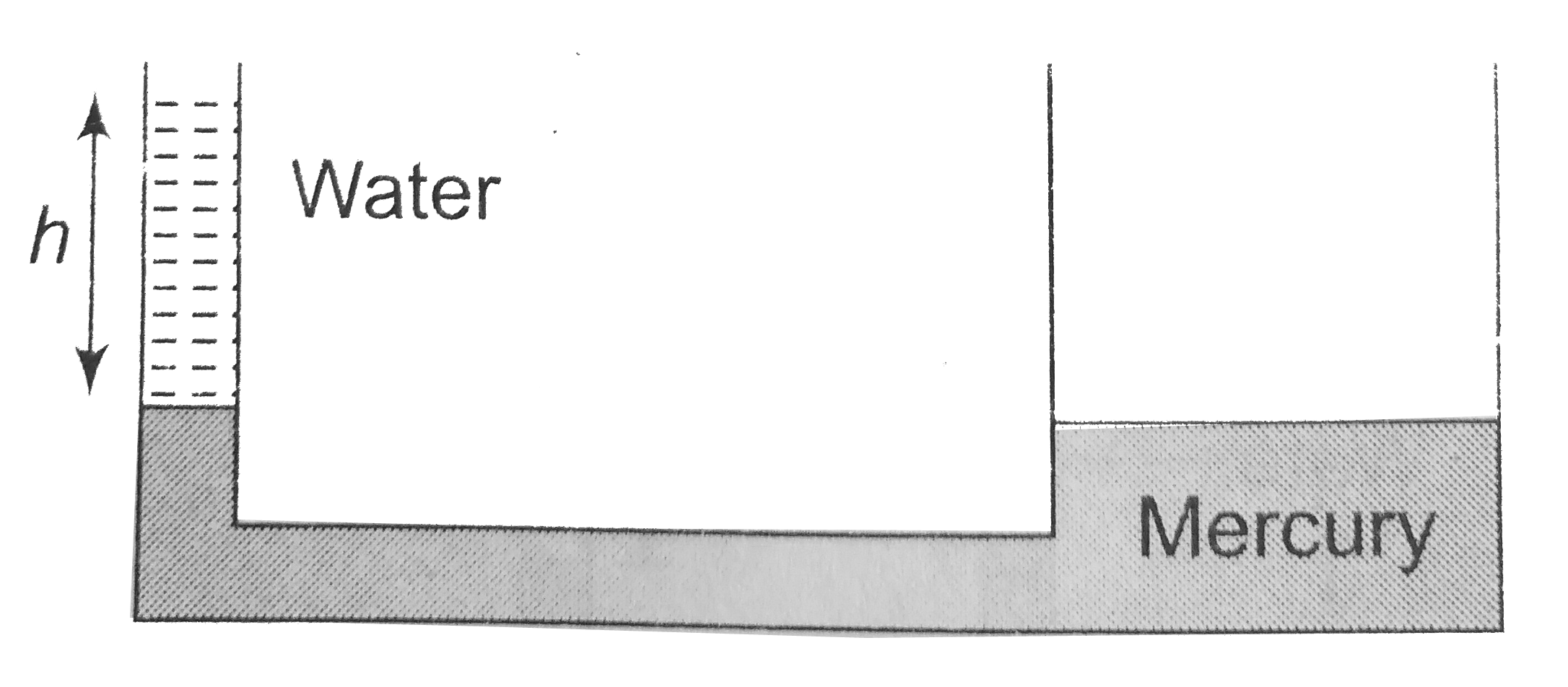 View Solution
View SolutionAn open capillary tube is lowered in vessel with mercury. The difference between the levels of the mecury in the vessel and in the capillary tube △h=4.6mm. What is the radius of curvature of the mercury meniscus in the capillary tube? Surface tension of mercury is 0.46N/m, density of mercury is 13.6gm/cc.
View SolutionA vessel whose bottom has round holes with a diameter of d=0.1mm is filled with water. The maximum height of the water level h at which the water does not flow out, will be (The water does not wet the bottom of the vessel). [STofwater=70dyne/cm]
View Solution

