In the bottom of a vessel with mercury of density ρ there is a round hole of radius r. At what maximum height of the mercury layer will the liquid still not flow out through this hole. (Surface tension = T)–
Trρg
T2rρg
2Trρg
4Trρg
The correct Answer is:C
To solve the problem of determining the maximum height of the mercury layer that will not flow out through a hole at the bottom of a vessel, we can follow these steps:
Step 1: Understand the forces acting on the liquid
The liquid (mercury) experiences two main forces:
1. The downward pressure due to the height of the mercury column.
2. The upward force due to surface tension at the hole.
Step 2: Define the pressure at the hole
At a height h of the mercury, the pressure at the hole can be expressed as:
PA=Patm+ρgh
where:
- PA is the pressure at the hole,
- Patm is the atmospheric pressure,
- ρ is the density of mercury,
- g is the acceleration due to gravity,
- h is the height of the mercury column.
Step 3: Calculate the upward force due to surface tension
The surface tension force acting on the liquid at the hole can be calculated using:
Fs=T⋅L
where:
- T is the surface tension,
- L is the length around the hole, which is the circumference of the hole, L=2πr.
Thus, the surface tension force becomes:
Fs=T⋅2πr
Step 4: Calculate the upward pressure force
The upward force due to the pressure difference can be expressed as:
Fp=(Patm−PA)⋅A
where A is the area of the hole, given by A=πr2. Therefore:
Fp=(Patm−(Patm+ρgh))⋅πr2=−ρgh⋅πr2
Step 5: Set the forces equal for equilibrium
For the liquid to not flow out, the upward surface tension force must balance the downward pressure force:
Fs=|Fp|
This gives us:
T⋅2πr=ρgh⋅πr2
Step 6: Simplify the equation
Cancel πr from both sides:
2T=ρghr
Step 7: Solve for height h
Rearranging the equation to find h:
h=2Tρgr
Final Answer
Thus, the maximum height of the mercury layer that will not flow out through the hole is:
h=2Tρgr
The liquid (mercury) experiences two main forces:
1. The downward pressure due to the height of the mercury column.
2. The upward force due to surface tension at the hole.
At a height h of the mercury, the pressure at the hole can be expressed as:
PA=Patm+ρgh
where:
- PA is the pressure at the hole,
- Patm is the atmospheric pressure,
- ρ is the density of mercury,
- g is the acceleration due to gravity,
- h is the height of the mercury column.
The surface tension force acting on the liquid at the hole can be calculated using:
Fs=T⋅L
where:
- T is the surface tension,
- L is the length around the hole, which is the circumference of the hole, L=2πr.
Fs=T⋅2πr
The upward force due to the pressure difference can be expressed as:
Fp=(Patm−PA)⋅A
where A is the area of the hole, given by A=πr2. Therefore:
Fp=(Patm−(Patm+ρgh))⋅πr2=−ρgh⋅πr2
For the liquid to not flow out, the upward surface tension force must balance the downward pressure force:
Fs=|Fp|
This gives us:
T⋅2πr=ρgh⋅πr2
Cancel πr from both sides:
2T=ρghr
Rearranging the equation to find h:
h=2Tρgr
Thus, the maximum height of the mercury layer that will not flow out through the hole is:
h=2Tρgr
Similar Questions
A vessel containing mercury has a hole at its bottom. The diameter of the hole is 60×10−6m. Find the maximum height of mercury in the vessel so that it does not flow out through the hole. Surface tension of mercury is 54×10−2N/m.
View SolutionThere is a circular hole of diameter d=140μm at the bottom of a vessel containing mercury . The minimum height of mercury layer so that the mercury will not flow out of this hole is
( Surface tension, σ=490×10−3Nm−1)
View Solution
Knowledge Check
A cylinider is filled with non viscous liquid of density d to a height h0 and a hole is made at a height h1 from the bottom of the cylinder. The velocity issuing out of the hole is
A√2gh0B√2g(h0−h1)C√dgh1D√dgh0
A vessel whose bottom has round holes with diameter of 1 mm is filled with water Assuming that surface tension acts only at holes, then the maximum height to which the water can be filled in vessel without leakage is (given surface tension of water is 75×10−3N/m) and g=10m/s2
View SolutionA paper disc of radius R from which a hole of radius r is cut out is floating in a liquid of the surface tension S. The force on the disc due to the surface tension is
View SolutionA vessel whose bottom has round holes with a diameter of d=0.1mm is filled with water. The maximum height of the water level h at which the water does not flow out, will be (The water does not wet the bottom of the vessel). [STofwater=70dyne/cm]
View SolutionMercury has an angle of contact of 120∘ with glass. A narrow tube of radius 1.0mm made of the glass is dipped in a through containing mercury. By what amount does the mercury dip down in the tube relative to the liquid surface outside. Surface tension of mercury at the temperature of the experiment os 0.5N/m and density of mercury is 13.6×103kg/m3.
(Take g=9.8m/s2).
View SolutionOn heating water, bubbles being formed at the bottom of the vessel detach and rise. Take the bubbles to be spheres of radius R and making a circular contact of radius r with the bottom of the vessel. If r<<R and the surface tension of water is T, value of r just before bubbles detach is: (density of water is ρw)
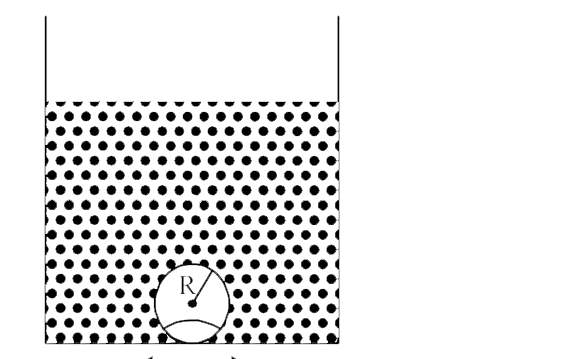
View SolutionOn heating water, bubbles being formed at the bottom of the vessel detach and rise. Take the bubbles to be spheres of radius R and making a circular contact of radius r with the bottom of the vessel. If r<<R and the surface tension of water is T, value of r just before bubbles detach is: (density of water is ρw)

View SolutionMercury has an angle of contact equal to 140∘ with soda lime galss. A narrow tube of radius 1.00mm made of this glass is dipped in a through containing mercury. By what amount does the mercury dip down in the tube relative to the mercury surface outside? Surface tension of mercury at the temperature of the experiment is 0.465Nm−1. Density of mercury = 13.6×103kgm−3.
View Solution
