A thin tube sealed at both ends is 90 cm long. It is kept horizontally, the middle 10cm containing mercury and the two equal ends containing air at standard atmospheric pressure. The tube is turned and kept in vertical position. By what amount will the mercury be displaced?
To solve the problem of how much the mercury will be displaced when the tube is turned from a horizontal to a vertical position, we can follow these steps:
1. Understand the Setup:
- The tube is 90 cm long, with 10 cm of mercury in the middle.
- The remaining 80 cm is filled with air at standard atmospheric pressure (76 cm of mercury).
2. Identify the Lengths:
- When the tube is horizontal, the mercury occupies the middle 10 cm, leaving 40 cm of air on each side (total length = 90 cm).
- In vertical position, the mercury will fall due to gravity, causing a displacement.
3. Define Variables:
- Let X be the amount of mercury displaced downwards.
- The height of air column in chamber A becomes 40+X cm.
- The height of air column in chamber B becomes 40−X cm.
4. Apply Boyle's Law:
- For chamber A (initially at 40 cm of air):
PA⋅VA=PA⋅(A⋅(40+X))
where PA is the pressure in chamber A.
- For chamber B (initially at 40 cm of air):
PB⋅VB=PB⋅(A⋅(40−X))
where PB is the pressure in chamber B.
5. Set Up the Equations:
- The initial pressure in both chambers is atmospheric pressure (76 cm of mercury):
PA=76⋅4040+X(for chamber A)
PB=76⋅4040−X(for chamber B)
6. Pressure Balance:
- The pressure in chamber B must balance the pressure from the mercury and chamber A:
PB=PHg+PA
- The pressure due to mercury is PHg=ρgh, where h=10 cm (the height of mercury).
7. Combine the Equations:
- Substitute the expressions for PA and PB into the balance equation:
76⋅4040−X=76⋅4040+X+76
8. Solve for X:
- Rearranging and simplifying leads to a quadratic equation:
680X+5776X2−121600=0
- Use the quadratic formula to solve for X:
X=−b±√b2−4ac2a
- Substituting a=5776,b=680,c=−121600 yields:
X≈4.09 cm
Final Answer:
The mercury will be displaced by approximately 4.09 cm when the tube is turned to a vertical position.
- The tube is 90 cm long, with 10 cm of mercury in the middle.
- The remaining 80 cm is filled with air at standard atmospheric pressure (76 cm of mercury).
- When the tube is horizontal, the mercury occupies the middle 10 cm, leaving 40 cm of air on each side (total length = 90 cm).
- In vertical position, the mercury will fall due to gravity, causing a displacement.
- Let X be the amount of mercury displaced downwards.
- The height of air column in chamber A becomes 40+X cm.
- The height of air column in chamber B becomes 40−X cm.
- For chamber A (initially at 40 cm of air):
PA⋅VA=PA⋅(A⋅(40+X))
where PA is the pressure in chamber A.
- For chamber B (initially at 40 cm of air):
PB⋅VB=PB⋅(A⋅(40−X))
where PB is the pressure in chamber B.
- The initial pressure in both chambers is atmospheric pressure (76 cm of mercury):
PA=76⋅4040+X(for chamber A)
PB=76⋅4040−X(for chamber B)
- The pressure in chamber B must balance the pressure from the mercury and chamber A:
PB=PHg+PA
- The pressure due to mercury is PHg=ρgh, where h=10 cm (the height of mercury).
- Substitute the expressions for PA and PB into the balance equation:
76⋅4040−X=76⋅4040+X+76
- Rearranging and simplifying leads to a quadratic equation:
680X+5776X2−121600=0
- Use the quadratic formula to solve for X:
X=−b±√b2−4ac2a
- Substituting a=5776,b=680,c=−121600 yields:
X≈4.09 cm
The mercury will be displaced by approximately 4.09 cm when the tube is turned to a vertical position.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 (SOLVED EXAMPLES)
11 Videos
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 1 (ELASTICITY)
2 Videos
- PROPERTIES OF MATTERBOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 2 (SOLVED EXAMPLES)
22 Videos
- OSCILLATIONS BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)
2 Videos
- SAMPLE QUESTION PAPER - 01 BOOK - ICSECHAPTER - SAMPLE QUESTION PAPER - 01 EXERCISE - SECTION - D
12 Videos
Similar Questions
A thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of 60∘ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at 30∘C).
View SolutionA glass tube sealed at both ends is 100cm long, It lies horizontally with the middle 10cm containing mercury. The two ends of the tube contain air at 27∘Cand at a pressure 76cm of mercury. The air column on one side is maintained at 0∘Cand the other side is maintained at 127∘C. Calculate the length of the air column on the cooler side. neglect the changes in the volume of mercury and of the glass.
View Solution
Knowledge Check
A thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of 60∘ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at 30∘C).
A75.4B45.8C67.5D89.3
In the given figure a glass tube lies horizontally with the middle 20cm containing mercury. The two ends of the tube contains air at 27∘C and at a pressure 76cm of mercuy. Now the air column on one side in maintained at 0∘C and the other side is maintained at 127∘C. Find the new length of the air column on the cooler side. Neglect the changes in the volume of mercury and of the glass.

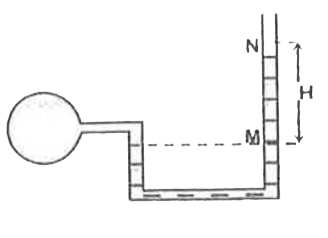
View SolutionA U - tube filled with mercury is connected to bulb containing a gas as shown . If atmospheric pressure is 1.013×105 Pa and H=0.05 m , then
View SolutionA metre long narrow bore held horizontally (and close at one end) contains a 76 cm long mercury thread, which traps a 15 cm column of air. What happens if the tube is held vertically with the open end at the bottom?
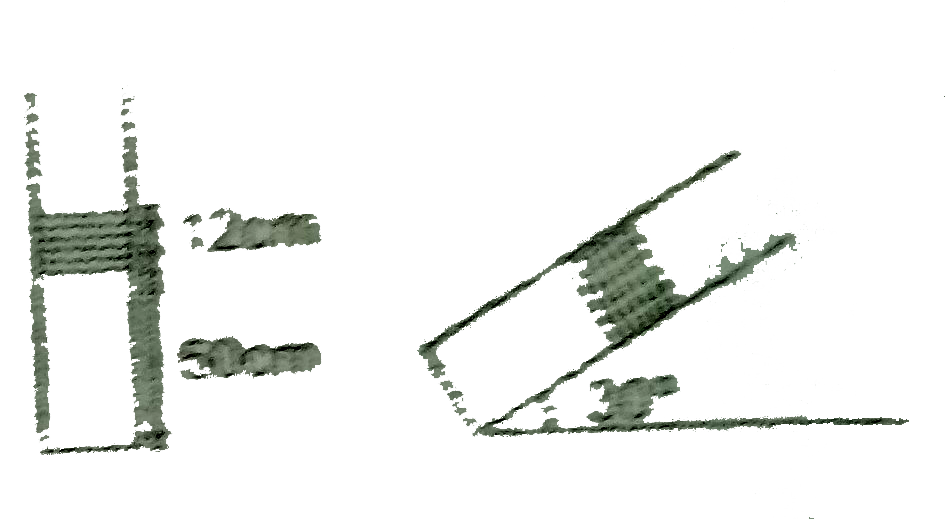
View SolutionIn given figure, an ideal gas is trapped between a mercury column and the closed end of a uniform vertical tube. The upper end of the tube is open to the atmosphere. Initially the lengths of the mercury column and the trapped air colume are 12cm and 50cm respectively. when the tube is titted solwly in a vertical plane through an angle of 30∘ with horizontal then the new length of air colume is x41m. Find x. Assuming the temperature to remains constant. (Patm=76cmofHg)
View SolutionA uniform tube closed at one end, contains a pallet of mercury 4 cm long. When the tube is kept vertically with closed end upwards, the length of air column between closed end and mercury pallet is 10 cm. the tube is inverted so that open end becomes upwards. find the length of trapped air column (in cm) trapped. take atmospheric pressure 76 cm of Hg.
View SolutionA narrow tube 50 cm length is closed at one end contains air trapped by a certain column of Hg. When the tubes is held horizontally, the air column in if has a length of 10cm. What is the pressure exerted by air when the tube is held vertically with the open end at the bottom and the length of the air column is 15cm? Also find the vertical height of the mercury column inside the tube.
View SolutionTorricelli was the first do devise an experiment for measuring atmospheric pressure . He took calibrated hard glass tube , 1 m in lengt and of uniform cross section , closed at one end . He filled the whole tube with dry mercury taking care than no air or water droplets remain inside the tube , closed the opposite end of the tube tightly with thumb and inverted it . He put this inverted mercury tube into a mercury through , taking care that the end of the tube remains inside the mercury through , An interesting thing was noticed . Mercury in the tube fell down at first and then stopped at a particular position . The height was 76 cm above the free surface of mercury in the through . When the given tube was inclined or lowered in the mercury trough , the vertical haight of mercury level in the tube was always found constant .
Torricelli explained this by saying that the free surface of mercury in the trough . Hence , the hydrostatic pressure exerted by the trough measures the atmospheric pressure .
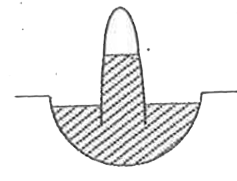
If this expriment uses water instead of mercury , then
A. length of water will be equal to 76 cm
B. length of water will be less than 76 cm
C. length of water will be greater than 76 cm
D. none of the above
View Solution
