Obtain the equation of a harmonic wave travelling in the negative x-direction with the characteristics given below. Amplitude is 4.5 cm, wavelength is 20 cm, wåve velocity is 40ms−1?
The correct Answer is:y=4.5sin2π
To obtain the equation of a harmonic wave traveling in the negative x-direction with the given characteristics, we will follow these steps:
Step 1: Identify the wave equation
The general equation for a wave traveling in the negative x-direction is given by:
y=Asin(ωt+kx)
where:
- y is the displacement,
- A is the amplitude,
- ω is the angular frequency,
- k is the angular wave number,
- t is the time,
- x is the position.
Step 2: Determine the amplitude
From the question, the amplitude A is given as:
A=4.5 cm
Step 3: Calculate the angular wave number k
The angular wave number k can be calculated using the formula:
k=2πλ
where λ is the wavelength. Given that the wavelength λ is 20 cm, we convert it to meters:
λ=20 cm=0.2 m
Now substituting the value of λ:
k=2π0.2=10π m−1
Step 4: Calculate the angular frequency ω
We know the relationship between wave velocity v, angular frequency ω, and angular wave number k:
v=ωk
Rearranging this gives:
ω=v⋅k
Given that the wave velocity v is 40 m/s, we can substitute the values:
ω=40⋅10π=400π rad/s
Step 5: Write the wave equation
Now we have all the necessary parameters:
- Amplitude A=4.5 cm
- Angular frequency ω=400π rad/s
- Angular wave number k=10π m−1
Substituting these values into the wave equation:
y=4.5sin(400πt+10πx)
Final Equation
Thus, the equation of the harmonic wave traveling in the negative x-direction is:
y=4.5sin(400πt+10πx)
---
The general equation for a wave traveling in the negative x-direction is given by:
y=Asin(ωt+kx)
where:
- y is the displacement,
- A is the amplitude,
- ω is the angular frequency,
- k is the angular wave number,
- t is the time,
- x is the position.
From the question, the amplitude A is given as:
A=4.5 cm
The angular wave number k can be calculated using the formula:
k=2πλ
where λ is the wavelength. Given that the wavelength λ is 20 cm, we convert it to meters:
λ=20 cm=0.2 m
Now substituting the value of λ:
k=2π0.2=10π m−1
We know the relationship between wave velocity v, angular frequency ω, and angular wave number k:
v=ωk
Rearranging this gives:
ω=v⋅k
Given that the wave velocity v is 40 m/s, we can substitute the values:
ω=40⋅10π=400π rad/s
Now we have all the necessary parameters:
- Amplitude A=4.5 cm
- Angular frequency ω=400π rad/s
- Angular wave number k=10π m−1
y=4.5sin(400πt+10πx)
Thus, the equation of the harmonic wave traveling in the negative x-direction is:
y=4.5sin(400πt+10πx)
Topper's Solved these Questions
- BOOK - ICSECHAPTER - WAVESEXERCISE - From Sound Wave Velocity37videos
WAVES
- BOOK - ICSECHAPTER - WAVESEXERCISE - From Organ Pipes22videos
WAVES
- BOOK - ICSECHAPTER - WAVESEXERCISE - Very Short Answer Questions14videos
WAVES
- BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - UNSOLVED PROBLEMS79videos
VECTORS SCALARS ELEMENTARY CALCULUS
Similar Questions
Obtain the equation of a harmonic wave travelling is the +ve x-direction and has the characteristics given below. Amplitudes is 4cm, speed is 2m/s and frequency is 8Hz.
View Solution(b) Write the equation of a progressive wave propagating along the positive x-direction, whose amplitude is 5 cm, frequency 250 Hz and velocity 500ms−1.
View Solution
Knowledge Check
- Question 1 - Select One or More
A wave is represented by the equation
y=Asin(10πx+15πt+π/3)
Where x is in metre and t is in second.
a wave travelling in the positive x-direction with a velocity of 1.5 m/s
a wave travelling in the negative x-direction with a velocity 1.5 m/s
a wave travelling in the negative x-direction with a wavelength of 0.2 m
a wave travelling in the positive x-direction with a wavelength 0.2 mAa wave travelling in the positive x-direction with a velocity of 1.5 m/sBa wave travelling in the negative x-direction with a velocity 1.5 m/sCa wave travelling in the negative x-direction with a wavelength of 0.2 mDa wave travelling in the positive x-direction with a wavelength 0.2 m
Write down the equation of progressive wave travelling along the negative X-axis and having an amplitude of 0.21m frequency of 225 Hz nd a wavelength of 0.6 m.
View SolutionA sinusoidal wave trsvelling in the positive direction on a stretched string has amplitude 2.0cm, wavelength 1.0m and velocity 5.0m/s. At x=0 and t=0 it is given that y=0 and ∂y∂t<0. Find the wave function y(x,t).
View SolutionFigure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is 20cms−1. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .View Solution
.View SolutionDetermine the amplitudes of the resultant motion when two sinusoidal waves of same frequency , travelling in the same direction are combined . Their amplitudes are 3.0cmand4.0cm and they differ in phase by π/2radians.
View SolutionTwo sinusoidal wave having the same frequency and travelling in the same direction are combined. What is the amplitude of the resultant motion if their amplitudes are 3cm and 4cm and they differ in phase by π/2 radian?
View SolutionA wave is travelling towards positive x-direction. If the pulse of the wave at any instant is given as shown in figure then which particle will have negative velocity at any instant?
View SolutionFigure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
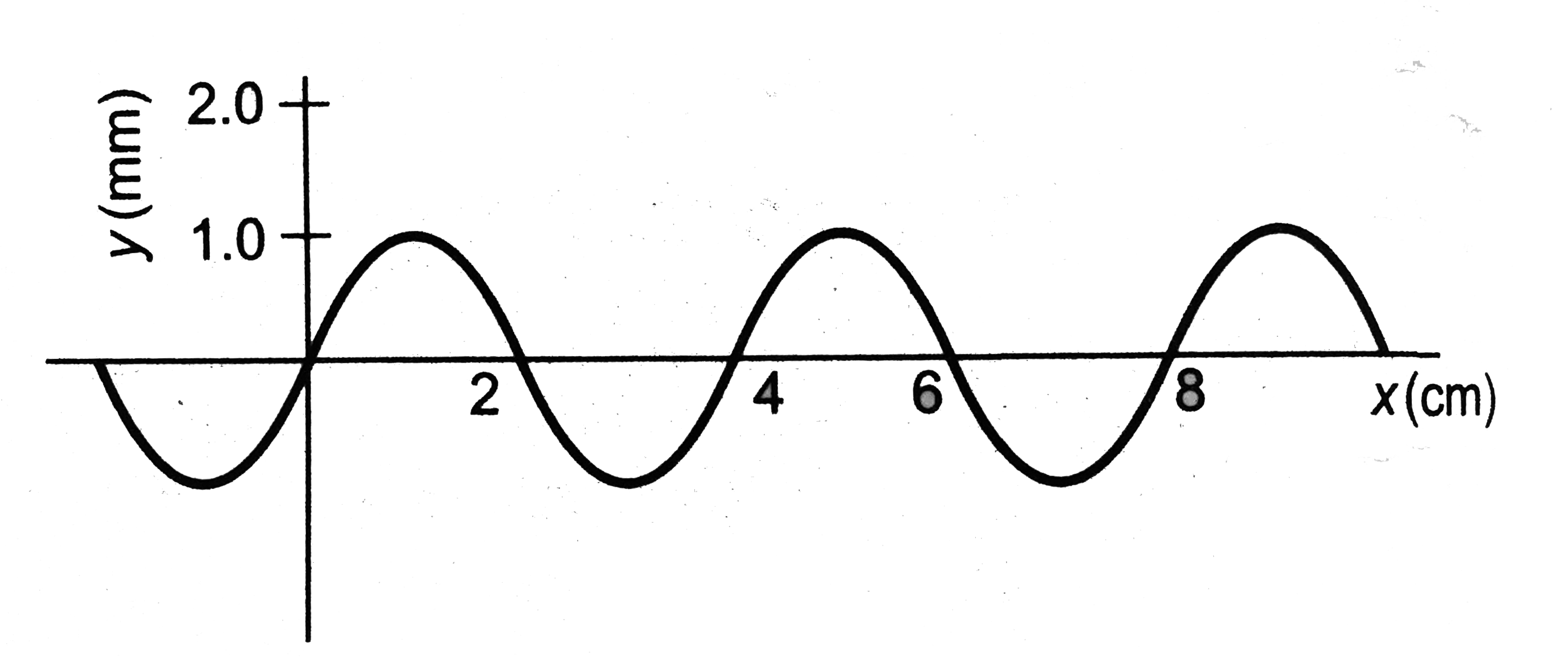 View Solution
View Solution

