Let ABC be a triangle inscribed in a circle and let la=maMa,lb=mbMb,lc=mcMc where ma,mb,mc are the lengths of the angle bisectors of angles A, B and C respectively , internal to the triangle and Ma,MbandMc are the lengths of these internal angle bisectors extended until they meet the circumcircle.
Q. The minimum value of the expression lasin2A+lbsin2B+lcsin2C is :
2
3
4
none of these
The correct Answer is:B
To find the minimum value of the expression
lasin2A+lbsin2B+lcsin2C
where la=maMa, lb=mbMb, and lc=mcMc, we will follow these steps:
Step 1: Understand the Definitions
We know that:
- ma, mb, mc are the lengths of the internal angle bisectors of angles A, B, and C respectively.
- Ma, Mb, Mc are the lengths of these angle bisectors extended until they meet the circumcircle.
Step 2: Express la, lb, and lc
Using the known formulas for the lengths of the angle bisectors, we have:
ma=2bcb+ccos(A2)
Ma=2bcb+c⋅RR−a
where R is the circumradius of triangle ABC.
Thus, we can express la as:
la=maMa=cos(A2)RR−a=(R−a)cos(A2)R
Step 3: Substitute into the Expression
Now substituting la, lb, and lc into the expression:
lasin2A+lbsin2B+lcsin2C
This becomes:
(R−a)cos(A2)Rsin2A+(R−b)cos(B2)Rsin2B+(R−c)cos(C2)Rsin2C
Step 4: Apply Cauchy-Schwarz Inequality
Using the Cauchy-Schwarz inequality, we can derive that:
(lasin2A+lbsin2B+lcsin2C)(sin2A+sin2B+sin2C)≥(la+lb+lc)2
Step 5: Find the Minimum Value
By applying the AM-GM inequality to la,lb,lc:
la+lb+lc3≥√(3)lalblc
This leads us to conclude that the minimum value of the expression can be shown to be 3.
Conclusion
Thus, the minimum value of the expression
lasin2A+lbsin2B+lcsin2C
is
3
We know that:
- ma, mb, mc are the lengths of the internal angle bisectors of angles A, B, and C respectively.
- Ma, Mb, Mc are the lengths of these angle bisectors extended until they meet the circumcircle.
Using the known formulas for the lengths of the angle bisectors, we have:
ma=2bcb+ccos(A2)
Ma=2bcb+c⋅RR−a
where R is the circumradius of triangle ABC.
la=maMa=cos(A2)RR−a=(R−a)cos(A2)R
Now substituting la, lb, and lc into the expression:
lasin2A+lbsin2B+lcsin2C
This becomes:
(R−a)cos(A2)Rsin2A+(R−b)cos(B2)Rsin2B+(R−c)cos(C2)Rsin2C
Using the Cauchy-Schwarz inequality, we can derive that:
(lasin2A+lbsin2B+lcsin2C)(sin2A+sin2B+sin2C)≥(la+lb+lc)2
By applying the AM-GM inequality to la,lb,lc:
la+lb+lc3≥√(3)lalblc
This leads us to conclude that the minimum value of the expression can be shown to be 3.
Thus, the minimum value of the expression
Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-4 : Matching Type Problems
2 Videos
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-5 : Subjective Type Problems
11 Videos
- SOLUTION OF TRIANGLESBOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-2 : One or More than One Answer is/are Correct
15 Videos
- SEQUENCE AND SERIES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
21 Videos
- STRAIGHT LINES BOOK - VIKAS GUPTA (BLACK BOOK) ENGLISHCHAPTER - STRAIGHT LINES EXERCISE - Exercise-5 : Subjective Type Problems
10 Videos
Similar Questions
Let ABC be a triangle inscribed in a circle and let la=maMa,lb=mbMb,lc=mcMc where ma,mb,mc are the lengths of the angle bisectors of angles A, B and C respectively , internal to the triangle and Ma,MbandMc are the lengths of these internal angle bisectors extended until they meet the circumcircle.
Q. la equals :
View SolutionLet ABC be a triangle inscribed in a circle and let la=maMa,lb=mbMb,lc=mcMc where ma,mb,mc are the lengths of the angle bisectors of angles A, B and C respectively , internal to the triangle and Ma,MbandMc are the lengths of these internal angle bisectors extended until they meet the circumcircle.
Q. The maximum value of the product (lalblc)×cos2(B−C2)×cos2(C−A)2)×cos2(A−B2) is equal to :
View SolutionIn Figure, ABC is an equilateral triangle. Find m ∠BEC
View SolutionIf l, m, n denote the side of a pedal triangle, then la2+mb2+nc2 is equal to
View SolutionLet ABC be a triangle with ∠B=900 . Let AD be the bisector of ∠A with D on BC. Suppose AC=6cm and the area of the triangle ADC is 10cm2. Find the length of BD.
View SolutionLet ABC be a triangle with ∠B=900 . Let AD be the bisector of ∠A with D on BC. Suppose AC=6cm and the area of the triangle ADC is 10cm2. Find the length of BD.
View SolutionABCD is a parallelogram. L and M are points on AB and DC respectively and AL=CM. prove that LM and BD bisect each other.
View SolutionIn ABC,∠A=500,∠B=700 and bisector of ∠C meets AB at D. find the angles of the triangle ADC and BDC.
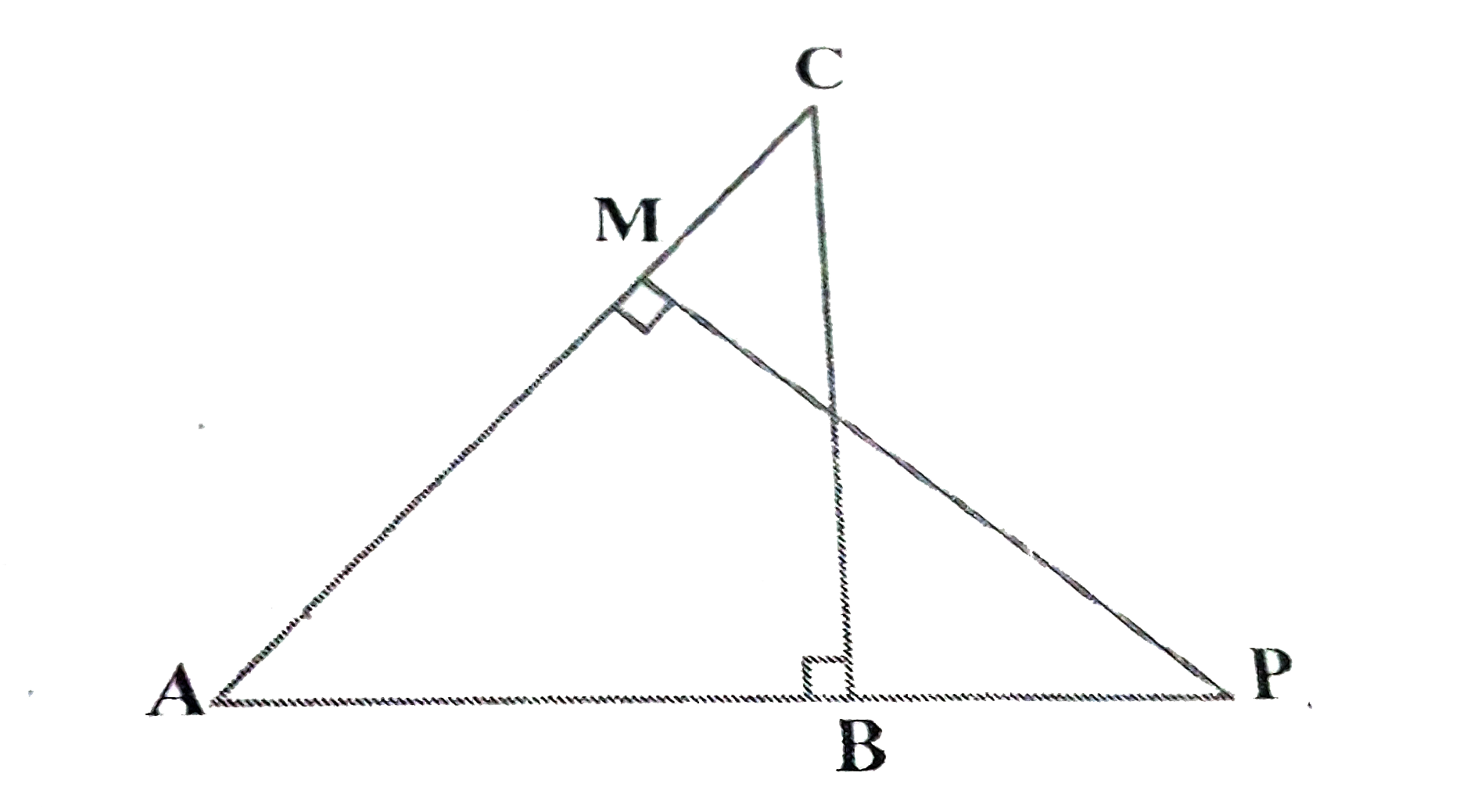
View SolutionIn figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that(i) ΔABC~ΔAMP (ii) CAPA=BCMP
View SolutionIn a right angled triangle ABC, right angled at B, BC=12cm and AB=5cm. The radius of the circle inscribed in the triangle (in cm) is :
View Solution
