In figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that(i) ΔABC~ΔAMP (ii) CAPA=BCMP
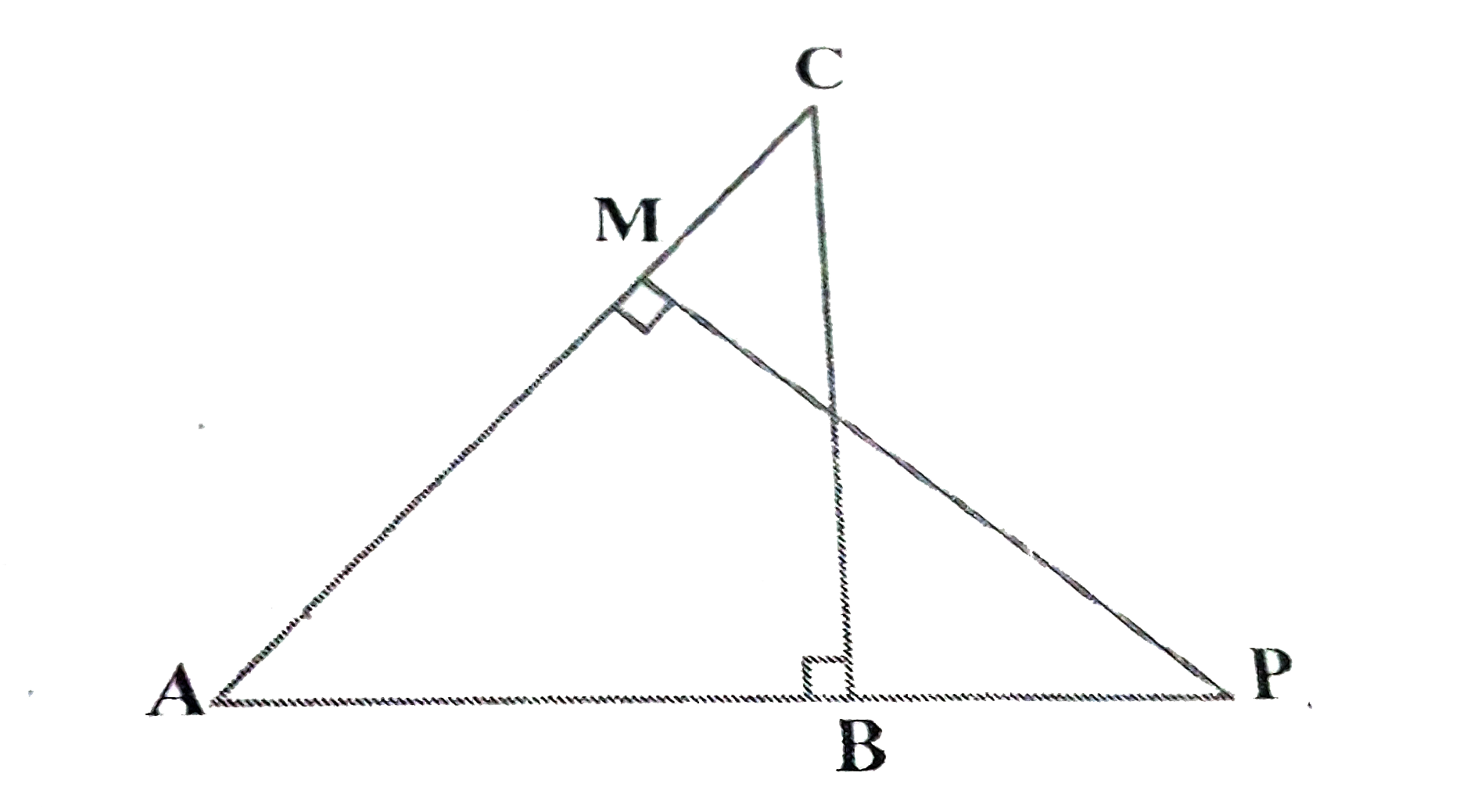
(i)In ΔABC and ΔAMP
∠BAC=∠PAM, As both angles are common.
∠ABC=∠AMP=90∘
As these two angles are equal, third angle will also be equal for these two triangles.
As, all three angles are equal, we can say that
ΔABC~ΔAMP
(ii)It also means,
ABAM=CAPA=BCMP
Hence,CAPA=BCMP
Similar Questions
If ABC and AMP are two right triangles, right angled at B and M respectively such that ∠MAP=∠BAC . Prove that CAPA=BCMP
View SolutionIf AD and PM are medians of triangles ABC and PQR, respectively whereΔABC~ΔPQR, prove that ABPQ=ADPM
View SolutionABC is an isosceles right triangle right-angled at C . Prove that AB2=2AC2 .
View SolutionBL and CM are medians of a triangle ABC right angled at A. Prove that 4(BL2+CM2)=5BC2
View SolutionABC is an isosceles right triangle, right-angled at C . Prove that: AB2=2AC2.
View SolutionIn figure Cm and RN are respectively the medians of ΔABCand ΔPQR. If ΔABC~ΔPQR, prove that: (i) ΔAMC~ΔPNR (ii) CMRN=ABPQ (ii) ΔCMB~ΔRNQ
View SolutionIn Figure, two chords AB and CD intersect each other at the point P. Prove that:
(i) ΔAPC~ΔDPB
(ii) AP⋅PB=CP⋅DP

View SolutionConstruct a right triangle, right angled at C in which AB=5.2\ cm and BC=4.6 cm.
View SolutionIn figure, if ΔABE≅ΔACD, show that ΔADE~ΔABC.

View SolutionIn Figure, ABC is a right triangle right angled at B and D is the foot of the the perpendicular drawn from B on AC . If DM⊥BC and DN⊥AB , prove that: (i)DM2=DN×MC (ii)DN2=DM×AN
View Solution