If high voltage current is applied on the field given by the graph y+|y|−x−|x|=0. on which of the following curve a person can move so that the remains safe ?
y=x2
y=sgn(−e2)
y=log1/3x
y=m+|x|,m>3
The correct Answer is:D
To solve the problem given by the equation y+|y|−x−|x|=0, we will analyze it in different quadrants based on the signs of x and y.
1. Understanding the Equation:
The equation can be rewritten as:
y+|y|=x+|x|
This means we need to consider different cases based on the signs of x and y.
2. Case 1: x≥0 and y≥0:
Here, |y|=y and |x|=x:
y+y=x+x⟹2y=2x⟹y=x
This represents the line y=x in the first quadrant.
3. Case 2: x<0 and y<0:
Here, |y|=−y and |x|=−x:
y−y=−x−(−x)⟹0=0
This case gives no new information.
4. Case 3: x≥0 and y<0:
Here, |y|=−y and |x|=x:
y−y=x−x⟹0=0
Again, this case gives no new information.
5. Case 4: x<0 and y≥0:
Here, |y|=y and |x|=−x:
y+y=−x+x⟹2y=0⟹y=0
This represents the line y=0 in the second quadrant.
6. Summary of Results:
- In the first quadrant: y=x
- In the second quadrant: y=0
- In the third quadrant: No new information.
- In the fourth quadrant: No new information.
7. Graphing the Results:
- The line y=x intersects the x-axis and y-axis at the origin.
- The line y=0 is the x-axis.
8. Identifying Safe Regions:
The regions where a person can move safely are outside the lines y=x and y=0.
9. Analyzing Options:
- Option 1: y=x2 - This parabola intersects y=x and is unsafe.
- Option 2: y=−1 - This line is below y=0 and is unsafe.
- Option 3: y=m+|x| where m>3 - This line is above y=0 and does not intersect y=x or y=0, making it safe.
Conclusion:
The correct answer is y=m+|x| where m>3.
The equation can be rewritten as:
y+|y|=x+|x|
This means we need to consider different cases based on the signs of x and y.
Here, |y|=y and |x|=x:
y+y=x+x⟹2y=2x⟹y=x
This represents the line y=x in the first quadrant.
Here, |y|=−y and |x|=−x:
y−y=−x−(−x)⟹0=0
This case gives no new information.
Here, |y|=−y and |x|=x:
y−y=x−x⟹0=0
Again, this case gives no new information.
Here, |y|=y and |x|=−x:
y+y=−x+x⟹2y=0⟹y=0
This represents the line y=0 in the second quadrant.
- In the first quadrant: y=x
- In the second quadrant: y=0
- In the third quadrant: No new information.
- In the fourth quadrant: No new information.
- The line y=x intersects the x-axis and y-axis at the origin.
- The line y=0 is the x-axis.
The regions where a person can move safely are outside the lines y=x and y=0.
- Option 1: y=x2 - This parabola intersects y=x and is unsafe.
- Option 2: y=−1 - This line is below y=0 and is unsafe.
- Option 3: y=m+|x| where m>3 - This line is above y=0 and does not intersect y=x or y=0, making it safe.
The correct answer is y=m+|x| where m>3.
Topper's Solved these Questions
- FUNCTION BOOK - VK JAISWAL ENGLISHCHAPTER - FUNCTION EXERCISE - ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT
23 Videos
- FUNCTION BOOK - VK JAISWAL ENGLISHCHAPTER - FUNCTION EXERCISE - COMPREHENSION TYPE PROBLEMS
15 Videos
- ELLIPSE BOOK - VK JAISWAL ENGLISHCHAPTER - ELLIPSE EXERCISE - Exercise-4 : Subjective Type Problems
2 Videos
- HYPERBOLABOOK - VK JAISWAL ENGLISHCHAPTER - HYPERBOLAEXERCISE - Exercise-4 : Subjective Type Problems
3 Videos
Similar Questions
Knowledge Check
Which of the following could be the graph of 2x+3y+12=0 ?
A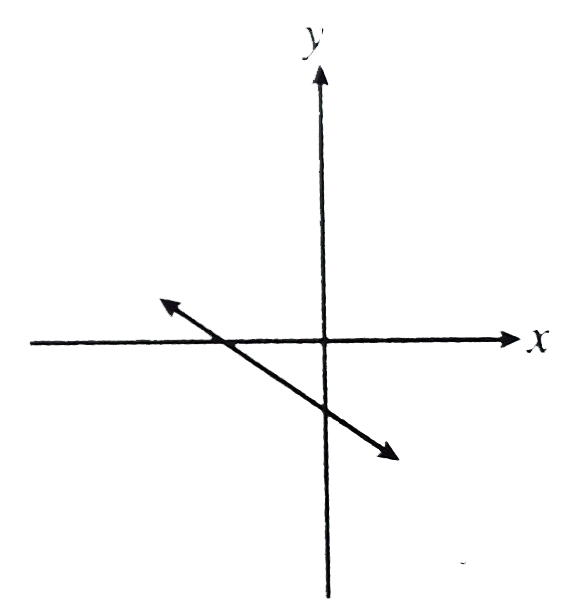 B
B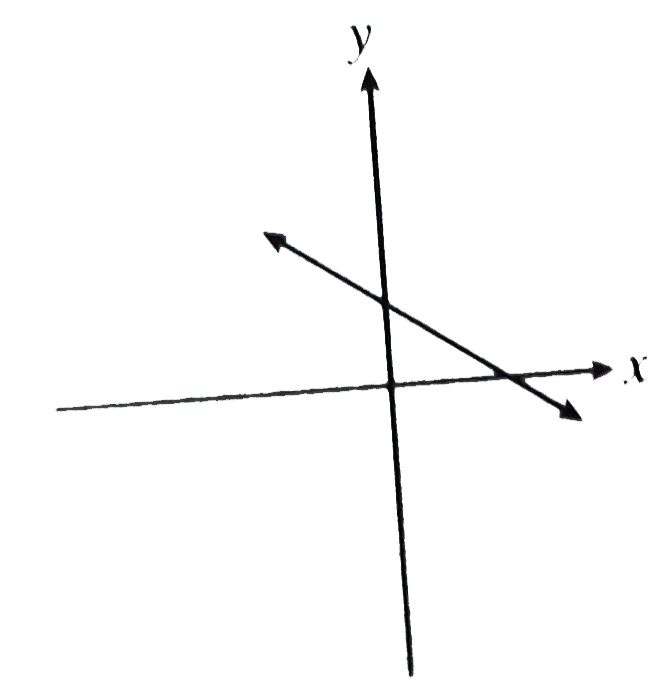 C
C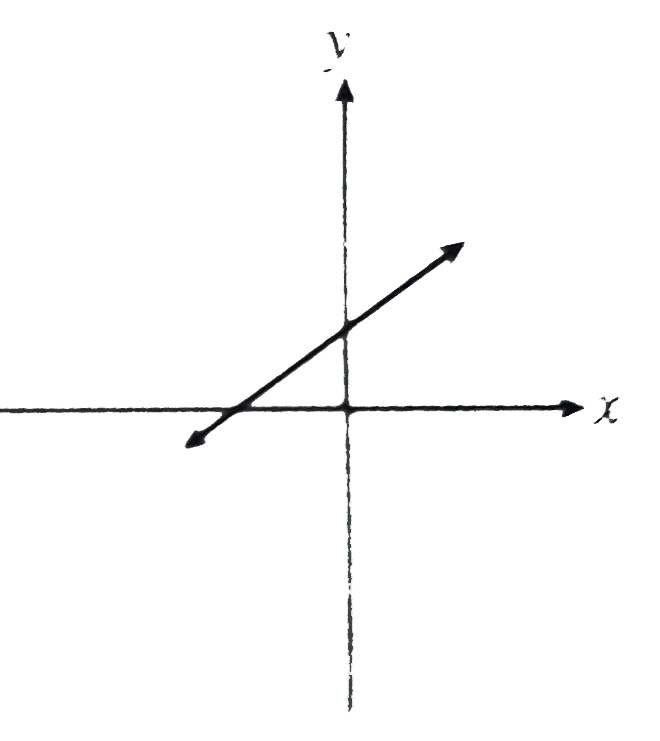 D
D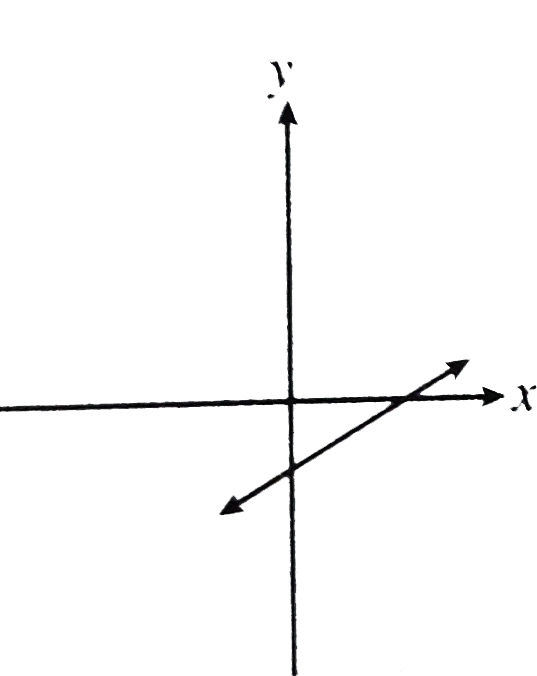
Which of the following could be the graph of 2x+3y+12=0 ?
A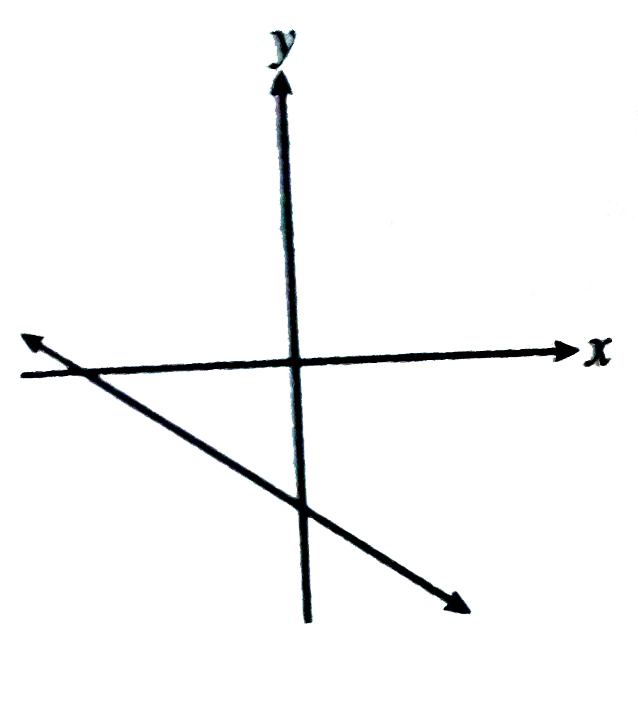 B
B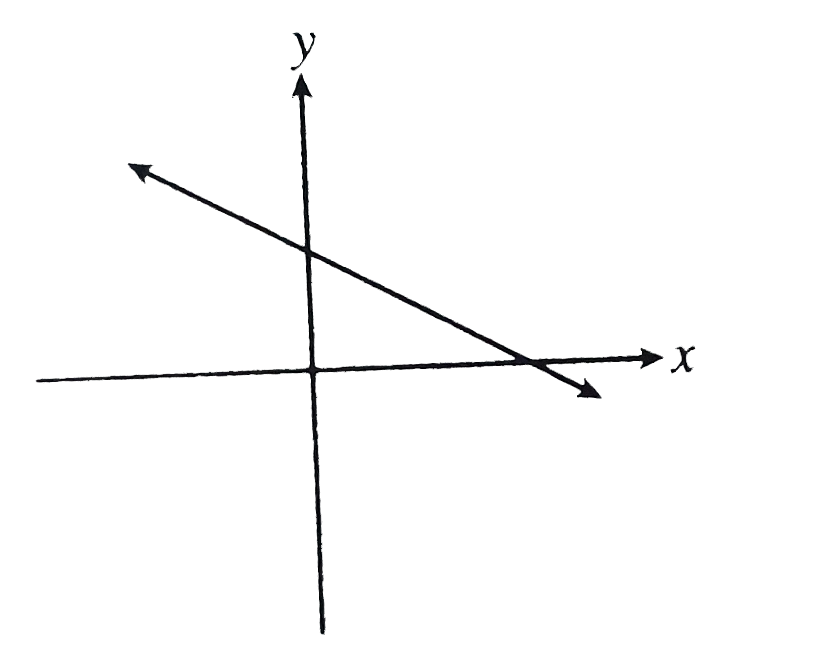 C
C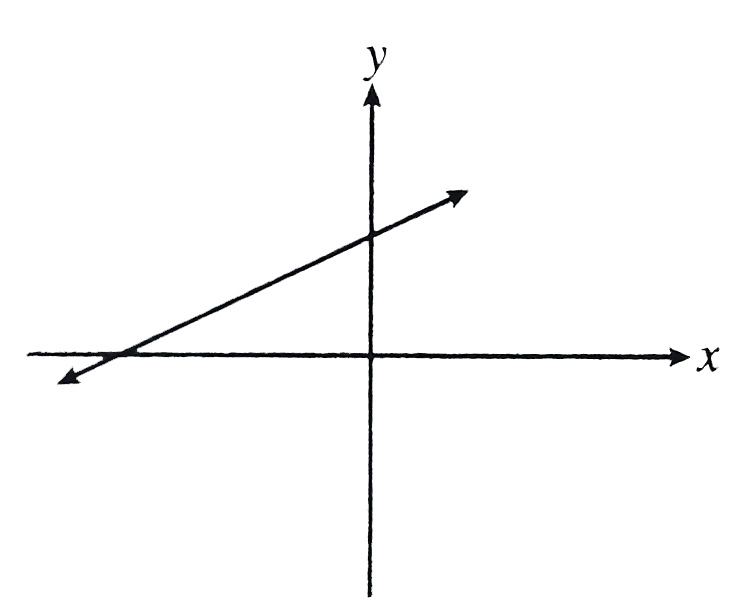 D
D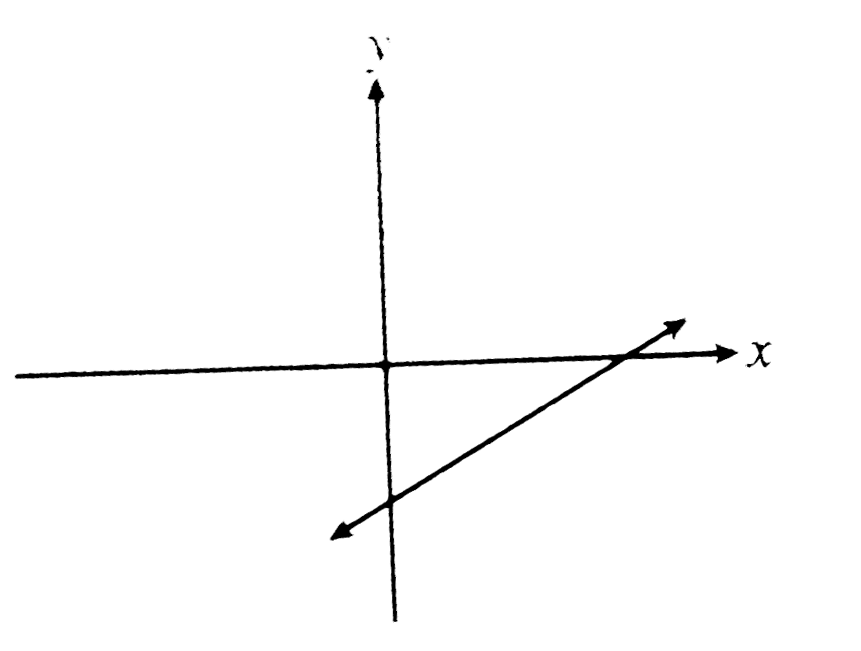
If (x,y) represents a point on the the graph of y=2x+1, which of the following could be a portion of the graph of the set of points (x,y2)?
A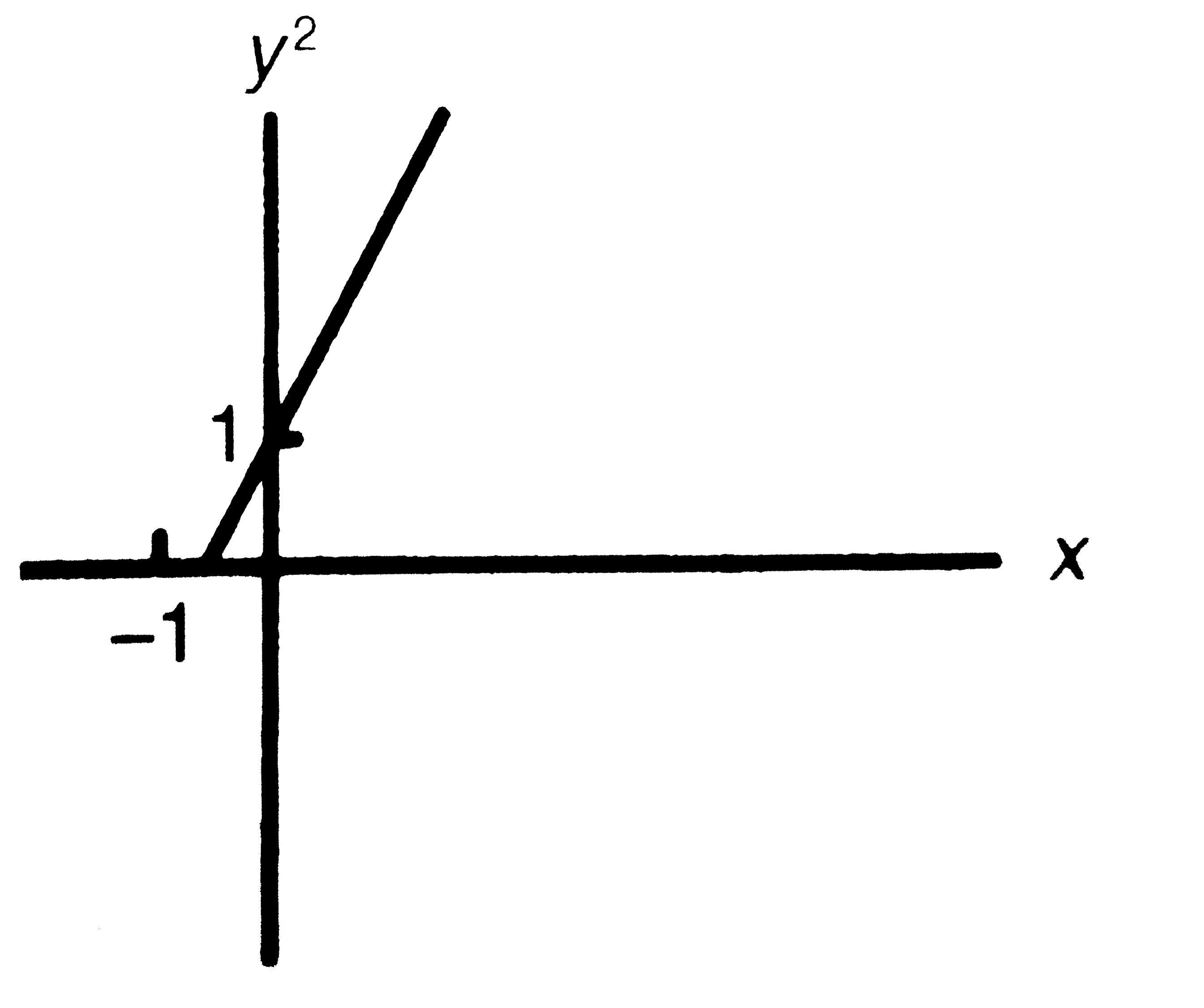 B
B C
C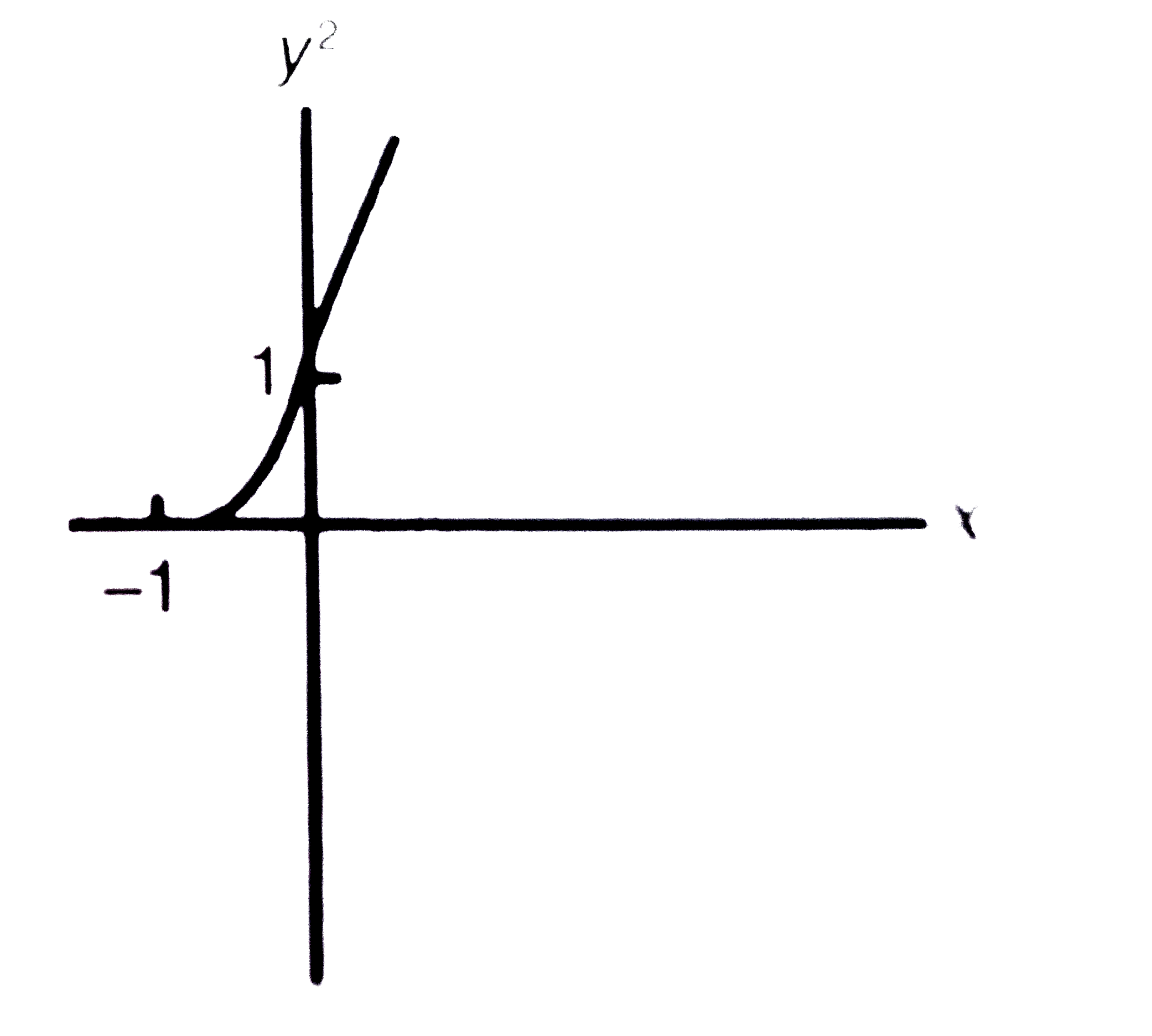 D
D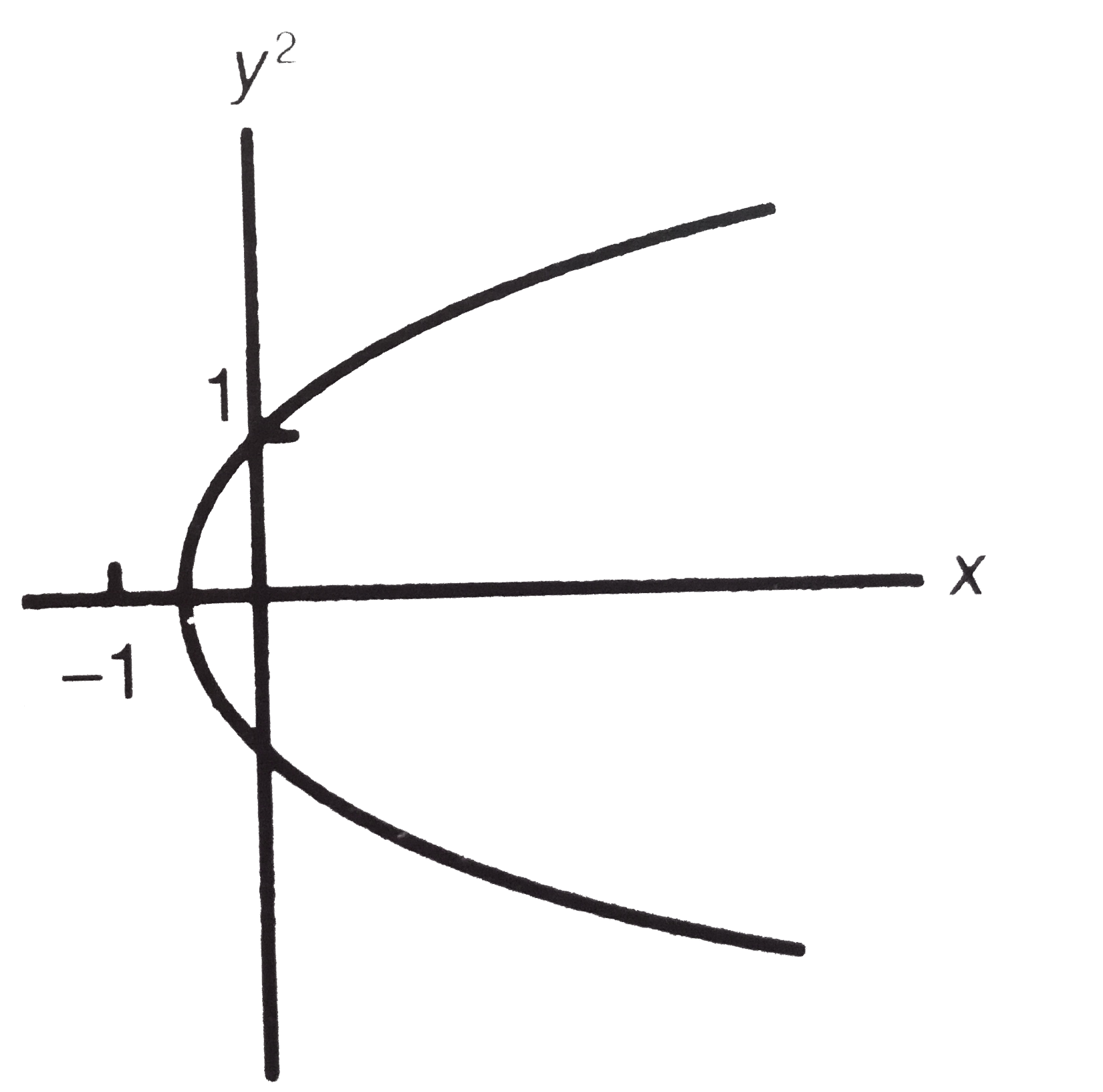
Which of the following can represent the curve x2=−2y ?
View SolutionGiven the graph of y=f(x)
View SolutionWhich of the following is the graph of the curve √|y|=x is ?
View Solution
The graph above shows y=2x. Which of the following graphs represents y=|2x|?
View SolutionThe graph of y=f(x) is shown below.
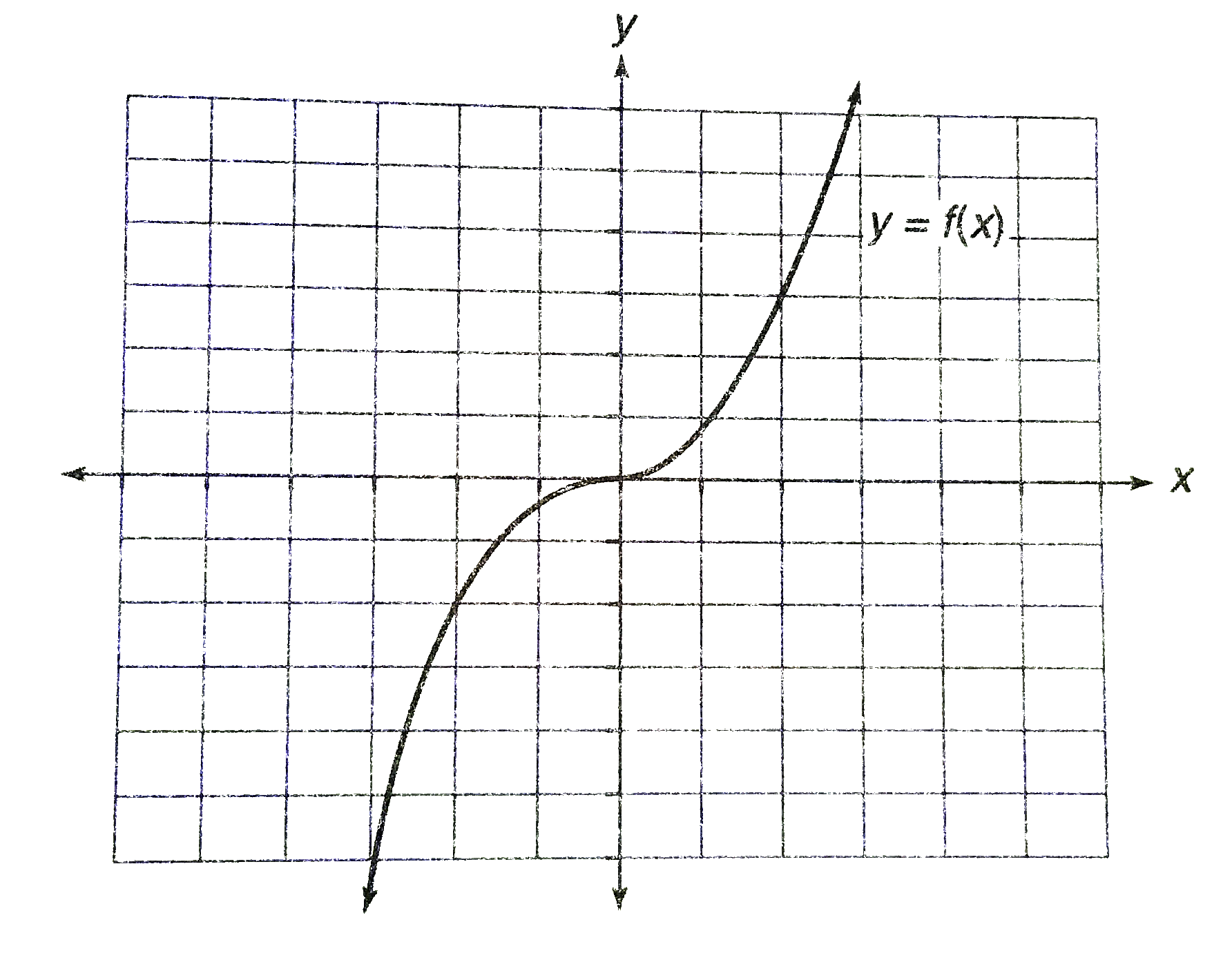
Which of the following could represents the graph of y=f(x−2)+1?
View Solution
