In a triangle ABC with altitude AD, ∠BAC=45∘,DB=3andCD=2. The area of the triangle ABC is : (a) 6 (b) 15 (c) 154 (d) 12
6
15
15/4
12
The correct Answer is:B
To find the area of triangle ABC with the given conditions, we can follow these steps:
Step 1: Understand the Triangle
We have triangle ABC with altitude AD. Given:
- Angle BAC = 45°
- DB = 3
- CD = 2
Step 2: Set Up the Diagram
Draw triangle ABC with point D on side BC such that:
- BD = 3
- CD = 2
- Therefore, BC = BD + CD = 3 + 2 = 5.
Step 3: Define the Altitude
Let the length of altitude AD be denoted as h.
Step 4: Use the Tangent Function
Since angle BAC = 45°, we can express the tangent of the angles:
- Let angle BAD = α
- Let angle CAD = β
- We know that α+β=45°.
Using the tangent addition formula:
tan(α+β)=tanα+tanβ1−tanαtanβ=1
Step 5: Express Tangents in Terms of h
From triangle ABD:
tanα=DBAD=3h
From triangle ACD:
tanβ=CDAD=2h
Step 6: Substitute into the Tangent Formula
Substituting the values into the tangent addition formula:
3h+2h1−3h⋅2h=1
This simplifies to:
5h1−6h2=1
Step 7: Cross Multiply
Cross multiplying gives:
5=h−6h
Multiplying through by h to eliminate the fraction:
5h=h2−6
Step 8: Rearranging the Equation
Rearranging gives us a quadratic equation:
h2−5h−6=0
Step 9: Factor the Quadratic
Factoring the quadratic:
(h−6)(h+1)=0
This gives us two solutions:
h=6orh=−1
Since h represents a length, we take h=6.
Step 10: Calculate the Area
The area A of triangle ABC can be calculated using the formula:
A=12×base×height
Here, the base BC=5 and height AD=h=6:
A=12×5×6=302=15
Final Answer
The area of triangle ABC is 15.
We have triangle ABC with altitude AD. Given:
- Angle BAC = 45°
- DB = 3
- CD = 2
Draw triangle ABC with point D on side BC such that:
- BD = 3
- CD = 2
- Therefore, BC = BD + CD = 3 + 2 = 5.
Let the length of altitude AD be denoted as h.
Since angle BAC = 45°, we can express the tangent of the angles:
- Let angle BAD = α
- Let angle CAD = β
- We know that α+β=45°.
tan(α+β)=tanα+tanβ1−tanαtanβ=1
From triangle ABD:
tanα=DBAD=3h
From triangle ACD:
tanβ=CDAD=2h
Substituting the values into the tangent addition formula:
3h+2h1−3h⋅2h=1
This simplifies to:
5h1−6h2=1
Cross multiplying gives:
5=h−6h
Multiplying through by h to eliminate the fraction:
5h=h2−6
Rearranging gives us a quadratic equation:
h2−5h−6=0
Factoring the quadratic:
(h−6)(h+1)=0
This gives us two solutions:
h=6orh=−1
Since h represents a length, we take h=6.
The area A of triangle ABC can be calculated using the formula:
A=12×base×height
Here, the base BC=5 and height AD=h=6:
A=12×5×6=302=15
The area of triangle ABC is 15.
Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - VK JAISWAL ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-2 : One or More than One Answer is/are Correct
14 Videos
- SOLUTION OF TRIANGLESBOOK - VK JAISWAL ENGLISHCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-3 : Comprehension Type Problems
12 Videos
- SEQUENCE AND SERIES BOOK - VK JAISWAL ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
21 Videos
- STRAIGHT LINES BOOK - VK JAISWAL ENGLISHCHAPTER - STRAIGHT LINES EXERCISE - Exercise-5 : Subjective Type Problems
10 Videos
Similar Questions
Knowledge Check
In triangle ABC, ∠BAC=90∘ and AD⊥BC Such that ∠OBA=∠DAC Then:
ABD.CD=BC2BAB.AC=BC2CBD.CD=AD2DAB.AC=AD2
In triangle ABC medians AD and CE are drawn, if AD=5, ∠DAC=π8 and ∠ACE=π4, then the area of triangle ABC is equal to a. 258 b. 253 c. 2518 d. 103
View SolutionThe internal bisectors of the angles of a triangle ABC meet the sides in D, E, and F. Show that the area of the triangle DEF is equal to 2△abc(b+c)(c+a)(a+b), where △ is the area of ABC.
View SolutionIn a triangle ABC, AB = AC. Show that the altitude AD is median also.
View SolutionIf in a ΔABC,∠A=45∘,∠B=75∘, then
View SolutionIf the area of triangle ABC is 15, what is the length of AC ?
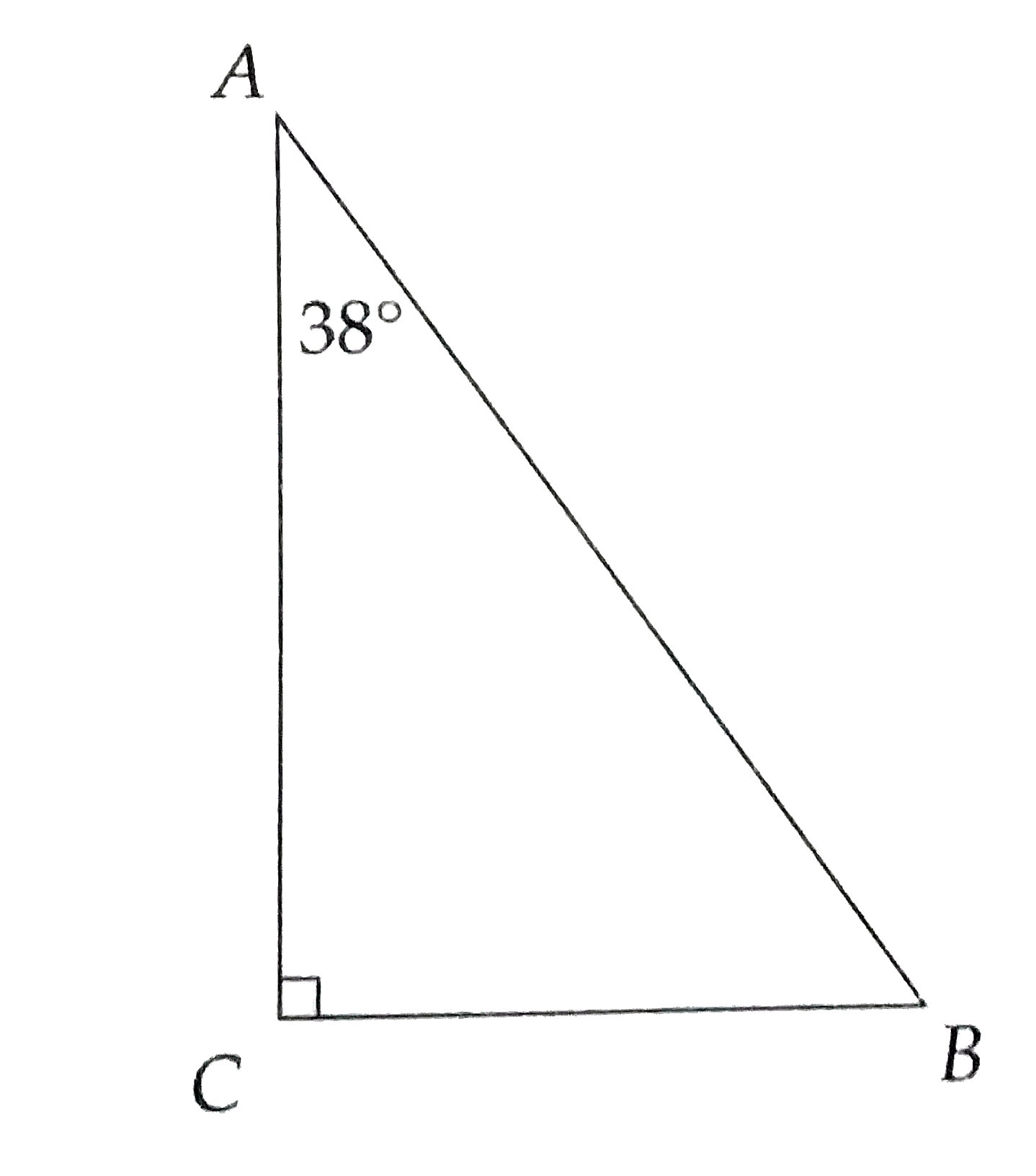
View SolutionIn a triangle ABC, medians AD and BE are deawn. IF AD=4,∠DAB=π6and∠ABE=π3, then the area of the trianlge ABC is-
View SolutionP1,P2,P3 are altitudes of a triangle ABC from the vertices A, B, C and Δ is the area of the triangle,
The value of P−11+P−12+P−13 is equal to-
View Solution
