Question

Plot a graph for the equation $y=-x^2+4x-1$.

Explanation

`a=-1, b=4, c=-1`. As a is negative, the parabola should open down.

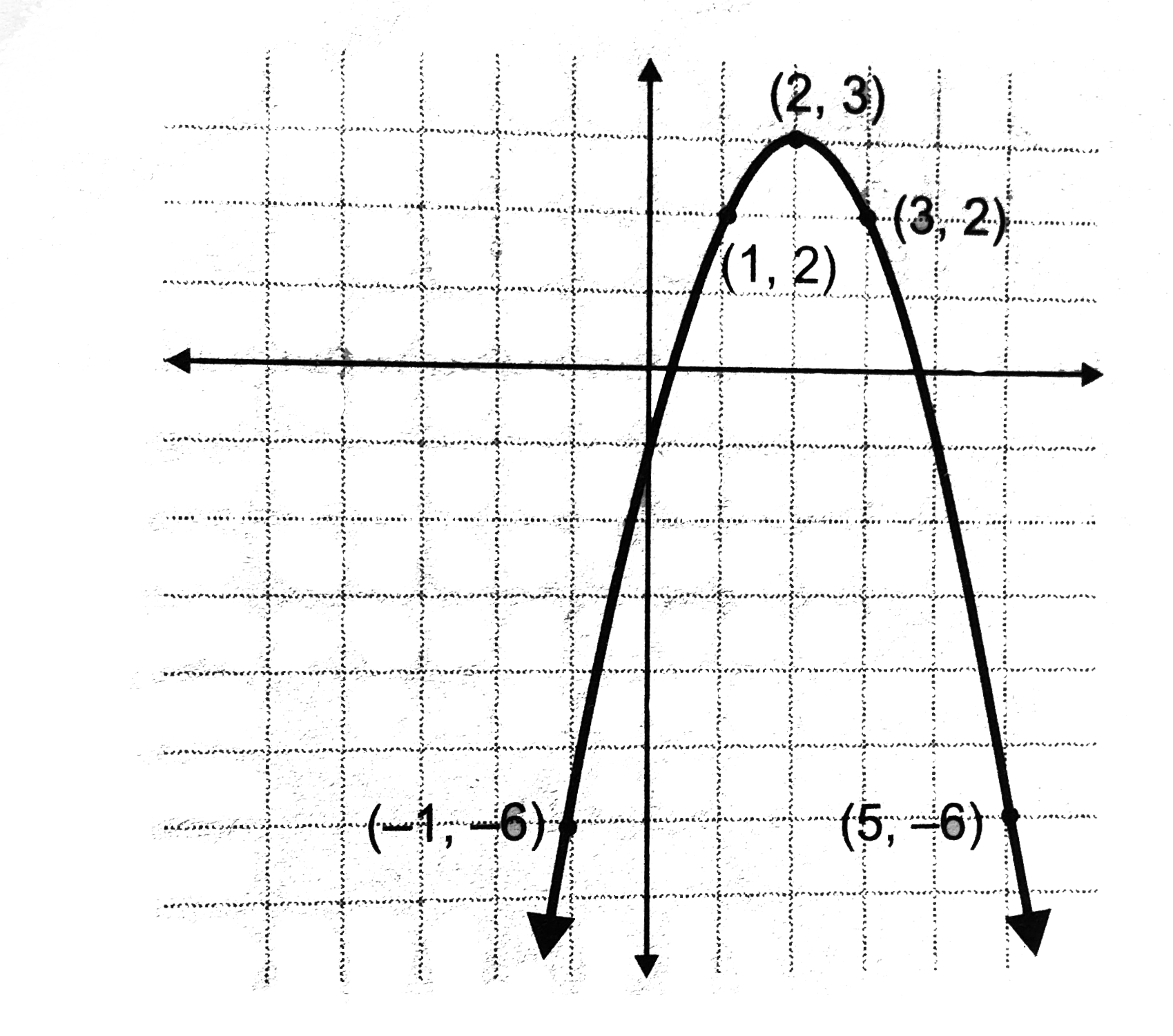
Vertex: `x=-b//2a=2`. Putting this value of x, we get `y=3`. Hence, the vertex of the parabola is `(2,3)`.
Assume two values of x as follows and find the corresponding values of y.
`|{:(x,1,-1),(y,2,-6):}|`
Points obtained are `(1,2)` and `(-1,-6)`.
Points obtained are `(2,3)(vertex)`,`[1,2]`, and `[-1,-6]`.
Symmetry of parabola: Mirror image points of `(1,2)` and
Now, sketch the parabola as shown in figure.