Two forces, while acting on particle in opposite directions,have the resultant of 10N. If they act at right angles to each other, the resultant is found to be 50N. Find the two forces?
To solve the problem, we need to find two forces F1 and F2 based on the conditions given. Let's break it down step by step.
Step 1: Understand the Problem
We have two forces acting on a particle:
1. When they act in opposite directions, the resultant force is 10N.
2. When they act at right angles to each other, the resultant force is 50N.
Step 2: Set Up the Equations
From the first condition (forces acting in opposite directions), we can write:
F1−F2=10(1)
Assuming F1>F2.
From the second condition (forces acting at right angles), we can use the Pythagorean theorem:
√F21+F22=50(2)
Squaring both sides gives:
F21+F22=2500(3)
Step 3: Substitute Equation (1) into Equation (3)
From equation (1), we can express F1 in terms of F2:
F1=F2+10
Now substitute this expression for F1 into equation (3):
(F2+10)2+F22=2500
Step 4: Expand and Simplify
Expanding (F2+10)2:
F22+20F2+100+F22=2500
Combining like terms:
2F22+20F2+100=2500
Subtracting 2500 from both sides:
2F22+20F2−2400=0
Step 5: Simplify the Quadratic Equation
Dividing the entire equation by 2:
F22+10F2−1200=0
Step 6: Solve the Quadratic Equation
To solve for F2, we can use the quadratic formula:
F2=−b±√b2−4ac2a
Where a=1, b=10, and c=−1200:
F2=−10±√102−4⋅1⋅(−1200)2⋅1
Calculating the discriminant:
F2=−10±√100+48002
F2=−10±√49002
F2=−10±702
Step 7: Calculate Possible Values for F2
Calculating the two possible values:
1. F2=602=30
2. F2=−802=−40 (not physically meaningful since force cannot be negative)
Thus, F2=30N.
Step 8: Find F1
Now substituting F2 back into equation (1):
F1=F2+10=30+10=40N
Final Answer
The two forces are:
- F1=40N
- F2=30N
We have two forces acting on a particle:
1. When they act in opposite directions, the resultant force is 10N.
2. When they act at right angles to each other, the resultant force is 50N.
From the first condition (forces acting in opposite directions), we can write:
F1−F2=10(1)
Assuming F1>F2.
√F21+F22=50(2)
Squaring both sides gives:
F21+F22=2500(3)
From equation (1), we can express F1 in terms of F2:
F1=F2+10
Now substitute this expression for F1 into equation (3):
(F2+10)2+F22=2500
Expanding (F2+10)2:
F22+20F2+100+F22=2500
Combining like terms:
2F22+20F2+100=2500
Subtracting 2500 from both sides:
2F22+20F2−2400=0
Dividing the entire equation by 2:
F22+10F2−1200=0
To solve for F2, we can use the quadratic formula:
F2=−b±√b2−4ac2a
Where a=1, b=10, and c=−1200:
F2=−10±√102−4⋅1⋅(−1200)2⋅1
Calculating the discriminant:
F2=−10±√100+48002
F2=−10±√49002
F2=−10±702
Calculating the two possible values:
1. F2=602=30
2. F2=−802=−40 (not physically meaningful since force cannot be negative)
Now substituting F2 back into equation (1):
F1=F2+10=30+10=40N
The two forces are:
- F1=40N
- F2=30N
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise 3.213videos
VECTORS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Subjective28videos
VECTORS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer9videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)4videos
WORK, POWER & ENERGY
Similar Questions
Two forces of 45 kg-f and 40 kg-f act on a body In opposite directions. What Is the resultant force ?
View SolutionThe resultant of two forces at right angles is 13N. The minimum resultant of the two forces is 7 N. The forces are
View SolutionA body is acted upon by two forces each of magnitude F, but in opposite directions. State the effect of the forces if
(a) both forces act at the same point of the body.
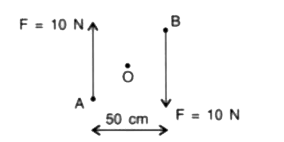
(b) the two forces act at two different points of the body at a separation?View SolutionFig.1.31 shows two forces each of magnitude 10N acting at points A and B at a separation of 50cm, in opposite directions. Calculate the resultant moment of the two forces about the point (i) A (ii) B and (iii) O situtated exactly at the middle of the two forces.
 View Solution
View SolutionTwo forces act at a point and are such that if the direction of one is reversed, the resultant is turned through a right angle. Show that the two forces must be equal in magnitude.
View SolutionTwo forces whose magnitudes are in the ratio 3:5 give a resultant of 28N. If the angle of their inclination is 60∘, find the magnitude of each force.
View SolutionTwo forces whose magnitudes are in the ratio 3:5 give a resultant of 28N. If the angle of their inclination is 60∘, find the magnitude of each force.
View SolutionTwelve forces each of 5 N act on a body simultaneously. If each force makes an angle of 30° with other their resultant is
View SolutionThe sum of the magnitudes of two forces acting at a point is 18 and the magnitude of their resultant is 12. If the resultant is at 90∘ with the force of smaller magnitude, What are the magnitudes of forces?
View SolutionThe sum of the magnitudes of two forces acting at a point is 18 and the magnitude of their resultant is 12. If the resultant is at 90∘ with the force of smaller magnitude, What are the magnitudes of forces?
View Solution

