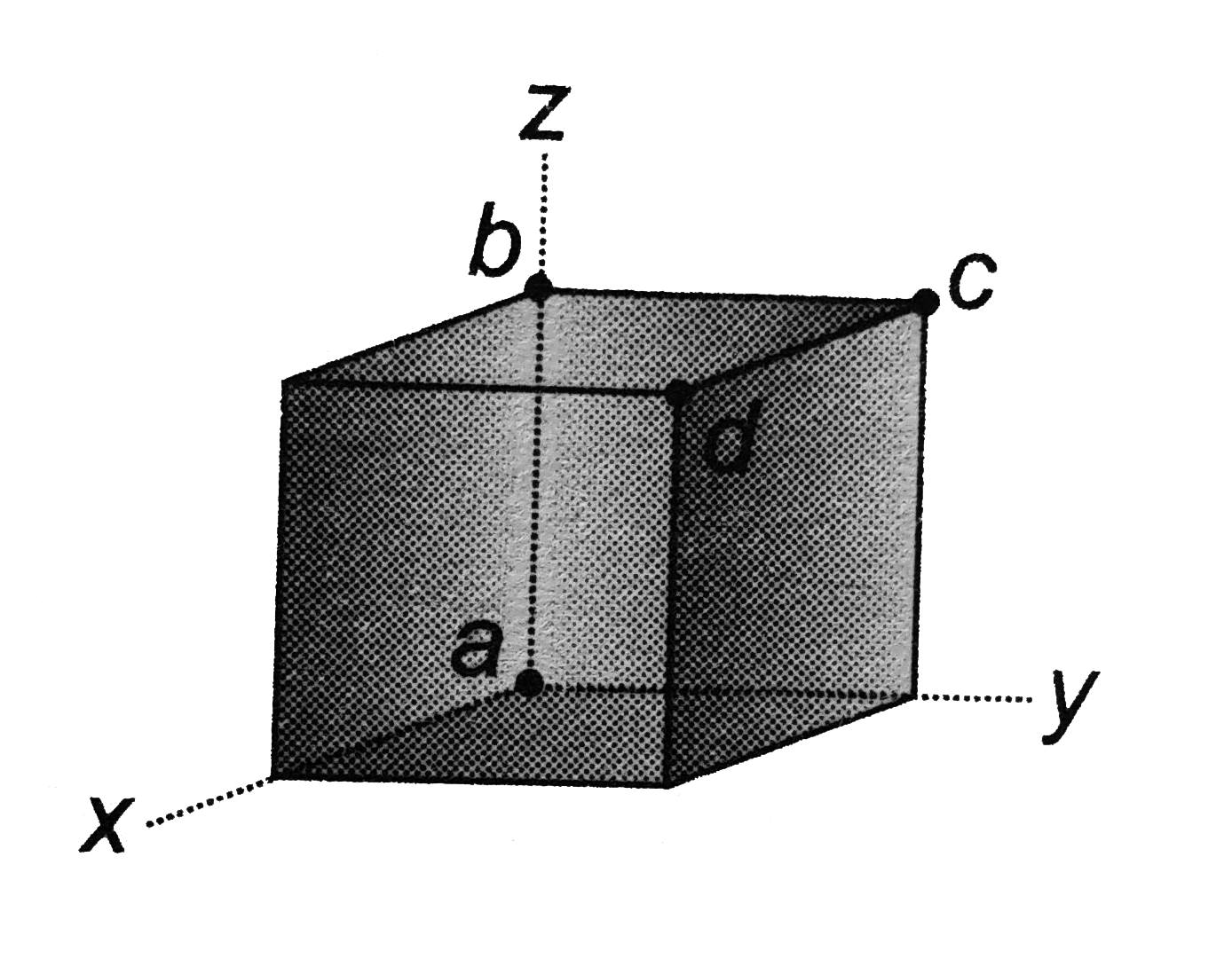
A cube is placed so that one corner is at the origin and three edges are along the x-,y-, and ,z-axes of a coordinate system (figure).Use vector to compute
a.The angle between the edge along the z-axis (line ab) and the diagonal from the origin to the opposite corner (line ad).
b. The angle between line ac (the diagonal of a face ) and line ad.
The correct Answer is:a.cos−1(1√3); b. cos^(-1)(sqrt(2)/sqrt(3))
a.Let side of the cube is d,then →ab=dˆk and −→ad=dˆi+dˆj+dˆk
cosθ=→ab.−→ad∣∣∣→ab∣∣∣∣∣∣−→ad∣∣∣=d2d√3d=1√3⇒θ=cos−1(1√3)
→ac=dˆj+dˆk
cosθ=→ac.−→ad∣∣→ac∣∣∣∣∣−→ad∣∣∣=2d2√2d√3d=√2√3
⇒cos−1(√2√2)
Topper's Solved these Questions
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Single Correct
51 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise 3.2
13 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
Similar Questions
Find the equation of the straight line passing through the origin and bisecting the portion of the line ax+by+c=0 intercepted between the coordinate axes.
View SolutionA cube of side l has one corner at the origin of coordinates and extends along the positive x−,y−andz−axes. Suppose that the electric field in this region is given by →E=(a+by)ˆj. Determine the charge inside the cube (a and b are some constants).
View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any edge and a face not containing the edge is
View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any two faces is
View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The value of [→a→b→c]2 is
View SolutionA straight line L at a distance of 4 units from the origin makes positive intercepts on the coordinate axes and the perpendicular from the origin to this line makes an angle of 60∘ with the line x+y=0. Then, an equation of the line L is
View SolutionA particle of mass m moves in the XY plane with constant velocity v along the straight line PQ as shown in the figure. Its angular momentum with respect to origin O is(θ is angle between→v and x-axis)

View SolutionA line forms a triangle of area 54√3square units with the coordinate axes. Find the equation of the line if the perpendicular drawn from the origin to the line makes an angle of 600 with the X-axis.
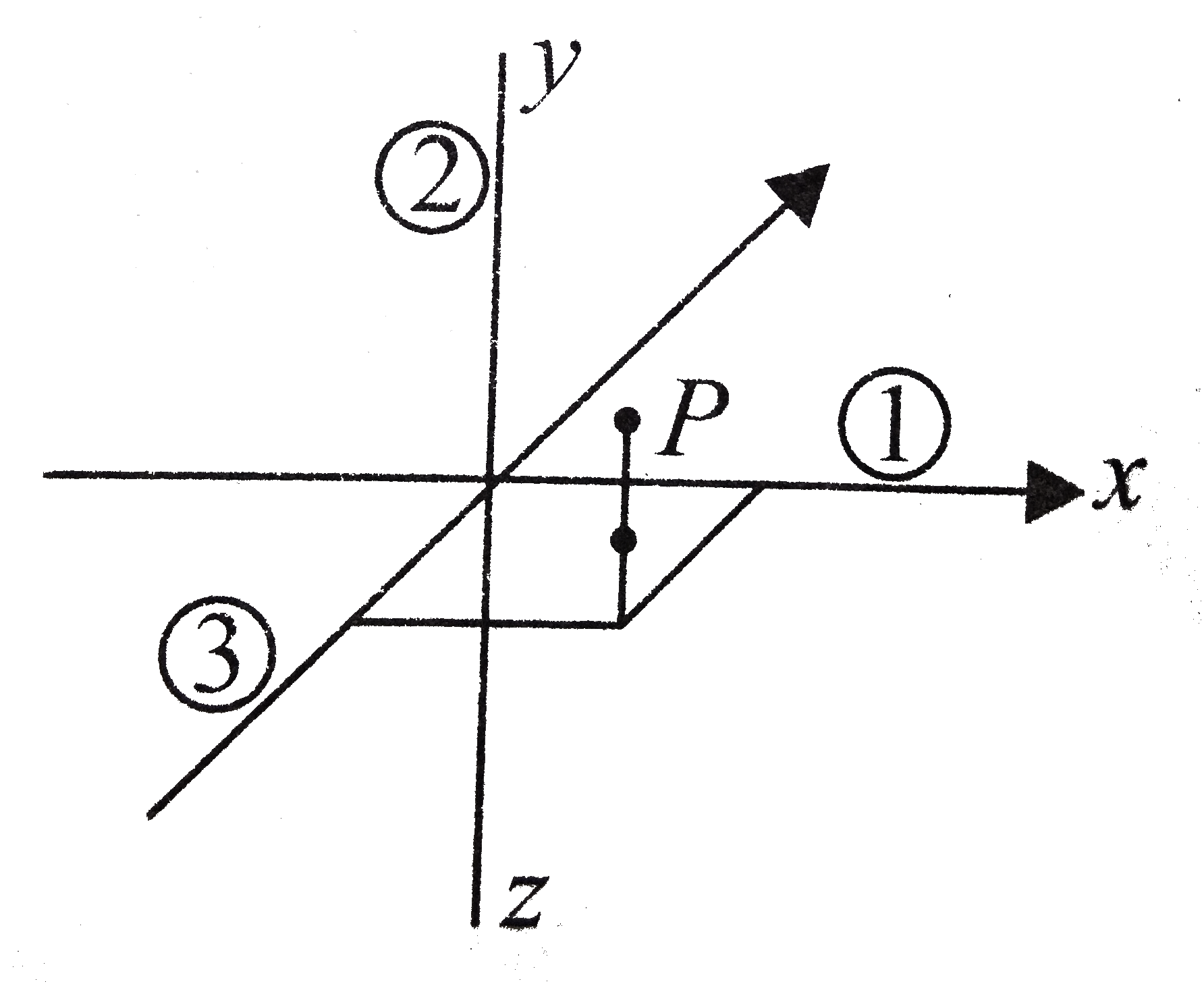
View SolutionFind the electric field vector at P (a,a,a) due to three infinitely long lines of charges along the x-, y- and z-axis, respectively. The charge density i.e. charge per unit length of each wire is (λ),
View SolutionA circle of radius 5 units has diameter along the angle bisector of the lines x+y=2 and x−y=2. If the chord of contact from the origin makes an angle of 450 with the positive direction of the x-axis, find the equation of the circle.
View Solution
