Figure shows a pulley over which is string passes and connected to two masses A and B. Pulley moves up with a velocity VP and mass B is also going up at a velocity VB.
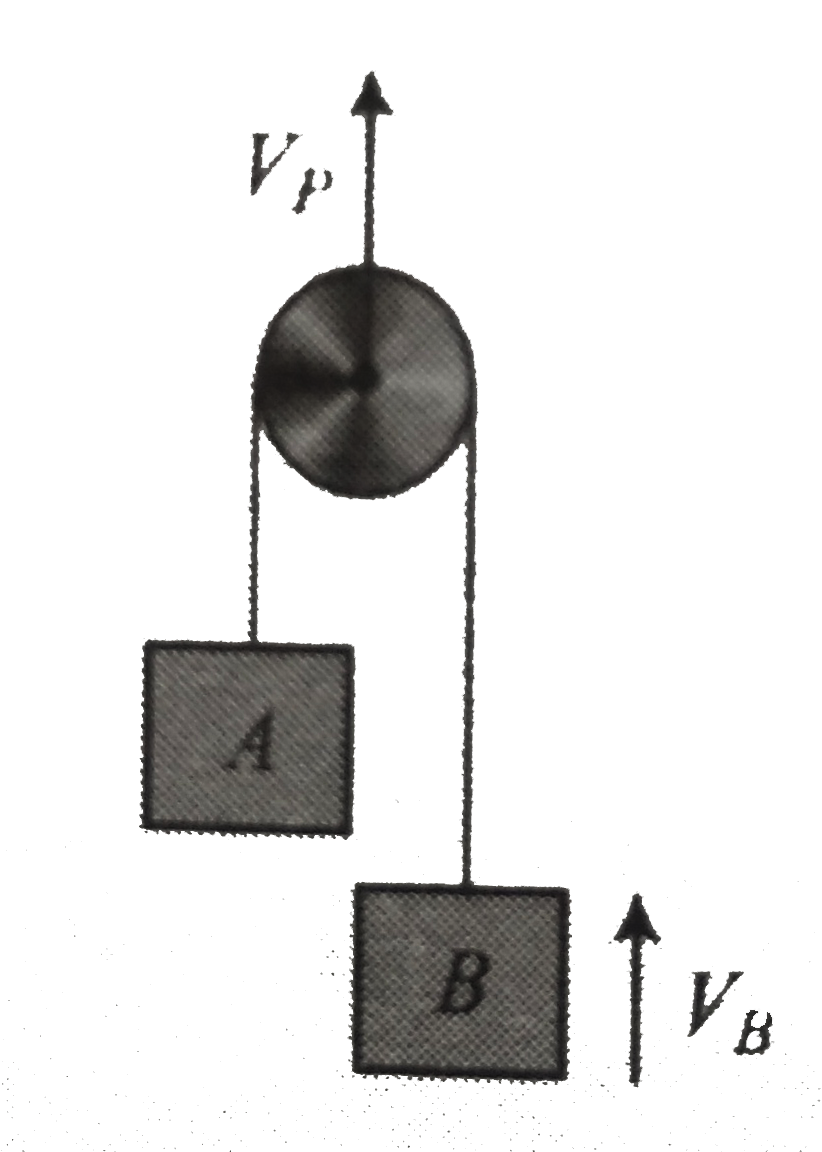
Find the velocity of mass A if
(a) VP=5ms−1 and VB=10ms−1
(b) VP=5ms−1 and VB=−20ms−1.
(a)VP=VA+VB2⇒5=VA+102⇒VA=0
(b) 5=VA−202⇒VA=30ms−1 .
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.4
13 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective
15 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.2
35 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
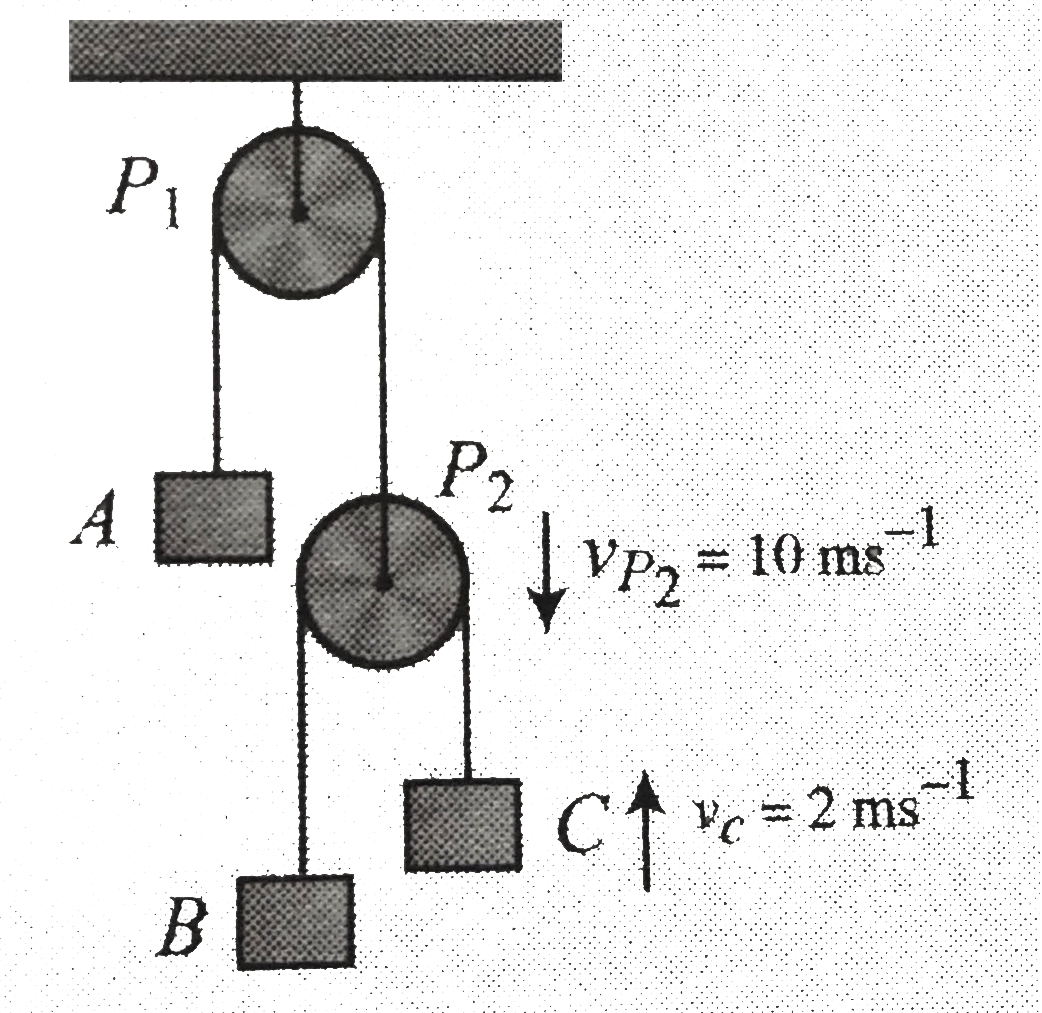
The three block shown in fig. move with constant velocities. Find the velocity of blocks A and B. given VP2=10ms−1⏐↓,VC=2ms−1↑⏐.
View SolutionA man A moves due to East with velocity 6ms−1 and another man B moves in N−30∘E with 6ms−1 . Find the velocity of B w.r.t. A.
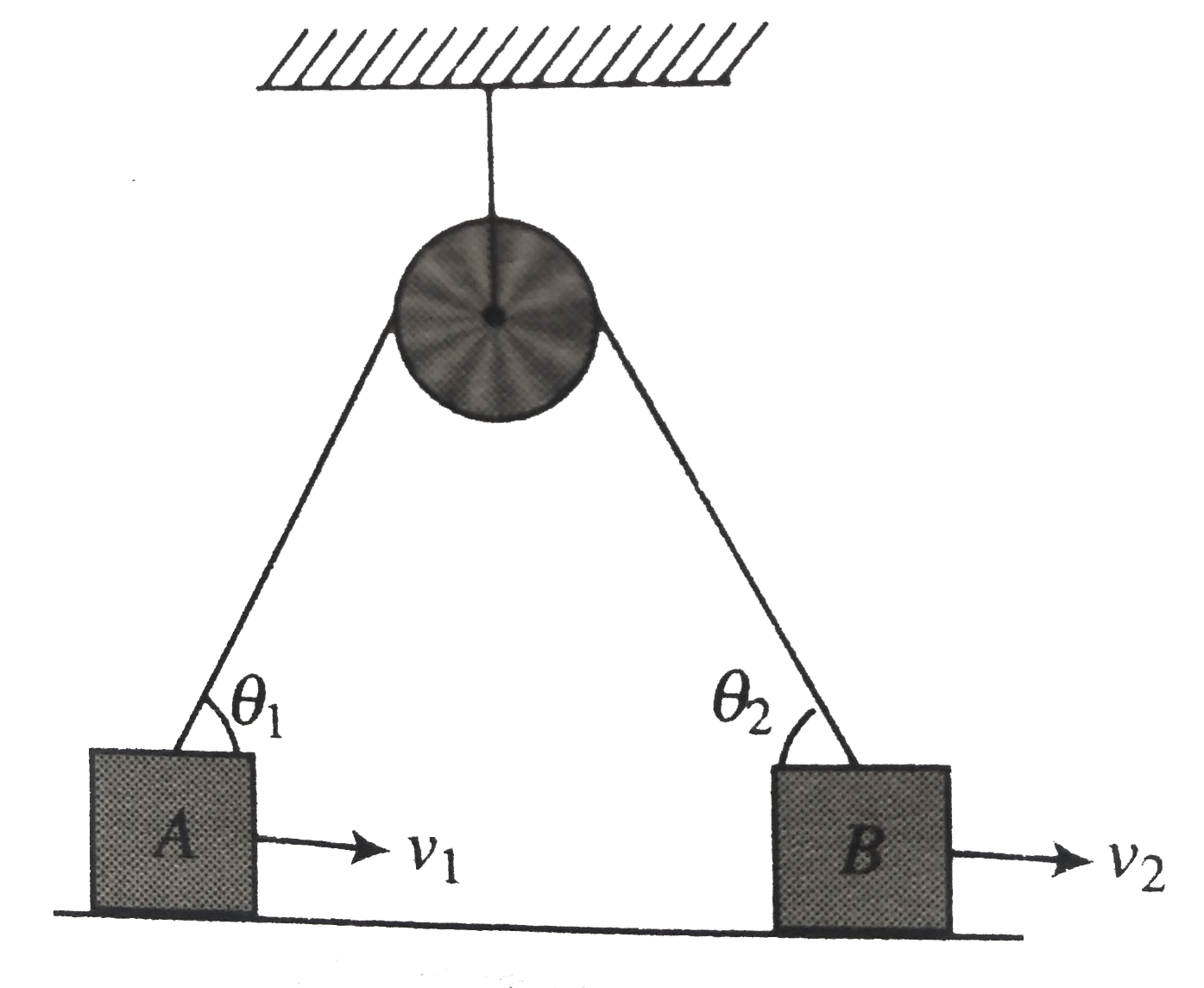
View SolutionIn fig., blocks A and B move with velocities v1 and v2 along horizontal direction. Find the ratio of v1/v2
View SolutionIn a reference frame a man A is moving with velocity (3ˆi−4ˆj)ms−1 and another man B is moving with velocity (ˆi+ˆj)ms−1 relative to A. Find the actual velocity of B.
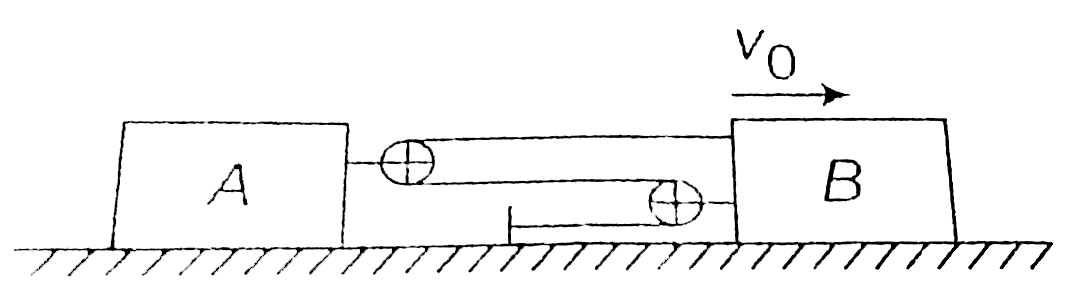
View SolutionBlock B moves to the right with a constant velocity v0. The velocity of body a relative to b is
View SolutionIn the system shown, the block A is moving down eith velocity v1 and C is moving up with velocity v3. Express velocity of the block B in terms of velocities of the blocks a and C.
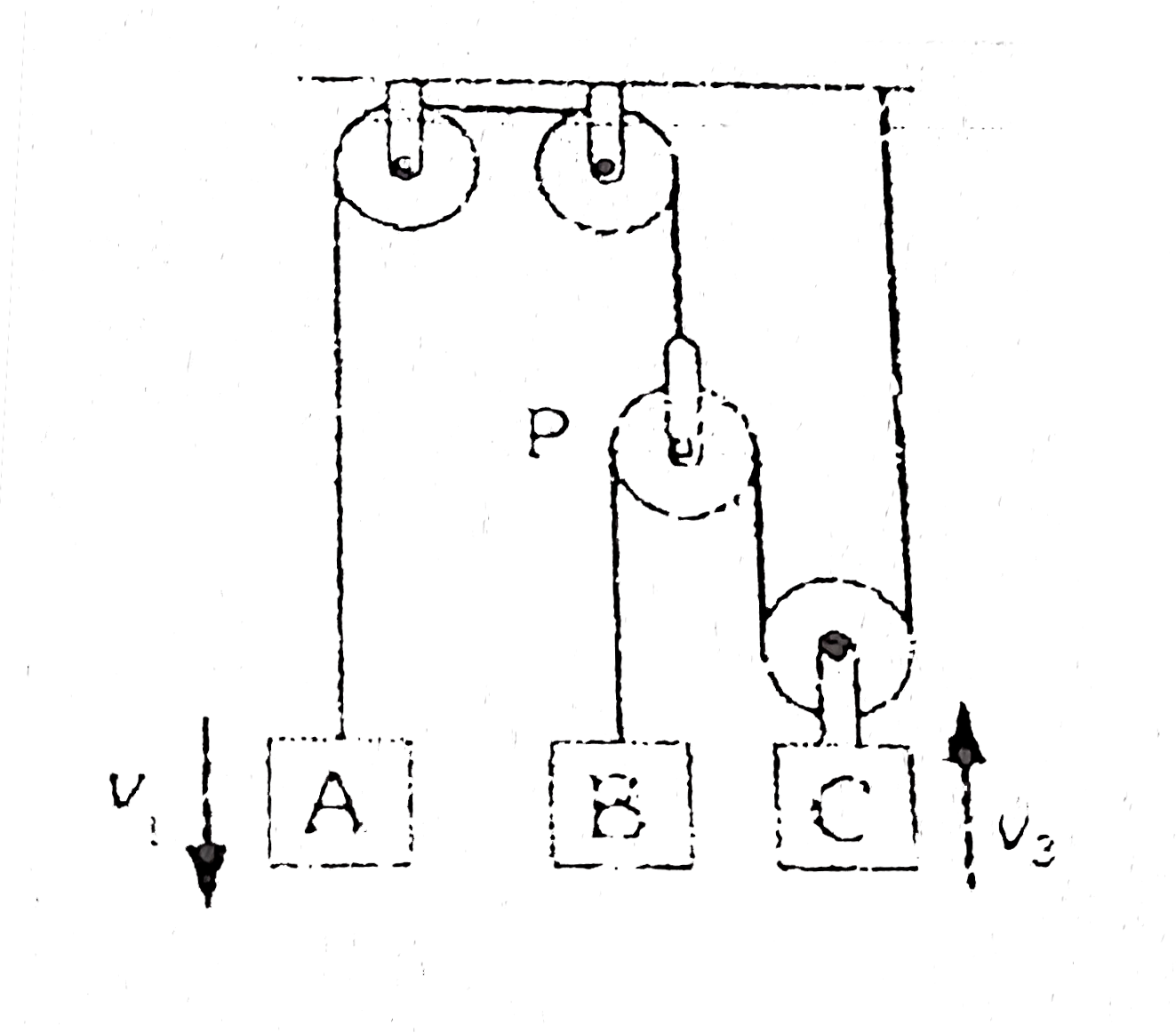
View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View SolutionTwo objects A and B are moving in opposite directions with velocities vAandvB respectively, the magnitude of relative velocity of A w.r.t. B is
View SolutionTwo balls AandB of same masses are thrown from the top of the building A. Thrown upward with velocity VandB, thrown downward with velocity V, then
View SolutionA particle of mass m is moving with a uniform velocity v1. It is given an impulse such that its velocity becomes v2. The impulse is equal to
View Solution
