An object is suspended from a spring balance in a lift. The reading is 240 N when the lift is at rest. If the spring balance reading now change to 220N, then the lift is moving
Downward with constant speed
Downward with decreasing speed
Downward with increasing speed
Upward with increasing speed
The correct Answer is:C
To solve the problem, we need to analyze the situation of an object suspended from a spring balance in a lift. The reading on the spring balance changes based on the motion of the lift.
1. Understanding the Initial Condition:
- When the lift is at rest, the reading on the spring balance is 240 N. This indicates that the weight of the object (mg) is equal to the reading on the spring balance.
- Therefore, we can conclude that the actual weight of the object is:
mg=240N
2. Analyzing the Change in Reading:
- When the lift is moving, the reading on the spring balance changes to 220 N. This indicates that the tension (T) in the spring balance is now less than the weight of the object.
- We have:
T=220N
3. Identifying the Motion of the Lift:
- Since the reading on the spring balance (220 N) is less than the weight of the object (240 N), it suggests that the lift is accelerating downwards.
- According to Newton's second law, if the lift is moving downwards with increasing speed, the net force acting on the object can be expressed as:
mg−T=ma
- Here, mg is the weight of the object, T is the tension (reading on the spring balance), and a is the acceleration of the lift.
4. Calculating the Acceleration:
- Rearranging the equation gives us:
mg−T=ma⟹240N−220N=ma⟹20N=ma
- This means the net force acting on the object is 20 N, which is causing it to accelerate downwards.
5. Conclusion:
- Since the reading on the spring balance decreased from 240 N to 220 N, we conclude that the lift is moving downwards with increasing speed. Thus, the correct answer is that the lift is moving downwards with increasing speed.
Final Answer:
The lift is moving downward with increasing speed.
---
- When the lift is at rest, the reading on the spring balance is 240 N. This indicates that the weight of the object (mg) is equal to the reading on the spring balance.
- Therefore, we can conclude that the actual weight of the object is:
mg=240N
- When the lift is moving, the reading on the spring balance changes to 220 N. This indicates that the tension (T) in the spring balance is now less than the weight of the object.
- We have:
T=220N
- Since the reading on the spring balance (220 N) is less than the weight of the object (240 N), it suggests that the lift is accelerating downwards.
- According to Newton's second law, if the lift is moving downwards with increasing speed, the net force acting on the object can be expressed as:
mg−T=ma
- Here, mg is the weight of the object, T is the tension (reading on the spring balance), and a is the acceleration of the lift.
- Rearranging the equation gives us:
mg−T=ma⟹240N−220N=ma⟹20N=ma
- This means the net force acting on the object is 20 N, which is causing it to accelerate downwards.
- Since the reading on the spring balance decreased from 240 N to 220 N, we conclude that the lift is moving downwards with increasing speed. Thus, the correct answer is that the lift is moving downwards with increasing speed.
The lift is moving downward with increasing speed.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Multiple Correct11videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Assertion-reasoning15videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective15videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
The reading in the spring balance is
 View Solution
View SolutionThe reading in the spring balance is:
 View Solution
View SolutionA body is suspended from a spring balance kept in a satellite. The reading of the balance is W1 when the satellite goes in an orbit of radius R and is W2 when it goes in an orbit of radius 2R.
View SolutionWhat is the reading of the spring balance in the following device?
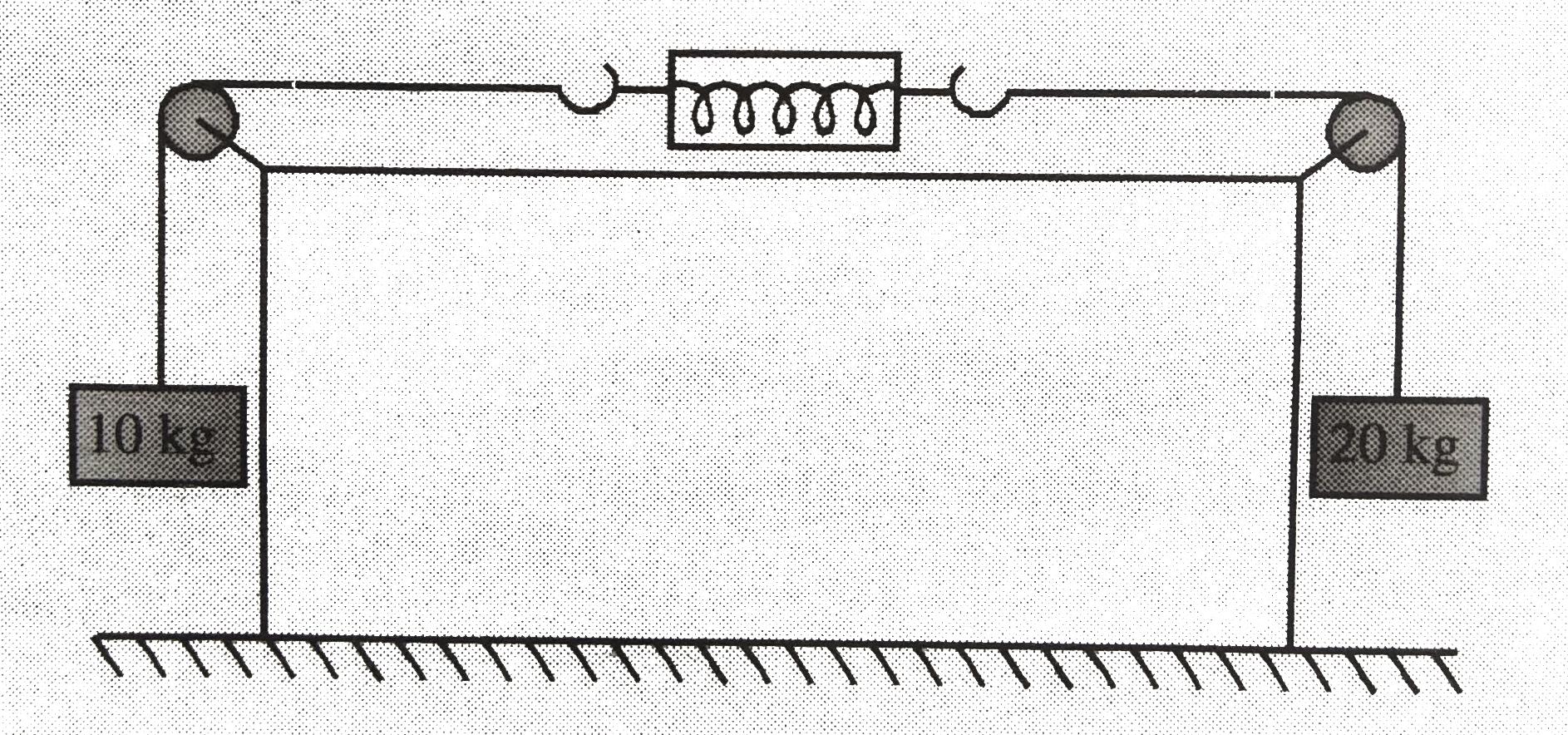 View Solution
View SolutionA body suspended on a spring balance in a ship weighs W0 when the ship is at rest. When the ship begins to move along the equator with a speed v, show that the scale reading is very close to W0(1±2ωV/g), where ω is the angular speed of the earth.
View SolutionA body of mass 50 kg is suspended using a spring balance inside a lift at rest. If the lift startts falling freely, the reading of the spring balance is
View SolutionA block of mass 4 kg and volume 5×10−4m3 is suspended by a spring balance in a lift which is accelerating. The apparent weight shown by the spring balance is 3 kg. Now the block is immersed in water in kg shown by the spring balance is
View SolutionFor the arrangement shown in the figure, the reading of spring balance is
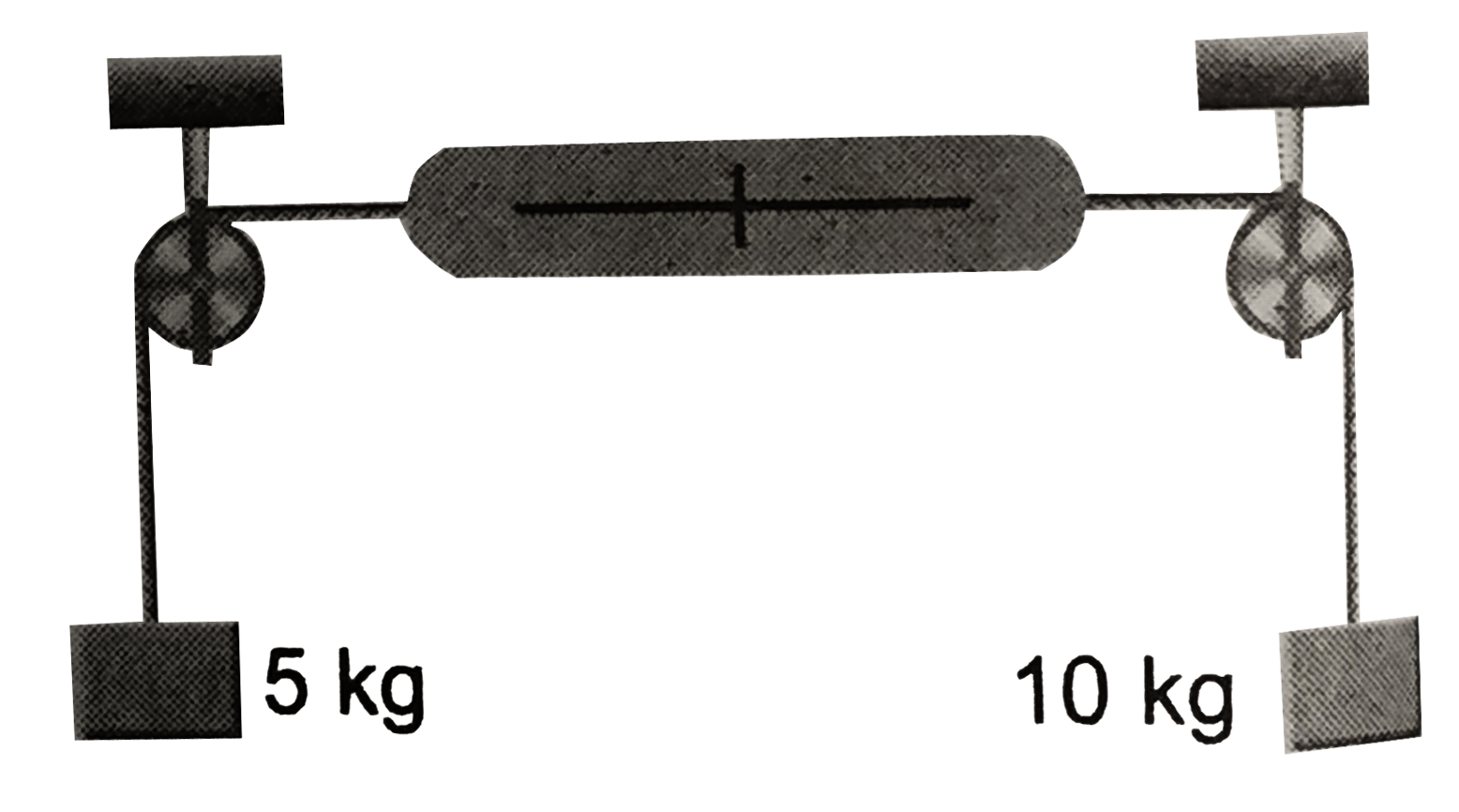 View Solution
View SolutionA body of mass 50 kg is hung by a spring balance in a lift. Calculate the reading of the balance when :
The lift is ascending with an acceleration of 2m/s2.View SolutionWhen a piece of wood is suspended from the hook of a spring balance, it reads 70 gf. The wood is now lowered into water. What reading do you expect on the scale of spring balance?
View Solution

