A block of mass 1kg is tied to a string of length 1m the other end of which is fixed The block is moved on a smooth horizontal table with constant speed 10ms−1. Find the tension in the string
To find the tension in the string when a block of mass 1 kg is tied to a string of length 1 m and is moving with a constant speed of 10 m/s on a smooth horizontal table, we can follow these steps:
1. Identify the Forces Acting on the Block:
- The block is moving in a circular path due to the tension in the string. The only force providing the necessary centripetal force is the tension (T) in the string.
2. Understand the Concept of Centripetal Force:
- When an object moves in a circular path, it requires a centripetal force directed towards the center of the circle. This force can be calculated using the formula:
Fc=mv2r
- Where:
- Fc is the centripetal force,
- m is the mass of the object,
- v is the velocity of the object,
- r is the radius of the circular path.
3. Substitute the Known Values:
- In this case:
- Mass m=1kg
- Velocity v=10m/s
- Radius r=1m
- Plugging these values into the centripetal force formula gives:
Fc=1kg×(10m/s)21m
4. Calculate the Centripetal Force:
- Calculate (10m/s)2=100m2/s2.
- Now substituting back:
Fc=1kg×100m2/s21m=100N
5. Conclusion:
- The tension in the string, which acts as the centripetal force, is therefore:
T=100N
Final Answer:
The tension in the string is 100 Newtons.
---
- The block is moving in a circular path due to the tension in the string. The only force providing the necessary centripetal force is the tension (T) in the string.
- When an object moves in a circular path, it requires a centripetal force directed towards the center of the circle. This force can be calculated using the formula:
Fc=mv2r
- Where:
- Fc is the centripetal force,
- m is the mass of the object,
- v is the velocity of the object,
- r is the radius of the circular path.
- In this case:
- Mass m=1kg
- Velocity v=10m/s
- Radius r=1m
- Plugging these values into the centripetal force formula gives:
Fc=1kg×(10m/s)21m
- Calculate (10m/s)2=100m2/s2.
- Now substituting back:
Fc=1kg×100m2/s21m=100N
- The tension in the string, which acts as the centripetal force, is therefore:
T=100N
The tension in the string is 100 Newtons.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples12videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.125videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
Knowledge Check
- Question 1 - Select One
A stone of mass 5 kg is tied to a string of length 10 m is whirled round in a horizontal circle. What is the maximum speed with which the stone can be whirled around if the string can withstand a maximum tension of 200 N?
A10ms−1B15ms−1C20ms−1D25ms−1
A block of mass m is tied to a string of length L and the assembly is kept on a smooth horizontal table as shown in the figure. Now the other end of the string is moved with a constant velocity v0ˆj. (xy plane is the plane of the table) Then
 View Solution
View SolutionA stone of mass 1 kg tied at the end of a string of length 1 m and is whirled in a verticle circle at a constant speed of 3ms−1. The tension in the string will be 19 N when the stone is (g=10ms−1)
View SolutionA block is tied to one end of a light string of length 1 whose other end is fixed to a rigid support. The block is given a speed of √7gl/2 from the lowermost position. Find the height and speed at which the block leaves the circle. Also find the maximum height to which it rises finally.
View SolutionA stone of mass 0.1 kg tied to one end of a string lm long is revolved in a horizontal circle at the rate of 10π rev/s. Calculate the tension in the string.
View SolutionA bullet of mass 0.01kg and travelling at a speed of 500ms−1 strikes a block of mass 2kg which is suspended by a string of length 5m. The centre of gravity of the block is found to raise a vertical distance of 0.2m. What is the speed of the bullet after it emerges from the block?
View SolutionTwo charged particles having charge 2.0×10−8C each are joined by an insulating string of length 1 m and the system is kept on a smooth horizontal table. Find the tension in the string.
View SolutionIn the figure shown, a person pulls a light string with a constant speed u=10m/s. The other end of the string is tied to a very small block which moves on a smooth horizontal surface. Find the angle 'θ'when the block 3 leaves the surface . Take g=10m/s2.
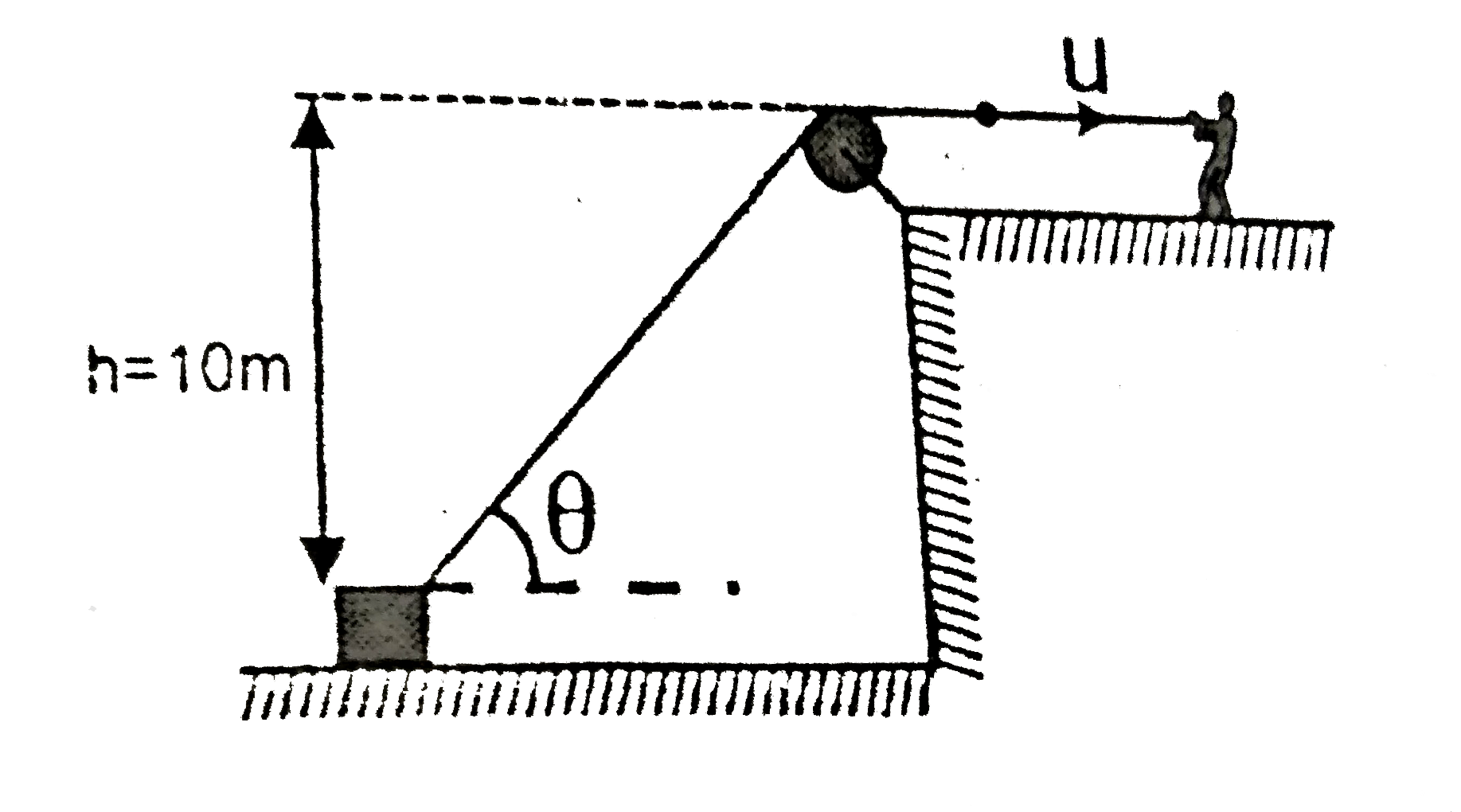 View Solution
View Solution


