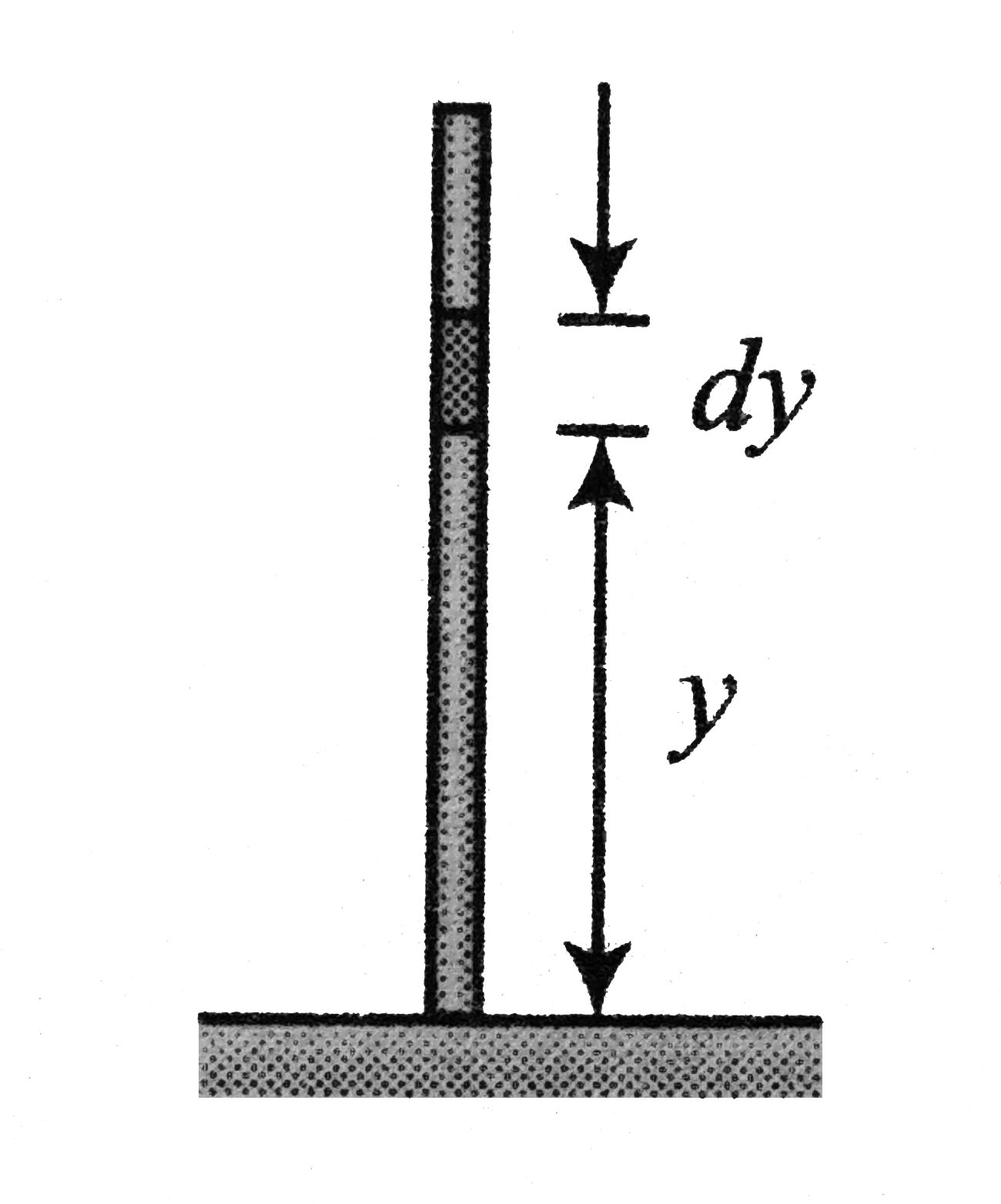
A rod of mass m and length l is held vertical. Find its gravitational potential energy with respect to zero potential energy at its lowest end.
Since parts of the rod are at different levels from the horizontal surface, therefore, we have to use the integration to find its potential energy.
Consider a small element of length dy at a height y from the surface.
Mass of the element is dm=MLdy
Potential energy of the element is
dU=(dm)gy=MLgydy
On integrating, we get
U=MgLL∫0ydy=MgL[y22]L0=12MgL
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Solved Examples
15 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
The gravitational potential energy is maximum at :
View SolutionDifferentiate between gravitational potential energy and elastic potential energy.
View SolutionShow that at infinity, gravitational potential energy becomes zero.
View SolutionDefine gravitational potential and gravitational potential energy.
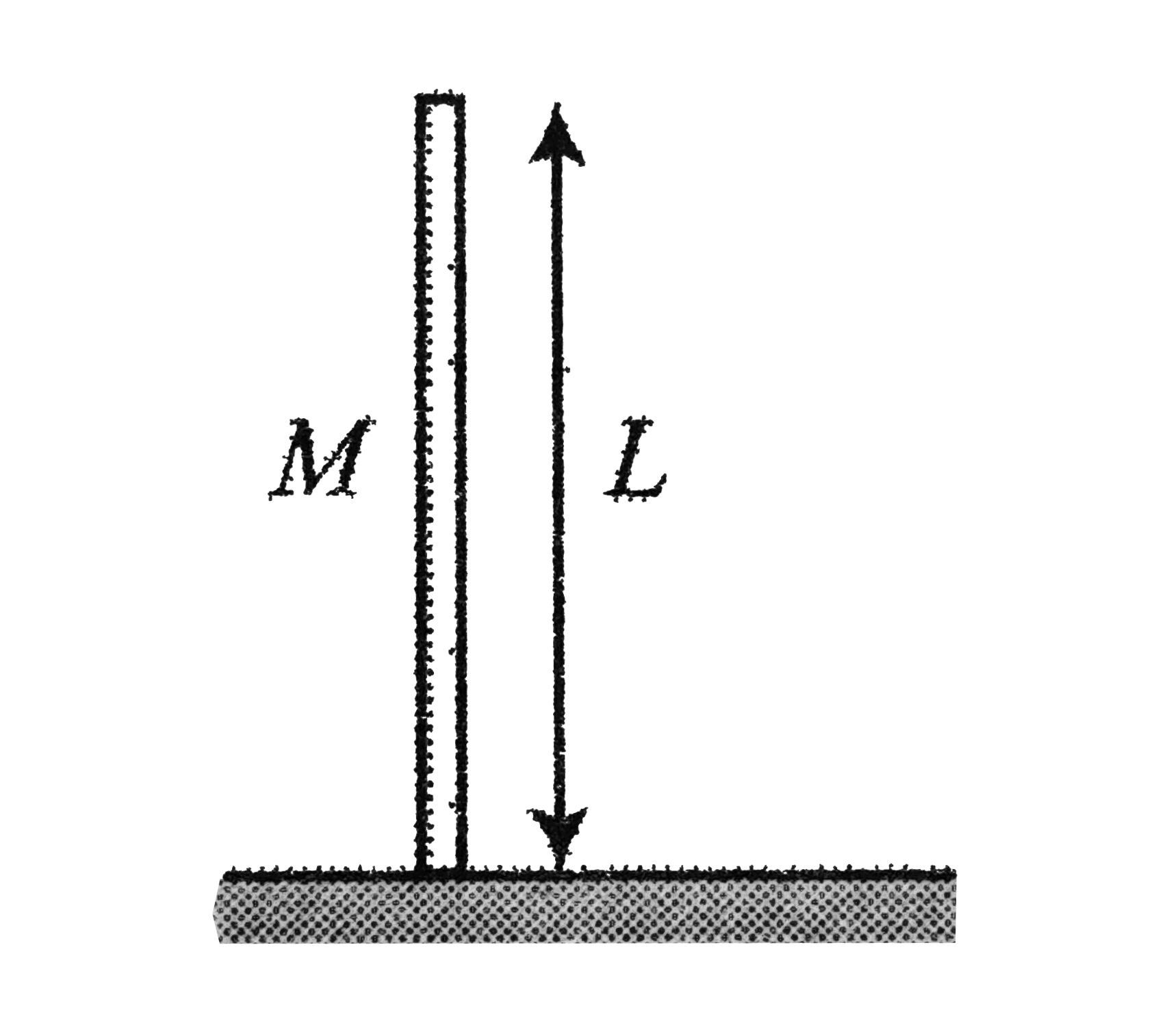
View SolutionA uniform rod of mass M and length L is held vertically upright on a horizontal surface as shown in figure. Assuming zero potential energy at the base of the rod, determine the potential energy of the rod.
View SolutionA rod of mass M and length L is made to stand vertically. Potential energy of the rod in this position is
View SolutionA rod of length L and mass m is kept vertically on the ground. Its potential energy is :
View SolutionA stick of mass m and length l is pivoted at one end and is displaced through an angle θ. The increase in potential energy is
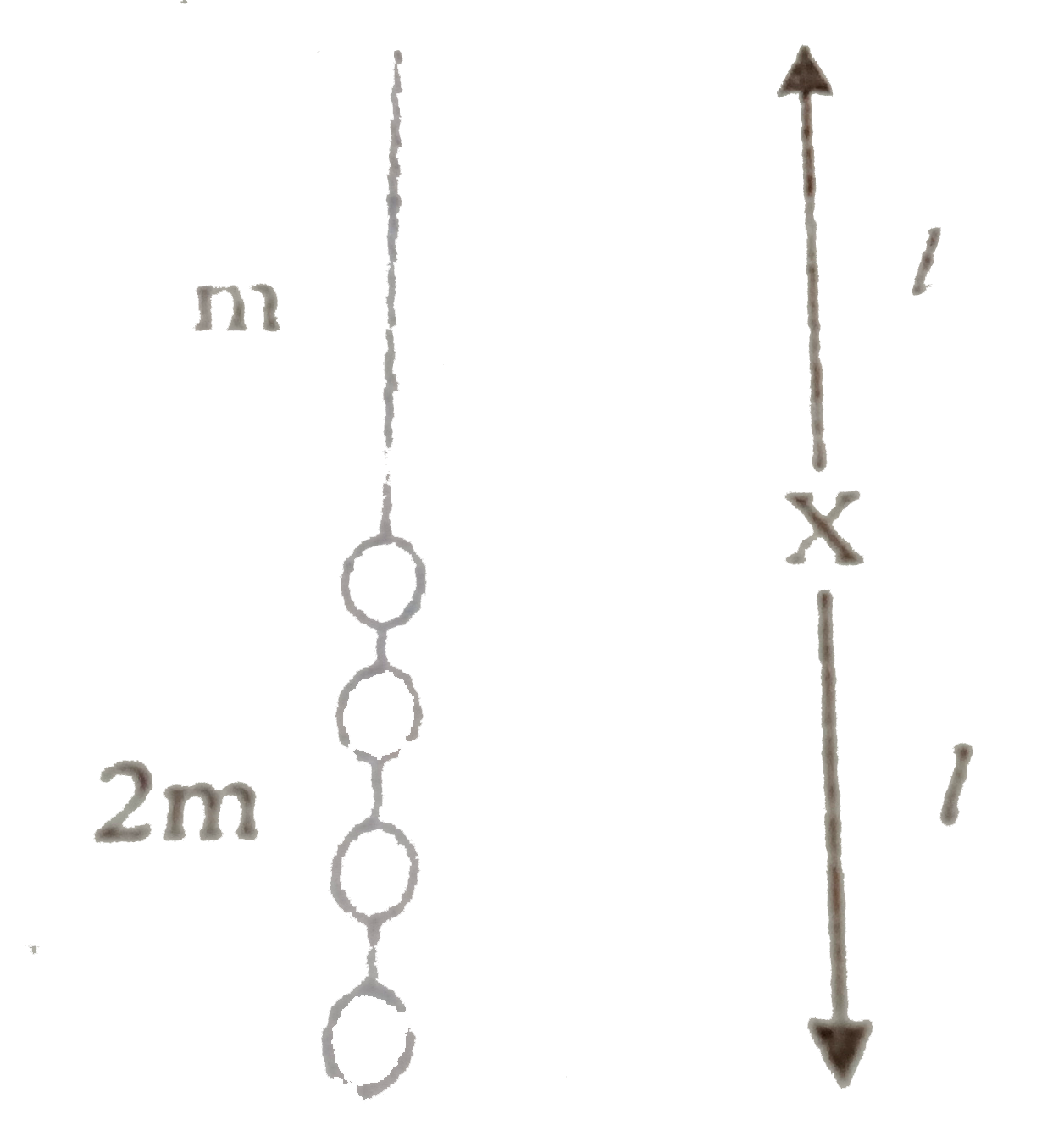
View SolutionA rope of length l and mass 'm' is connected to a chain of length l and mass 2m and hung vertically as shown. What is the change in graviational potential energy if the system is inverted and hung from same point.
 .
.
View SolutionTwo bodies of equal masses are placed at heights and 2h. Find the ratio of their gravitational potential energies
View Solution
