Question

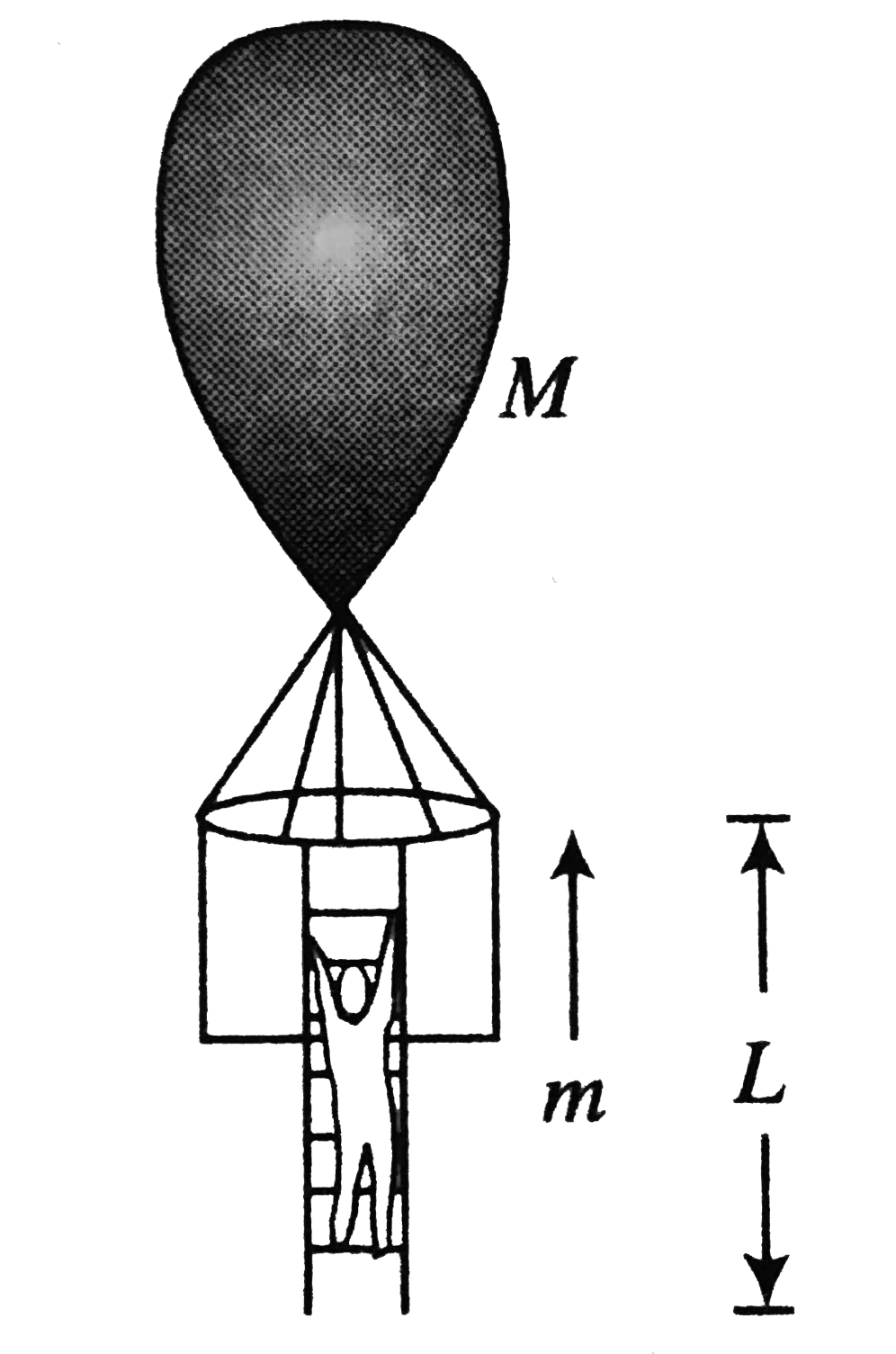
A ladder of length $l$ carrying a man of mass $m$ at its end is attached to the basket of a balloon of mass M. The entire system is in equilibrium in the air. As the man climbs up the ladder into the balloon, the balloon descends by a height h.
The potential energy of the man

Options

A. (a) Increases by `mg(l-h)`
B. (b) Increases by `mgl`
C. (c) Increases by `mgh`
D. (d) Increases by `mg(2l-h)`

Answer

A

Explanation

To solve the problem step by step, we will analyze the situation involving the ladder, the man, and the balloon.

Step 1: Understand the System
We have a ladder of length \( l \) with a man of mass \( m \) at its end. The ladder is attached to a balloon of mass \( M \). The entire system is in equilibrium in the air.

Hint: Identify the components of the system and their masses.

Step 2: Analyze the Climbing Action
When the man climbs the ladder, he moves upwards. As he climbs, the balloon descends by a height \( h \).

Hint: Consider the conservation of energy and how the movement of the man affects the balloon.

Step 3: Calculate the Potential Energy Change
The potential energy of the man when he climbs to a height \( l \) is given by:
\( \Delta PE_{\text{man}} = mgh \)
where \( h \) is the height he climbs.

Hint: Remember that potential energy is calculated using the formula \( PE = mgh \).

Step 4: Consider the Balloon's Descent
As the man climbs, the balloon descends by height \( h \). The potential energy lost by the balloon is:
\( \Delta PE_{\text{balloon}} = Mgh \)

Hint: The potential energy change for the balloon is also based on its mass and the height it descends.

Step 5: Set Up the Energy Balance Equation
Since the system is in equilibrium, the work done by the man climbing is equal to the potential energy lost by the balloon:
\( mgh = Mgh \)

Hint: This equation represents the balance of energy in the system.

Step 6: Solve for the Potential Energy of the Man
The total potential energy of the man when he climbs up is:
\( PE_{\text{man}} = mgl \)
The change in potential energy of the man as he climbs is:
\( \Delta PE_{\text{man}} = mgl - Mgh \)

Hint: Calculate the total potential energy considering both the man and the balloon.

Step 7: Conclusion
The potential energy of the man increases by:
\( \Delta PE = mgl - Mgh \)

Thus, the potential energy of the man increases by this amount as he climbs into the balloon.

Final Answer: The potential energy of the man increases by \( mgl - Mgh \).