A cart of mass M is tied to one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M2. The entire ststem is on a smooth horizontal surface. If the man pulls the cart by the rope then find the distance by which the man will slip on the horizontal surface before the man and the cart will meet each other.
203m
103m
0
None
The correct Answer is:A
To solve the problem, we can follow these steps:
Step 1: Understand the System
We have a cart of mass M tied to one end of a massless rope of length 10 m. The other end of the rope is held by a man of mass M2. The entire system is on a smooth horizontal surface, meaning there is no friction.
Step 2: Identify the Displacements
Let’s denote:
- The distance the man slips as x (to the left).
- The distance the cart moves as 10−x (to the right).
Step 3: Center of Mass Calculation
Since there are no external forces acting on the system, the center of mass (CM) of the system will not move. The formula for the center of mass xCM of the system is given by:
xCM=m1x1+m2x2m1+m2
Where:
- m1=M2 (mass of the man)
- x1=−x (displacement of the man)
- m2=M (mass of the cart)
- x2=10−x (displacement of the cart)
Step 4: Set Up the Equation
Since the center of mass does not move, we set the center of mass equation to zero:
(M2⋅(−x))+(M⋅(10−x))M2+M=0
Step 5: Simplify the Equation
The total mass of the system is:
M2+M=3M2
Substituting this into the equation gives:
−Mx2+10M−Mx3M2=0
Step 6: Clear the Denominator
Multiplying through by 3M2 to eliminate the denominator:
−Mx2+10M−Mx=0
Step 7: Combine Like Terms
Combine the terms involving x:
10M−3Mx2=0
Step 8: Solve for x
Rearranging gives:
10M=3Mx2
Dividing both sides by M (assuming M≠0):
10=3x2
Multiplying both sides by 2:
20=3x
Finally, divide by 3:
x=203 meters
Conclusion
The distance by which the man will slip before he and the cart meet is 203 meters.
---
We have a cart of mass M tied to one end of a massless rope of length 10 m. The other end of the rope is held by a man of mass M2. The entire system is on a smooth horizontal surface, meaning there is no friction.
Let’s denote:
- The distance the man slips as x (to the left).
- The distance the cart moves as 10−x (to the right).
Since there are no external forces acting on the system, the center of mass (CM) of the system will not move. The formula for the center of mass xCM of the system is given by:
- m1=M2 (mass of the man)
- x1=−x (displacement of the man)
- m2=M (mass of the cart)
- x2=10−x (displacement of the cart)
Since the center of mass does not move, we set the center of mass equation to zero:
The total mass of the system is:
Multiplying through by 3M2 to eliminate the denominator:
Combine the terms involving x:
Rearranging gives:
The distance by which the man will slip before he and the cart meet is 203 meters.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (ROTATIONAL MOTION)
72 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (GRAVITATION)
25 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (CIRCULAR MOTION)
17 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A cart of mass M is tied to one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M. The entire system is on a smooth horizontal surface. The man is at x=0 and the cart at x=10m. If the man pulls the cart by the rope, the man and the cart will meet at the point
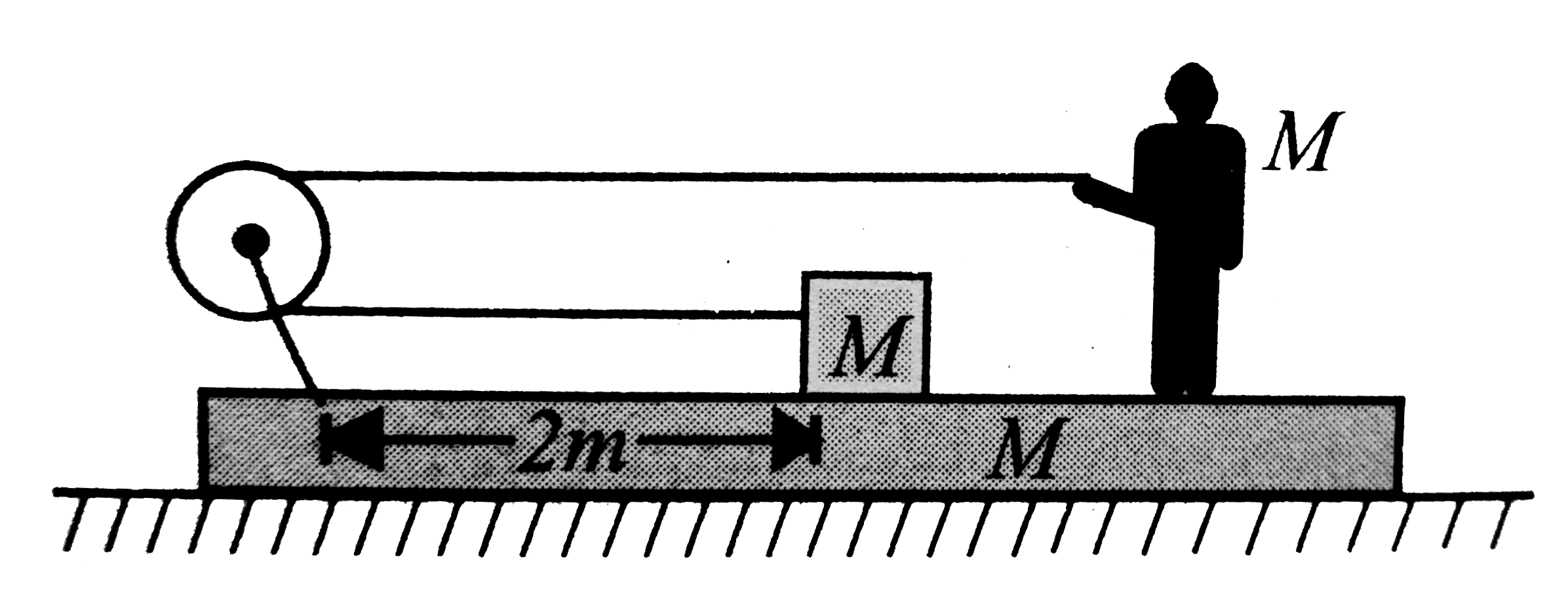
View SolutionA block of mass M is tied to one end of a massless rope. The other end of the rope is in the hands of a man of mass 2M as shown in Fig. The block and the man art resting on a rough wedge of mass M. The whole system is resting on a smooth horizontal surface. The man starts walking towards right while holding the rope in his hands. Pulley is massless and frictionless. Find the displacement of the wedge when the block meets the pulley. Assume wedge is sufficiently long so that man does not fall down.
View SolutionA man of mass m is standing at one end of a trolley of mass Mand length L placed at rest on a smooth horizontal surface. The man starts moving towards the other end and covers distance L, then distance moved by man w.r.t ground is
View SolutionA cat of mass m = 1 kg climbs to a rope hung over a leight frictionless pulley. The opposite end of the rope is tied to a weight of mass M = 2m lying on a smooth horizontal plane. What is the tension of the rope when the cat move upwards with in acceleration a=2m/s2 relative to the rope ?

View SolutionA man of mass m is standing on a plank of equal mass m resting on a smooth horizontal surface. The man starts moving on the plank with speed u relative to the plank. The speed of the man relative to the ground is

View SolutionA man of mass m stands at the left end of a uniform plank of length L and mass M, which lies on a frictionaless surface of ice. If the man walks to the other end of the plank, then by what distance does the plank slide on the ice?
View SolutionA man of mass m stands at the left end of a uniform plank of length L and mass M, which lies on a frictionaless surface of ice. If the man walks to the other end of the plank, then by what distance does the plank slide on the ice?
View SolutionA man of mass m stands at the left end of a uniform plank of length L and mass M, which lies on a frictionaless surface of ice. If the man walks to the other end of the plank, then by what distance does the plank slide on the ice?
View SolutionA plank of mas M and length L is at rest on as frictionless floor. The top surface of the plank has friction. At one end of it a man of mass m is standing as shown in figure. If the man walks towards the other end the find the distance, which the plank moves a till the man reaches the centre of the plank. b. till the man reaches the other end of the plank.

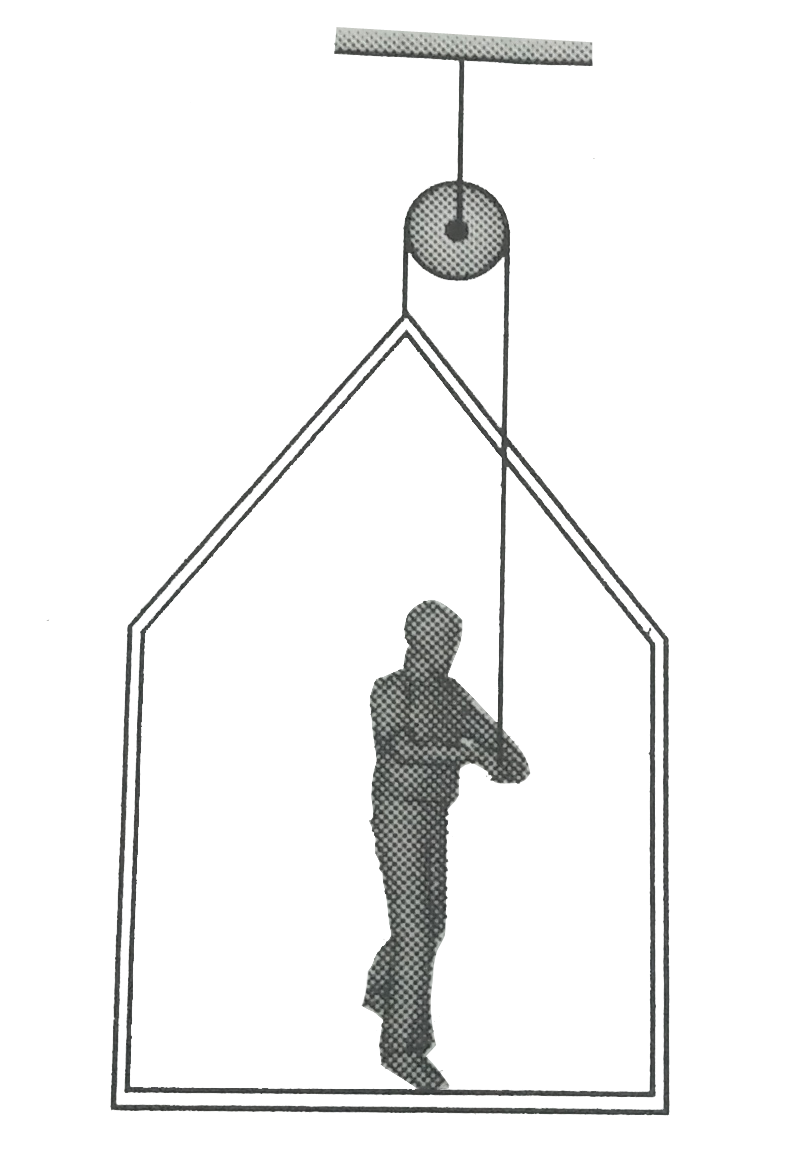
View SolutionA man of mass m stands on a frame of mass M. He pulls on a light rope, which passes over a pulley. The other end of the rope is attached to the frame. For the system to be in equilibrium, what force must the man exert on the rope?
View Solution
