Two particles of equal mass in are projected from the ground with speeds v1 and v2 at angles θ1, and θ2 as shown in the figure. Given θ2>θ1 and v1cosθ1=v2cosθ2Which statement/s is/are correct?
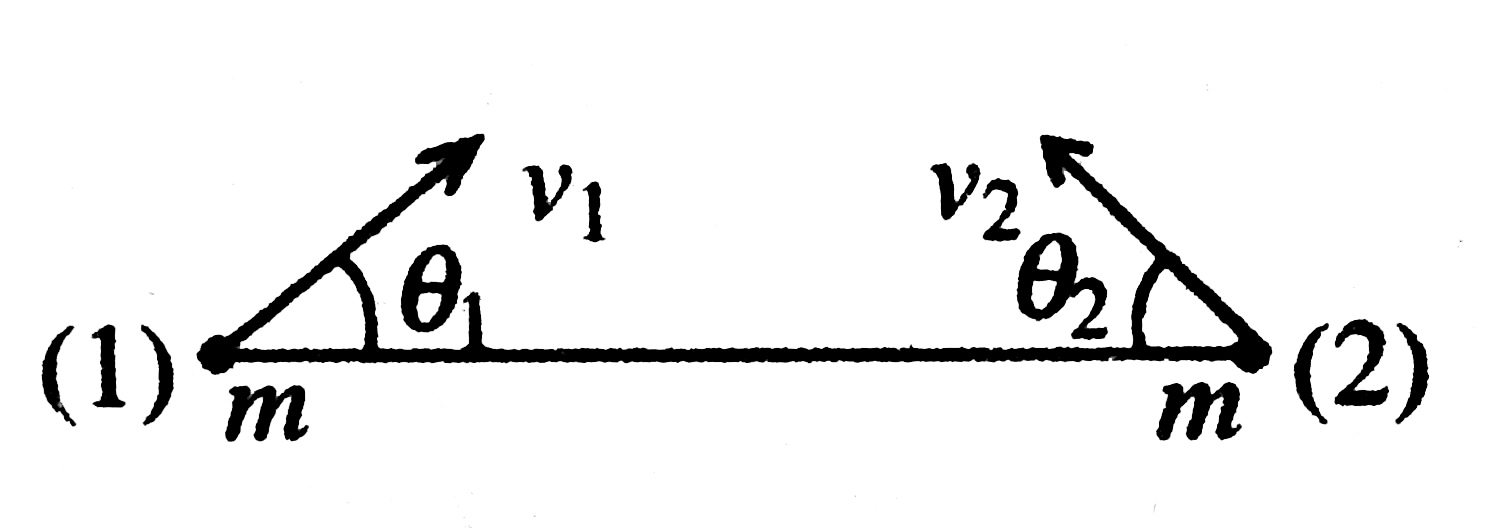
Centre of mass of particles will move along a vertical line.
Centre of mass of particles will move along a line inclined at some angle with vertical.
Particle '1' will be above vertical. centre of mass level when both particles are in air.
Particle '2' will be above centre of mass level both particles are in air.
The correct Answer is:A, D
vcm−x=mv1cosθ1m(−v2cosθ2)m+m=0
So horizontal velocity of centre of mass is zero. Hence centre of masss will move in vertical direction.
Now v2cosθ2=v1cosθ1
⇒v2v1=cosθ1cosθ2>1 (∵θ2>θ1)
⇒v2>v1
⇒v2sinθ2>>v1sinθ1v2sinθ2
At any time particle 2 will be greater height than particle 1. so particle 2 will be above centre of man level.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Linked Comprehension
105 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Single Correct
141 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Two particles of equal mass 'm' are projected from the ground with speed v1 and v2 at angles θ1 and θ2 at the same times as shown in figure. The centre of mass of the two particles.

View SolutionTrajectory of two particles projected from origin with speeds v1 and v2 at angles θ1 and θ2 with positive x- axis respectively are as shown in the figure. Given that (g=−10m/s2(ˆj)). Choose the correct option related to diagram.

View SolutionIf two particles of masses m1 and m2 are projected vertically upwards with speed v1 and v2, then the acceleration of the centre of mass of the system is
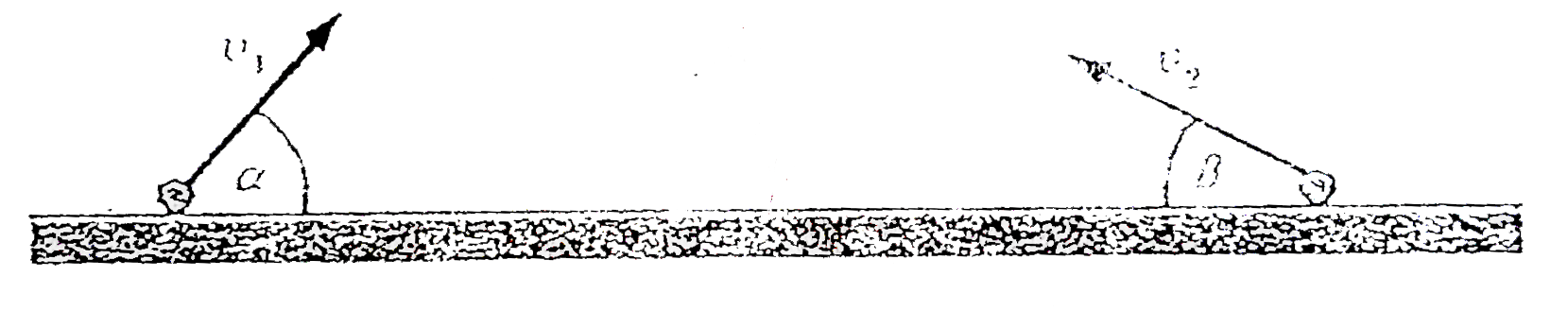
View SolutionTwo particles are projected at the same instant with speeds v1 and v2 making angles α and β with the horizontal as shown in the figure. Nelecting air resistance, the trajectory of the mass center the two particles
View Solutionsinθ1+cosθ+1+cosθsinθ=2cosecθ
View SolutionProve: 1+cosθ−sin2θsinθ(1+cosθ)=cotθ
View SolutionTwo particles are projected in air with speed v0 at angles θ1 and θ2 (both acute) to the horizontal,respectively.If the height reached by the first particle greater than that of the second,then thick the right choices
View SolutionTwo particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
View SolutionTwo projectiles are projected with velocity vA,vB at angles θA (from horizontal) and θB (from vertical) as shown in the figure below, such that vA>vB but having same horizontal component of velocity. Which of the following is correct ?

View SolutionProve that
1+cosθ−sin2θsinθ(1+cosθ)=cotθ
View Solution
