A wheel rolling along a rough horizontal surface with an angular velocity ω strikes a rough vertical wall, normally. Find the initial angular velocity, as it tends to roll up the wall (neglect any slippage) due to impulse.
To solve the problem of a wheel rolling along a rough horizontal surface with an angular velocity ω striking a rough vertical wall normally, we will use the principles of angular momentum and the conditions of pure rolling motion. Here’s a step-by-step solution:
Step 1: Understand the System
The wheel is rolling towards a vertical wall with an angular velocity ω. When it strikes the wall, it experiences an impulse that causes it to roll up the wall. We need to find the initial angular velocity as it tends to roll up.
Hint: Visualize the wheel's motion and the forces acting on it when it strikes the wall.
Step 2: Identify Forces and Points of Contact
When the wheel strikes the wall, we can denote:
- C1: the center of the wheel
- C2: the point of contact with the wall
The forces acting on the wheel include:
- Gravitational force mg acting downwards.
- Normal force N acting horizontally from the wall.
Hint: Draw a free-body diagram to identify all forces and their directions.
Step 3: Apply Conservation of Angular Momentum
We will apply the conservation of angular momentum about point C2 (the point of contact with the wall). The initial angular momentum Li before the collision is given by:
Li=Iω0
Where I is the moment of inertia of the wheel and ω0 is the initial angular velocity.
The final angular momentum Lf after the impulse is given by:
Lf=Iω+mvr
Where v is the linear velocity of the center of mass after the impulse, and r is the radius of the wheel.
Hint: Remember that for pure rolling, the relationship between linear velocity v and angular velocity ω is v=ωr.
Step 4: Relate Linear and Angular Velocities
Since the wheel rolls without slipping, we have:
v=ωr
Substituting this into the equation for angular momentum gives:
Lf=Iω+m(ωr)r=Iω+mωr2
Hint: Substitute the expression for v into the angular momentum equation.
Step 5: Moment of Inertia of the Wheel
For a solid disk or wheel, the moment of inertia I is given by:
I=12mr2
Substituting this into the angular momentum equations gives:
Li=12mr2ω0
Lf=12mr2ω+mωr2=12mr2ω+mωr2
Step 6: Set Initial and Final Angular Momentum Equal
Setting Li=Lf:
12mr2ω0=12mr2ω+mωr2
Step 7: Simplify the Equation
Canceling mr2 from both sides gives:
12ω0=12ω+ω
This simplifies to:
12ω0=32ω
Step 8: Solve for Initial Angular Velocity
Rearranging gives:
ω=ω03
Thus, the initial angular velocity as the wheel tends to roll up the wall is:
ω=ω03
Final Answer: The initial angular velocity as it tends to roll up the wall is ω03.
The wheel is rolling towards a vertical wall with an angular velocity ω. When it strikes the wall, it experiences an impulse that causes it to roll up the wall. We need to find the initial angular velocity as it tends to roll up.
When the wheel strikes the wall, we can denote:
- C1: the center of the wheel
- C2: the point of contact with the wall
- Gravitational force mg acting downwards.
- Normal force N acting horizontally from the wall.
We will apply the conservation of angular momentum about point C2 (the point of contact with the wall). The initial angular momentum Li before the collision is given by:
Since the wheel rolls without slipping, we have:
For a solid disk or wheel, the moment of inertia I is given by:
Lf=12mr2ω+mωr2=12mr2ω+mωr2
Setting Li=Lf:
Canceling mr2 from both sides gives:
Rearranging gives:
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective
19 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Single Correct
142 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.3
21 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
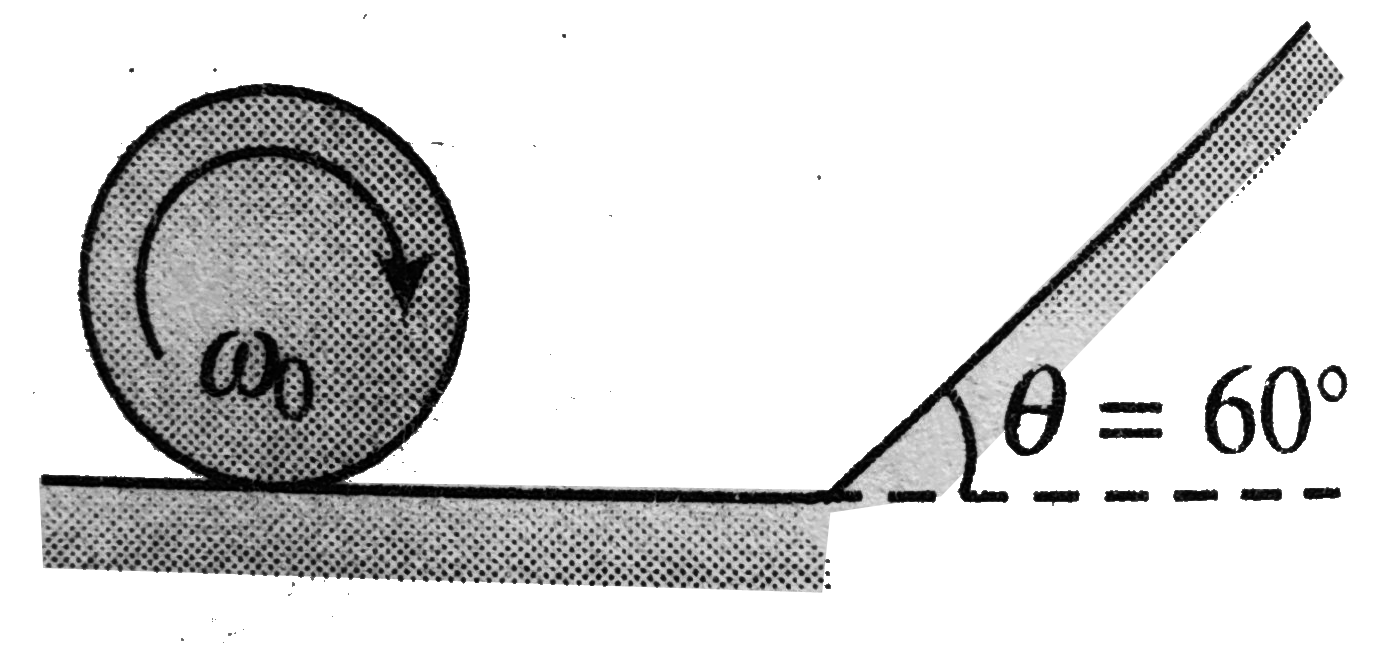
A uniform solid sphere of radius R, rolling without sliding on a horizontal surface with an angular velocity ω0, meets a rough inclined plane of inclination θ=60∘. The sphere starts pure rolling up the plane with an angular velocity ω Find the value of ω.
View SolutionA sphere rolls on a horizontal surface. Is there any point of the sphere which has a vertical velocity?
View Solution
The assembly of two discs as shown in figure is placed on a rough horizontal surface and the front disc is given an initial angular velocity ω0. Determine the final linear and angular velocity when both the discs start rolling. it is given that friction is sufficient to sustain rolling the rear wheel from the starting of motion.
View SolutionA solid sphere rolling on a rough horizontl surface with a lilner speed v collides elastically with a fixed, smooth, vertical wall. Find the speed of the sphere after it has started pure rolling in the backward direction.
View SolutionA uniform sphere of radius R is palced on a rough horizontal surface and given a linear velocity v0 and an angular velocity ω0 as shown . If the angular velocity and the linear velocity both become zero simultaneously, then
View SolutionA disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.

View Solution
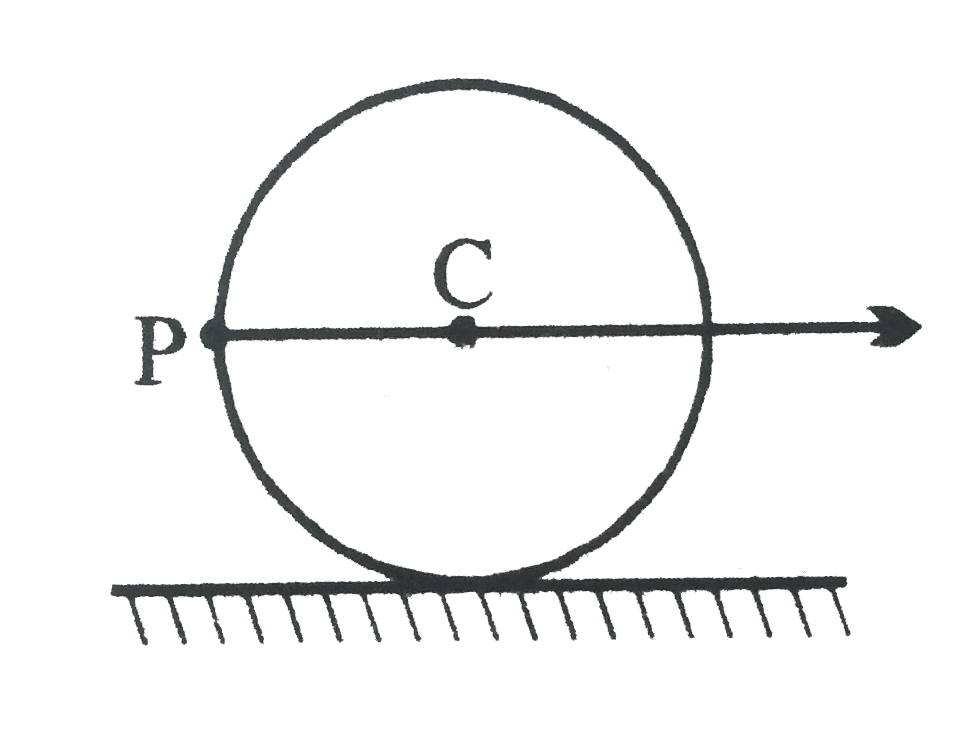
A disc of radius R is rolling purely on a flat horizontal surface, with a constant angular velocity the angle between the velocity ad acceleration vectors of point P is
View SolutionA disc of radius R is rolling purely on a flat horizontal surface, with a constant angular velocity. The angle between the velocity ad acceleration vectors of point P is

View SolutionA disc of radius R is rolling purely on a flat horizontal surface, with a constant angular velocity. The angle between the velocity and acceleration vectors of point P is
 .
.
View SolutionA solid sphere is rolling on a rough surface, whose centre of mass is at C at a certain instant. Find at that instant it has angular velocity ω. Its radius is R. Find the angular acceleration at that instant mass of sphere is m

View Solution
