A solid sphere rolls on a smooth horizontal surface at 10m/s and then rolls up a smooth inclined plane of inclination 30∘ with horizontal. The mass of the sphere is 2kg. Find the height attained by the sphere before it stops (in m).
The correct Answer is:7
To solve the problem of finding the height attained by a solid sphere rolling up an inclined plane, we can use the work-energy theorem. Here’s a step-by-step solution:
Step 1: Understand the Kinetic Energy of the Sphere
The total kinetic energy (KE) of the sphere consists of two parts: translational kinetic energy and rotational kinetic energy.
- Translational Kinetic Energy (TKE):
TKE=12mv2
- Rotational Kinetic Energy (RKE):
RKE=12Iω2
Where:
- m is the mass of the sphere (2 kg),
- v is the linear velocity (10 m/s),
- I is the moment of inertia of the sphere, and
- ω is the angular velocity.
Step 2: Calculate the Moment of Inertia
For a solid sphere, the moment of inertia I is given by:
I=25mr2
However, we will use the relationship between linear velocity and angular velocity:
ω=vr
Step 3: Substitute ω into the RKE Formula
Substituting ω into the RKE formula gives:
RKE=12I(vr)2=12(25mr2)(v2r2)=15mv2
Step 4: Total Kinetic Energy
Now, we can write the total kinetic energy:
Total KE=TKE+RKE=12mv2+15mv2
To combine these, we need a common denominator:
Total KE=(510+210)mv2=710mv2
Step 5: Apply the Work-Energy Theorem
According to the work-energy theorem, the total kinetic energy will be converted into potential energy (PE) when the sphere reaches its maximum height:
Total KE=mgh
Where h is the height attained, and g is the acceleration due to gravity (approximately 9.81m/s2).
Step 6: Set Up the Equation
Now, we can set up the equation:
710mv2=mgh
Step 7: Cancel Mass and Solve for Height
Since the mass m appears on both sides, we can cancel it out:
710v2=gh
Now, substituting v=10m/s and g=9.81m/s2:
710(10)2=9.81h
710×100=9.81h
70=9.81h
Now, solve for h:
h=709.81≈7.13m
Final Answer
The height attained by the sphere before it stops is approximately 7.13m.
---
The total kinetic energy (KE) of the sphere consists of two parts: translational kinetic energy and rotational kinetic energy.
TKE=12mv2
- Rotational Kinetic Energy (RKE):
RKE=12Iω2
Where:
- m is the mass of the sphere (2 kg),
- v is the linear velocity (10 m/s),
- I is the moment of inertia of the sphere, and
- ω is the angular velocity.
For a solid sphere, the moment of inertia I is given by:
I=25mr2
However, we will use the relationship between linear velocity and angular velocity:
ω=vr
Substituting ω into the RKE formula gives:
RKE=12I(vr)2=12(25mr2)(v2r2)=15mv2
Now, we can write the total kinetic energy:
Total KE=TKE+RKE=12mv2+15mv2
To combine these, we need a common denominator:
Total KE=(510+210)mv2=710mv2
According to the work-energy theorem, the total kinetic energy will be converted into potential energy (PE) when the sphere reaches its maximum height:
Total KE=mgh
Where h is the height attained, and g is the acceleration due to gravity (approximately 9.81m/s2).
Now, we can set up the equation:
710mv2=mgh
Since the mass m appears on both sides, we can cancel it out:
710v2=gh
Now, substituting v=10m/s and g=9.81m/s2:
710(10)2=9.81h
710×100=9.81h
70=9.81h
Now, solve for h:
h=709.81≈7.13m
The height attained by the sphere before it stops is approximately 7.13m.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Fill In The Blanks
7 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - True/False
4 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A uniform solid sphere rolls on a horizontal surface with a linear speed of 10 m/s. It then rolls up a.plane inclined at 30° to horizontal. What is the height upto wlrich the sphere rises ? (See Fig.) Assume that the surface is frictionless. Also calculate the distance travelled by the Fig. sphere on the inclined plane ?
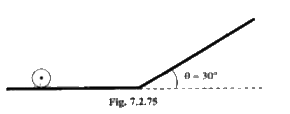
View SolutionA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA hollow sphere and a solid sphere having same mass and same radii are rolled down a rough inclined plane.
View SolutionA sphere is rolling down a plane of inclination θ to the horizontal. The acceleration of its centre down the plane is
View SolutionThe velocity of a sphere rolling down an inclined plane of height h at an inclination θ with the horizontal, will be :
View SolutionA sphere rolls down on an inclined plane of inclination θ. What is the acceleration as the sphere reaches bottom?
View SolutionA sphere rolls down on an inclied plane of inclination θ. What is the acceleration as the sphere reaches bottom ?
View SolutionA solid sphere rolling on a rough horizontal surface. Acceleration of contact point is zero.
A solid sphere can roll on the smooth surface.
View Solution
