The density of water at the surface of the ocean is ρ and atmospheric pressure is P0 If the bulk modulus of water is K, what is the density of ocean water at a depth where the pressure is nP0?
pBB−(α−1)p0
pBB+(α−1)p0
pBB−αp0
pBB+αp0
The correct Answer is:A
From the definition of bulk modulus B=−dpdV/V
As we move from surface to placed where pressure changes to αp0. Let us assume volume changes by △V then.
B=V△p△V=V(α−1)p0△V
New volume V'=V−△V=V[1−(α−)p0B]
Density at the given depth ρ'=ρV/V where ρ is density at surface
ρ'=ρ×BB−(α1)ρ0
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Multiple Correct
17 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Assertion- Reasoning
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Subjective
16 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
Knowledge Check
If the bulk modulus of water is 2100 M Pa, what is the speed of sound in water ?
A1450ms−1B2100ms−1C1400ms−1D1200ms−1The density of water at the surface of ocean is ρ . If the bulk modulus of water is B, then the density of ocean water at depth, when the pressure at a depth is αp0 and p0 is the atmospheric pressure is
AρKk−nP0BρKK+nP0CρKK−(n−1)P0DρK(K+(n−1)P0)The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ
Water is filled up to a height h in a beaker of radius R as shown in the figure. The density of water is ρ, the surface tension of water is T and the atmospheric presure is p0. Consider a vertical section ABCDof the water on one side of this section by water on the other side of this section has magnitude
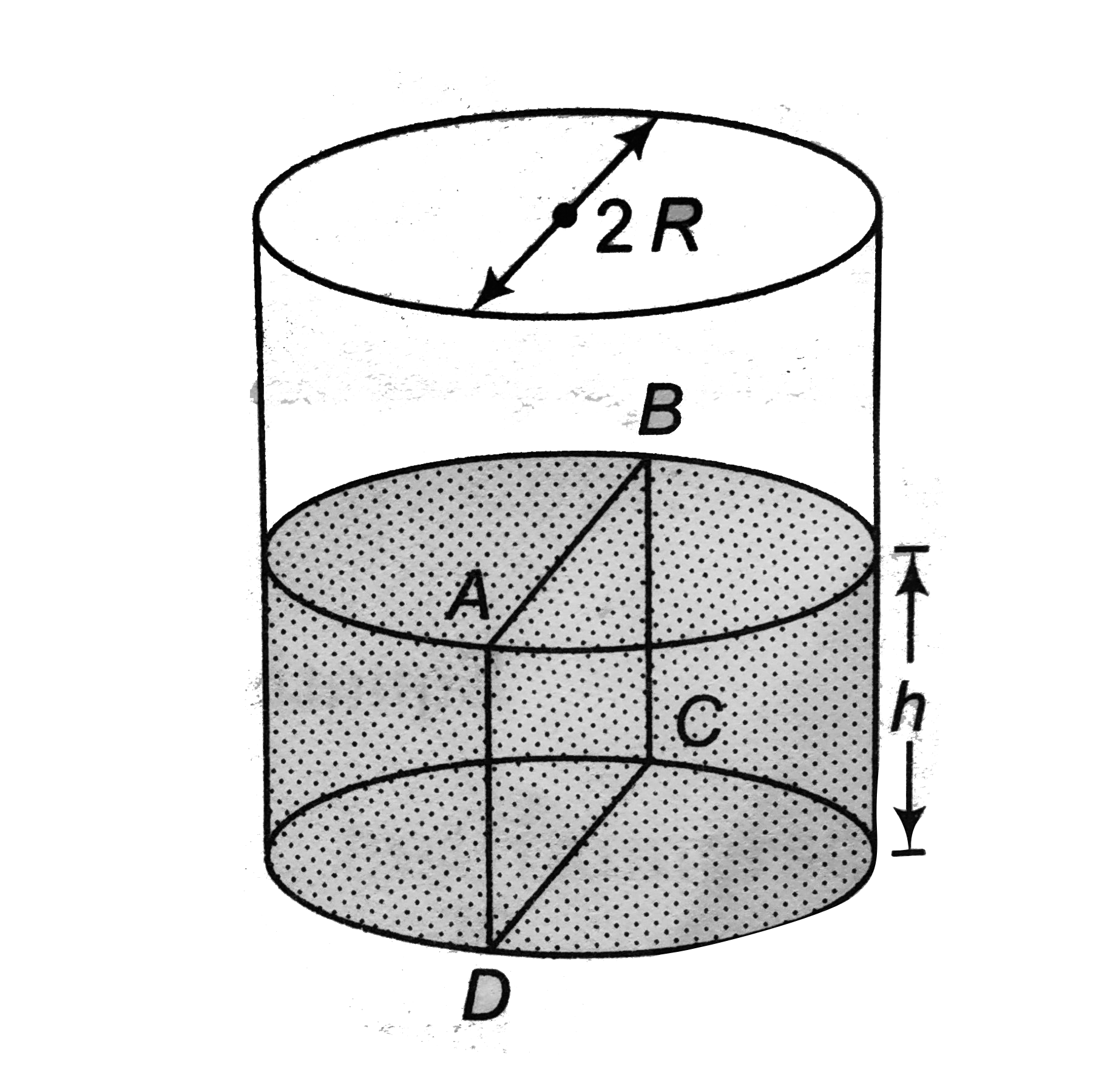
(a) ∣∣2p0Rh+πR2ρgh−2RT∣∣
(b)∣∣2p0Rh+Rρgh2=2RT∣∣
(c ) ∣∣p0(π)R2+Rρgh2−2RT∣∣
(d) ∣∣p0πR2+Rρgh2+2RT∣∣.
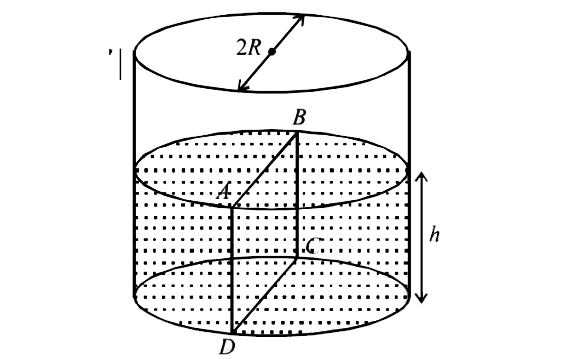
View SolutionWater is filled up to a height h in a beaker of radius R as shown in the figure. The density of water is ρ, the surface tension of water is T and the atmospheric pressure is P0. Consider a vertical section ABCD of the water column through a diameter of the beaker. The force on water on one side of this section by water on the other side of this section has magnitude
View SolutionFor a solution at atmospheric pressure, water potential is
View SolutionIf P is the pressure and ρ is the density of a gas, then P and ρ are related as :
View SolutionThe pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
View Solution
