Statement 1: The value of escape velocity from the surface of earth at 30∘ and 60∘ is v1=2ve,v2=2/3ve.
Statement II: The value of escape velocity is independent of angle of projection.
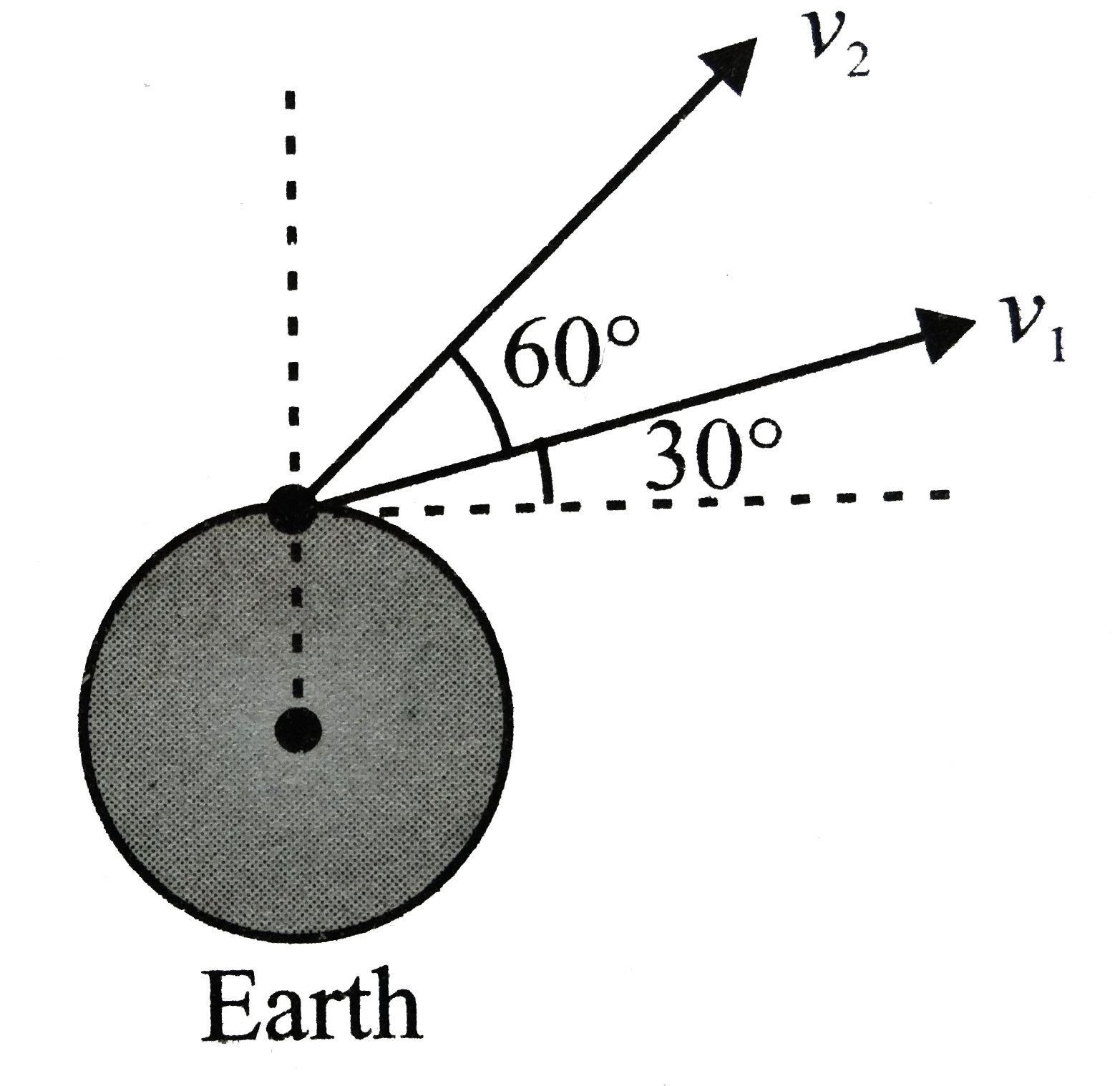
Statement I is True, Statement II is True: Statement II is a correct explanation for Statement I.
Statement I is True, Statement II is True: Statement II is Not a correct explanation for Statement I.
Statement I is True, Statement II is False.
Statement I is False, Statement II is True.
The correct Answer is:D
The value of escape velocity is derived from the method of conservation of total mechanical energy and energy is independnet of direction.
Topper's Solved these Questions
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:Linked Comprehension
Explore 23 Videos
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:Integer
Explore 5 Videos
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:Multiple Correct
Explore 24 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:INTEGER_TYPE
Explore 1 Video
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Integer
Explore 9 Videos
Similar Questions
Statement I: If a particle projected horizontally just above, the surface of the earth with a speed greater than escape speed, then it will escape from gravitational influence of the earth. Assume that particle has a clear path.
Statement II: Escape velocity is independent of its direction.
View SolutionA particle is thrown with escape velocity ve from the surface of earth. Calculate its velocity at height 3 R :-
View Solution
Knowledge Check
The escape velocity from the surface of the earth is (where RE is the radius of the earth )
A√2gREB√gREC2√gRED√3gREThe escape velocity from earth is 11.2 km per sec. If a body is to be projected in a direction making an angle 45∘ to the vertical, then the escape velocity is
A11.2×2 km/secB11.2 km/secC11.2×1√2 km/secD11.2×√2 km/sec
The escape velocity from the surface of the earth is Ve. The escape velcotiy from the surface of a planet whose mass and radius are three times those of the earth, will be
View SolutionSTATEMENT -1 : The escape valocity upon Earth's surface is 11.2 km/s. But velocity required for escaping is little less than 11.2 km/s if launched properly.
and
STATEMENT -2 : Escape velocity is independent of angle of projection. Conservation of mechanical energy uses KE and GPE which are scale quantities.
View SolutionA particle of mass 'm' is projected from the surface of earth with velocityυ=2υe, where υe is the value of escape velocity from the surface of earth . Find velocity of the particle on reaching to interstellar space (at infinity) in terms of υe.
View SolutionStatement-1: Escape velocity is independent of the angle of projection.
Statement-2: Escape velocity from the surface of earth is √2gR where R is radius of earth.
View SolutionA particle is given a velocity ve√3 in a vertically upward direction from the surface of the earth, where ve is the escape velocity from the surface of the earth. Let the radius of the earth be R. The height of the particle above the surface of the earth at the instant it comes to rest is :
View SolutionA mass is taken to a height R from the surface of earth and then is given horizontal velocity v. The minimum value of v so that mass escapes to infinity is √GMnR. Find the value of n.
View Solution
