A particle is projected with velocity 30∘ above on an inclined plane, inclination of which from horizontal is 37∘. Choose the appropriate path (air resistance is negligible)




The correct Answer is:D
To solve the problem, we need to analyze the motion of a particle projected at an angle of 30∘ above an inclined plane that is itself inclined at 37∘ to the horizontal. We will break down the steps to determine the appropriate path of the particle.
1. Identify the Components of Motion:
- The particle is projected with an initial velocity at an angle of 30∘ above the inclined plane.
- The inclined plane is at an angle of 37∘ to the horizontal.
- We need to resolve the gravitational force into two components: one parallel to the incline and one perpendicular to the incline.
2. Resolve the Gravitational Force:
- The gravitational force g can be resolved into two components:
- Along the incline: gsin(37∘)
- Perpendicular to the incline: gcos(37∘)
3. Initial Velocity Components:
- The initial velocity u of the particle can also be resolved into components relative to the inclined plane:
- The component of the initial velocity along the incline (x-direction): ux=ucos(30∘)
- The component of the initial velocity perpendicular to the incline (y-direction): uy=usin(30∘)
4. Analyze the Motion:
- As the particle moves, the vertical component of its velocity vy will decrease due to the gravitational pull acting down the incline.
- At the point of striking the inclined plane, the vertical displacement Sy will be zero, meaning that the final vertical velocity vy will equal the initial vertical velocity uy.
5. Use the Equations of Motion:
- We can apply the second equation of motion to analyze the vertical motion:
Sy=uyt+12(−gcos(37∘))t2
- Setting Sy=0 gives us an equation to solve for time t.
6. Final Velocity Components:
- The horizontal component of velocity vx will decrease due to the acceleration along the incline, which is negative. Thus:
vx=ux−gsin(37∘)t
- Since vx decreases, the angle of the velocity vector at the time of striking the inclined plane will be steeper than the angle of projection.
7. Determine the Path:
- The path of the particle will be parabolic, and due to the decrease in the horizontal component of velocity, the angle at which the particle strikes the inclined plane (let's call it β) will be greater than the angle of projection (α=30∘).
- Therefore, the path will appear steeper as it approaches the inclined plane.
Conclusion:
The appropriate path of the particle, when projected at 30∘ above the inclined plane inclined at 37∘, will be steeper than the angle of projection. Thus, the answer is that the angle β at which it strikes the incline is greater than 30∘.
- The particle is projected with an initial velocity at an angle of 30∘ above the inclined plane.
- The inclined plane is at an angle of 37∘ to the horizontal.
- We need to resolve the gravitational force into two components: one parallel to the incline and one perpendicular to the incline.
- The gravitational force g can be resolved into two components:
- Along the incline: gsin(37∘)
- Perpendicular to the incline: gcos(37∘)
- The initial velocity u of the particle can also be resolved into components relative to the inclined plane:
- The component of the initial velocity along the incline (x-direction): ux=ucos(30∘)
- The component of the initial velocity perpendicular to the incline (y-direction): uy=usin(30∘)
- As the particle moves, the vertical component of its velocity vy will decrease due to the gravitational pull acting down the incline.
- At the point of striking the inclined plane, the vertical displacement Sy will be zero, meaning that the final vertical velocity vy will equal the initial vertical velocity uy.
- We can apply the second equation of motion to analyze the vertical motion:
Sy=uyt+12(−gcos(37∘))t2
- Setting Sy=0 gives us an equation to solve for time t.
- The horizontal component of velocity vx will decrease due to the acceleration along the incline, which is negative. Thus:
vx=ux−gsin(37∘)t
- Since vx decreases, the angle of the velocity vector at the time of striking the inclined plane will be steeper than the angle of projection.
- The path of the particle will be parabolic, and due to the decrease in the horizontal component of velocity, the angle at which the particle strikes the inclined plane (let's call it β) will be greater than the angle of projection (α=30∘).
- Therefore, the path will appear steeper as it approaches the inclined plane.
The appropriate path of the particle, when projected at 30∘ above the inclined plane inclined at 37∘, will be steeper than the angle of projection. Thus, the answer is that the angle β at which it strikes the incline is greater than 30∘.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - MCQ_TYPE20videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - LC_TYPE34videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type3videos
MISCELLANEOUS KINEMATICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
Similar Questions
A particle is projected from the bottom of an inclined plane of inclination 30∘. At what angle α (from the horizontal) should the particle be projected to get the maximum range on the inclined plane.
View SolutionA particle is fired horizontally form an inclined plane of inclination 30∘ with horizontal with speed 50ms−1. If g=10ms−2 , the range measured along the incline is
View SolutionA particle is projected with a certain velocity at an angle ∝ above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally, then ∝ is equal to.
View SolutionA particle is projected with a certain velocity at an angle α above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally then α is equal to
View SolutionA particle is projected from the bottom of an inclined plane of inclination 30∘ with velocity of 40m/s at an angle of 60∘ with horizontal. Find the speed of the particle when its velocity vector is parallel to the plane. Take g=10m/s2.
View SolutionA particle is fired horizontally form an inclined plane of inclination 30 ∘ with horizontal with speed 50 m s − 1 . If g = 10 m s − 2 , the range measured along the incline is
View SolutionThe velocity of a sphere rolling down an inclined plane of height h at an inclination θ with the horizontal, will be :
View SolutionA particles is projected from the foot of an inclined plane having inclination 45∘ , with the velocity u at angle θ(>45∘) with the horizontal in a vertical plane containing the line of greatest slope through the point of projection. Find the value of tanθ if the particle stikes the plane.
(i) Horizontally
(ii) Normally
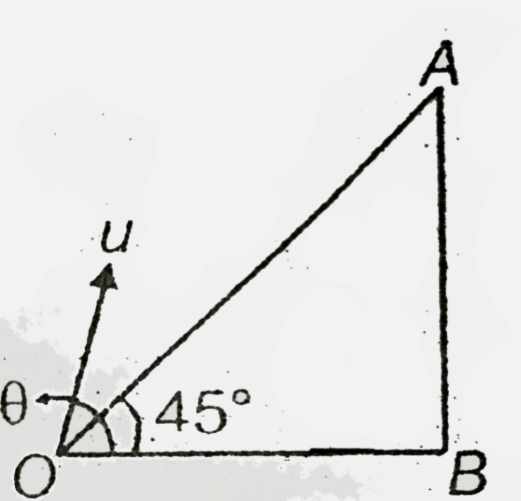 View Solution
View SolutionA particle is projected up an inclined plane of inclination β at an elevation ∝ to the horizontal. Find the ratio between tan∝andtanβ, if the particle strikes the plane horizontally.
View SolutionA particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View Solution

