A uniform nonconducting rod of mass ma and length l, with charge density $λ$ as shown in fig. is hanged at the midpoint at origin so that it can rotate in a horizontal plane without any friction. A uniform electric field E exists parallel to x axis in the entire region. Calculated the period of small oscillation of the rod .

Video Solution

Text Solution

Verified by Experts

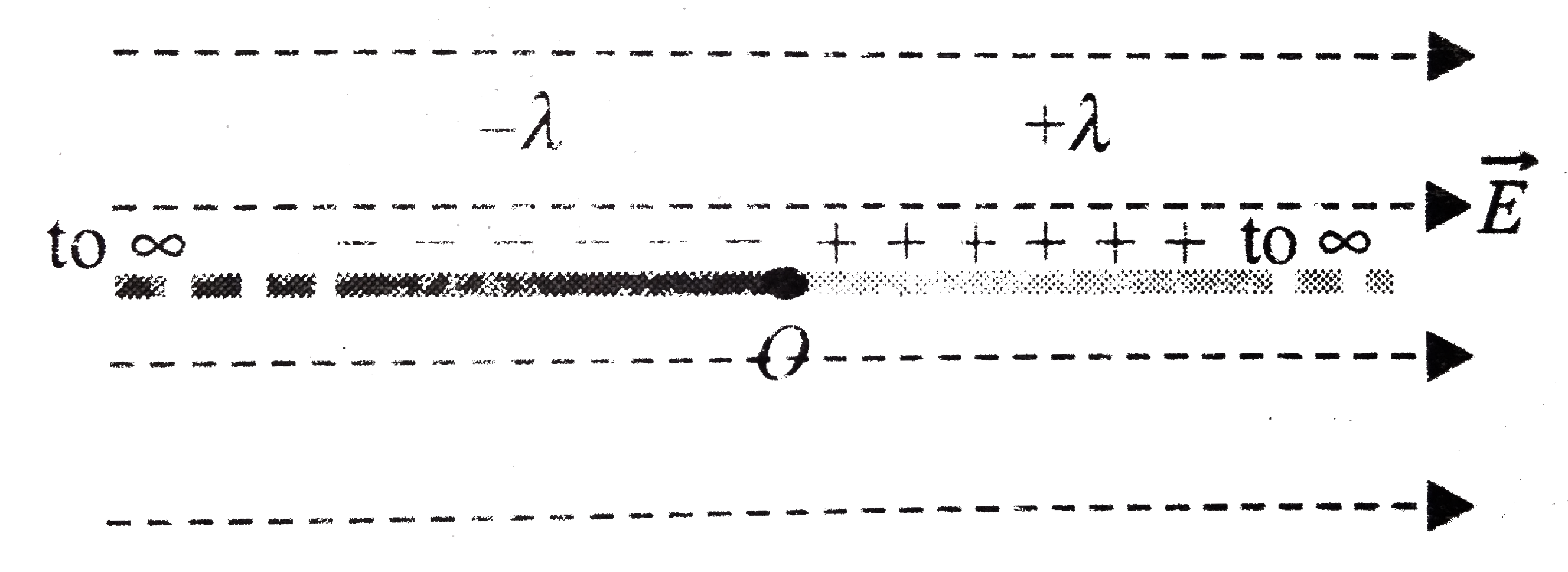
The correct Answer is: $2 π \sqrt{\frac{m}{3 E λ}}$ .

$τ = \int_{0}^{l / 2} d τ_{1} + \int_{0}^{l / 2} d τ_{2}$
$= 2 \int_{0}^{l / 2} E λ d x (x sin θ) = \frac{E λ l^{2}}{4} sin θ$

At $θ$ is small , $τ \approx \frac{E λ l^{2}}{4} θ$
$\frac{m l^{2}}{12} (α) = - \frac{E λ l^{2} θ}{4}$ or $ω = \sqrt{\frac{3 E λ}{m}}$
$∴ T = 2 (π) \sqrt{\frac{m}{3 E λ}}$ .

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
A $\frac{B l^{2} ω}{2}$
Bzero
C $(\frac{B l^{2} ω}{8})$
D $2 B l^{2} ω$

CENGAGE PHYSICS ENGLISH-COULOMB LAW AND ELECTRIC FIELD-Subjective

Home
Ask Doubt
Profile