An uncharged conducting large plate is placed as shown. Now an electric field E towards right is applied. Find the induced charge density on the right surface of the plate.

−ε0E
ε0E
−2ε0E
2ε0E
The correct Answer is:B
b. Net electric field at point P should be zero. For this electric field due to induced charges is equal to the applied electirc field. So,

σε0=Eorσ=ε0E.
Topper's Solved these Questions
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Multiple Correct
8 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Comprehension
36 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Subjective
12 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
15 Videos
Similar Questions
Two conducting plates X and Y, each having large surface aera A (on one side), are placed parallel to each other as show in figure.

Find the surface charge density at the inner surface of the plate X.
View SolutionThree charged conducting plates are separated by small distances as shown in figure. The charges on the plates are shown. Find the ratio of charge on right surface and left surface of the middle plate.

View SolutionTwo large conducting plates X and Y. each having large surface area A (on one side), are placed parallel to each other as shown in figure (30-E7). The plate X is given a charge Q whereas the other is neutral. Find (a) the surface charge density at the inner surface of the plates X, (b) the electric field at a point to the left of the plates, (c) the electric field at a point in between the plates and (d) the electric field at a point to the right of the plates.

View SolutionTwo large plates are given the charges as shown in figure. Now, the left plane is earthed. Find the amount of charge that will flow from the earth to the plate.

View SolutionA positive point charge (+q) is kept in the vicinity of an uncharged conducting plate. Sketch electric field lines originating from the point on to the surface of the plate.
Derive the expression for the electric field at the surface of a charged conductor.
View Solution
A point charge q'q is placed at distance 'a' from the centre of an uncharged thin spherical conducting shell of radius R=2a. A point 'P' is located at a distance 4a from the centre of the conducting shell as shown. The electric potential due to induced charge on the inner surface of the conducting shell at point 'P' is
View SolutionAn electron is projected from a distance d and with initial velocity u parallel to a uniformly charged flat conducting palte as shown. It strikes the plate after travelling a distance l along the direction of projection. The surface charge density of the conducting plate is equal to
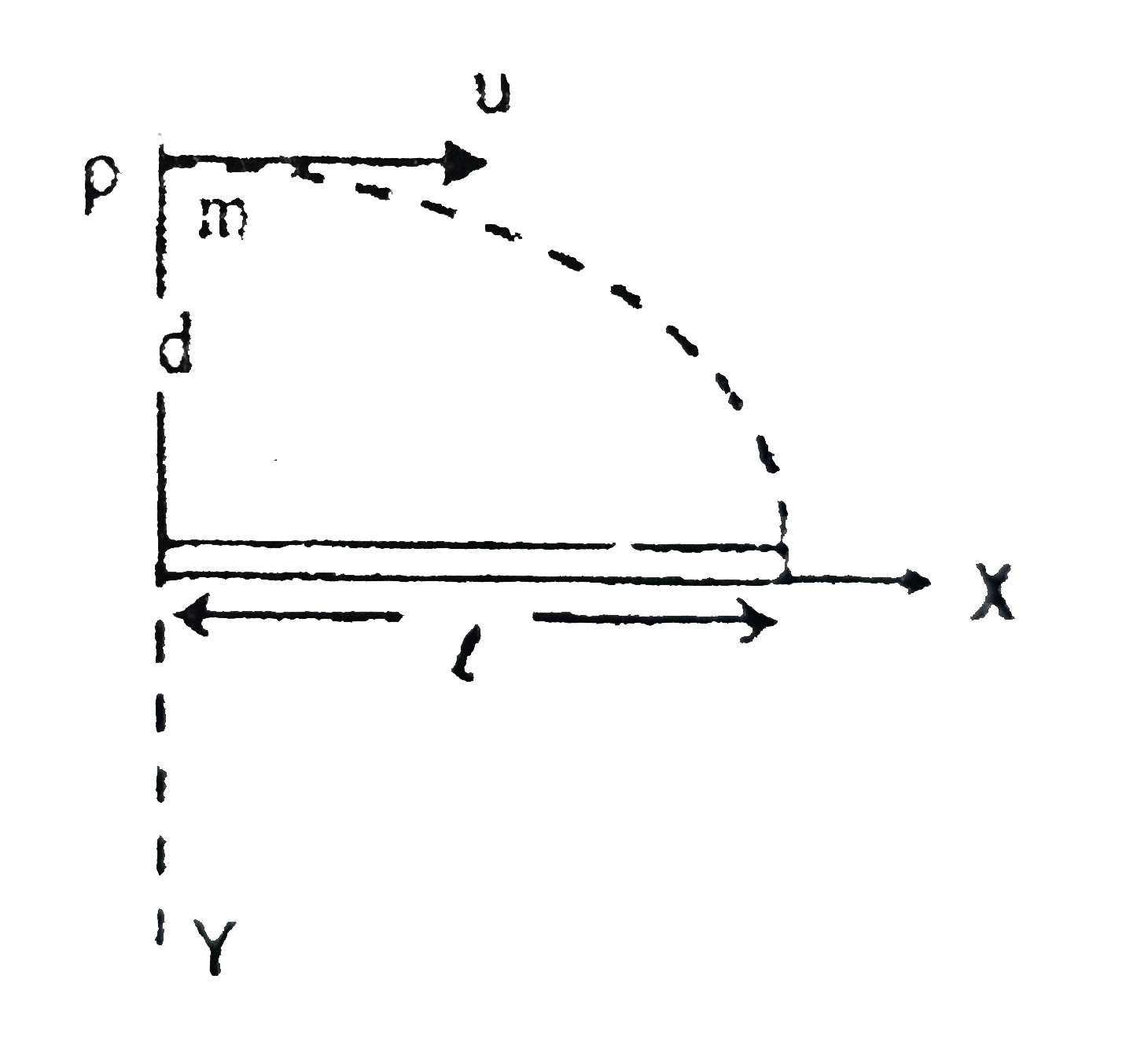
View SolutionTwo large conducting plates are placed parallel to each other with a separation of 2.00 cm betweeen them. An electron starting from rest near one of the plates reaches the other plate in 2.00 microseconds. Find the surface charge density on the inner surfaces.
View SolutionAn isolated conducting sheet of area A and carrying a charge Q is placed in a uniform electric field E, such that the electric field is perpendicular to the sheet and covers all the sheet. Find out the charges appearing on its two surfaces.

View SolutionFour large metallic plates of area A each are kept parallel to each other with a small separation between them as shown in the figure. A cell of emf V is connected across the two outermost plates through a switch K1. The two inner plates are similarly connected with a cell of emf 2 V through a switch k2 Initially both switched are open and the plates, starting form left to right (i.e. number 1 to 4) are given charges Q,2Q,−2Q and −Q respectively. Now answer the following questions

If K1 is closed and K2 is open the charge appearing on the right surface of plate-2 is
View Solution