Three concentric conducting shells of radii a, b and c are shown in (Fig. 3.100). Charge on the shell of radius b is Q. If the key K is closed, find the charges on the innermost and outermost shells and the radio of charge densities of the shells. Given that .
To solve the problem of finding the charges on the innermost and outermost conducting shells and the ratio of charge densities, we can follow these steps:
Step 1: Understand the Configuration
We have three concentric conducting shells with radii a, b, and c such that a:b:c=1:2:3. This means we can express the radii as:
- a=a
- b=2a
- c=3a
Step 2: Analyze the Charge Distribution
When the key K is closed, charge Q is placed on the shell of radius b. According to electrostatic principles, the charge on the inner shell (radius a) must be −Q1 (induced charge) and the outer shell (radius c) must have a charge Q2 such that the net charge in the system remains zero.
Step 3: Apply Gauss's Law
Using Gauss's Law, we can find the electric fields and the potentials at different shells. The electric field outside a charged shell is given by:
E=Qenc4πϵ0r2
where Qenc is the charge enclosed within the Gaussian surface.
Step 4: Set Up the Potential Equations
The potentials at the surfaces of the shells can be expressed as:
- For shell a:
Va=−Q14πϵ0a+Q4πϵ0b+Q24πϵ0c
- For shell b:
Vb=Q4πϵ0b
- For shell c:
Vc=Q24πϵ0c
Step 5: Equate Potentials
Since the shells are conductors, the potential on each shell must be the same when the key is closed:
Va=Vb=Vc
Step 6: Solve for Charges
From the equations derived, we can express the charge relationships:
1. −Q1+Q2+Q23=Q2
2. Q2=Q1+Q
By substituting and simplifying these equations, we can find Q1 and Q2.
Step 7: Calculate Charge Densities
The surface charge density σ on each shell can be calculated using:
σ=QA
where A is the surface area of the shell. The surface area of each shell is:
- For shell a: Aa=4πa2
- For shell b: Ab=4π(2a)2=16πa2
- For shell c: Ac=4π(3a)2=36πa2
Step 8: Find the Ratios of Charge Densities
The charge densities can be expressed as:
- σa=−Q14πa2
- σb=Q16πa2
- σc=Q236πa2
The ratio of charge densities can be calculated as:
σaσb:σbσc:σcσa
Final Step: Conclusion
After calculating the charges and the charge densities, we can summarize the results.
We have three concentric conducting shells with radii a, b, and c such that a:b:c=1:2:3. This means we can express the radii as:
- a=a
- b=2a
- c=3a
When the key K is closed, charge Q is placed on the shell of radius b. According to electrostatic principles, the charge on the inner shell (radius a) must be −Q1 (induced charge) and the outer shell (radius c) must have a charge Q2 such that the net charge in the system remains zero.
Using Gauss's Law, we can find the electric fields and the potentials at different shells. The electric field outside a charged shell is given by:
E=Qenc4πϵ0r2
where Qenc is the charge enclosed within the Gaussian surface.
The potentials at the surfaces of the shells can be expressed as:
- For shell a:
Va=−Q14πϵ0a+Q4πϵ0b+Q24πϵ0c
- For shell b:
Vb=Q4πϵ0b
- For shell c:
Vc=Q24πϵ0c
Since the shells are conductors, the potential on each shell must be the same when the key is closed:
Va=Vb=Vc
From the equations derived, we can express the charge relationships:
1. −Q1+Q2+Q23=Q2
2. Q2=Q1+Q
The surface charge density σ on each shell can be calculated using:
σ=QA
where A is the surface area of the shell. The surface area of each shell is:
- For shell a: Aa=4πa2
- For shell b: Ab=4π(2a)2=16πa2
- For shell c: Ac=4π(3a)2=36πa2
The charge densities can be expressed as:
- σa=−Q14πa2
- σb=Q16πa2
- σc=Q236πa2
σaσb:σbσc:σcσa
After calculating the charges and the charge densities, we can summarize the results.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.123videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.29videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.515videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q2videos
ELECTRICAL MEASURING INSTRUMENTS
Similar Questions
Two concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
View SolutionTwo concentric conducting spherical shells of radii a1anda2(a2>a1) are charged to potentials ϕ1 and ϕ2, respectively. Find the charge on the inner shell.
View SolutionThree concentric conducting shells A,B and C of radii a,b and c are as shown in figure. A dielectric of dielectric constant K is filled between A and B. Find the capacitance between A and C.
 View Solution
View SolutionThere are two thin concentric conducting shells of radii a and b(a<b) . Equal charges Q given to each shell. Find the charge distribution on the inner and outer shell.
View SolutionThree concentric conducting spherical shells carry charges as : +4Q on the inner shell, −2Q on the middle shell and −5Q on the outer shell. The charge on the inner surface of the outer shell is
View SolutionThree concentric conducting sphereical shells carry charges +4Q on the inner shell −2Q on the middle shel and +6Q on the outer shell. The charge on the inner surface of the outer shell is
View SolutionThere are two concentric spherical shell of radii r and 2r. Initially, a charge Q is given to the inner shell and both the switches are open.

If switch S1 is closed and then opened, charge on the outer shell will beView SolutionIn (Fig. 3.73), if the inner shell has charge +q0 and the outer shell is earthed, then
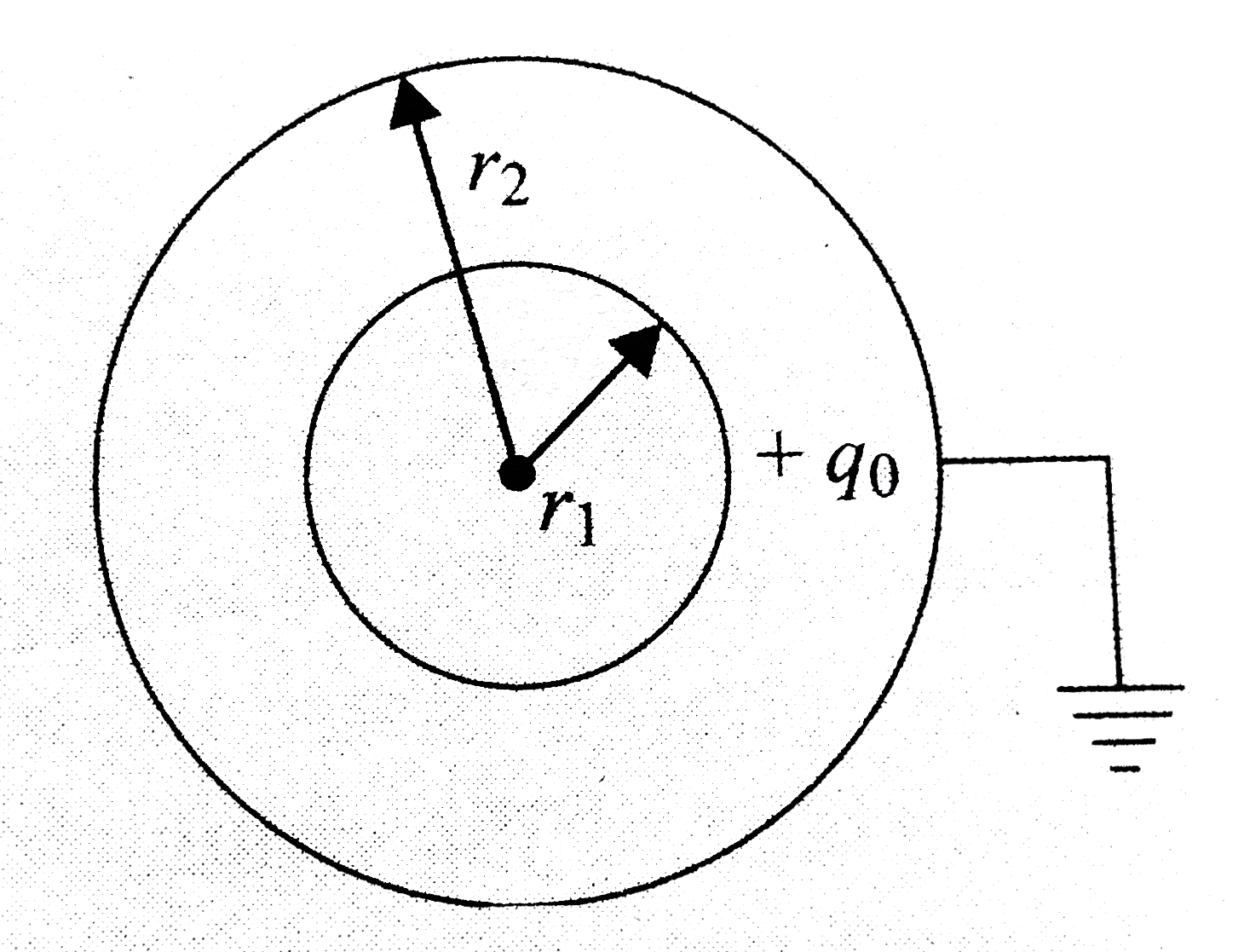 .
.
i. determine the charge on the outer shell, and
ii. find the potential of the inner shell.View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionA common test to find the genotype of a hybrid is by
View Solution

