A capacitor has rectangular plates of length a and width b. The top plate is inclined at a small angle as shown in. The plate separation varies from d=y0 at the left to d=2y0 at the right, where y0 is much less than a or b. system.
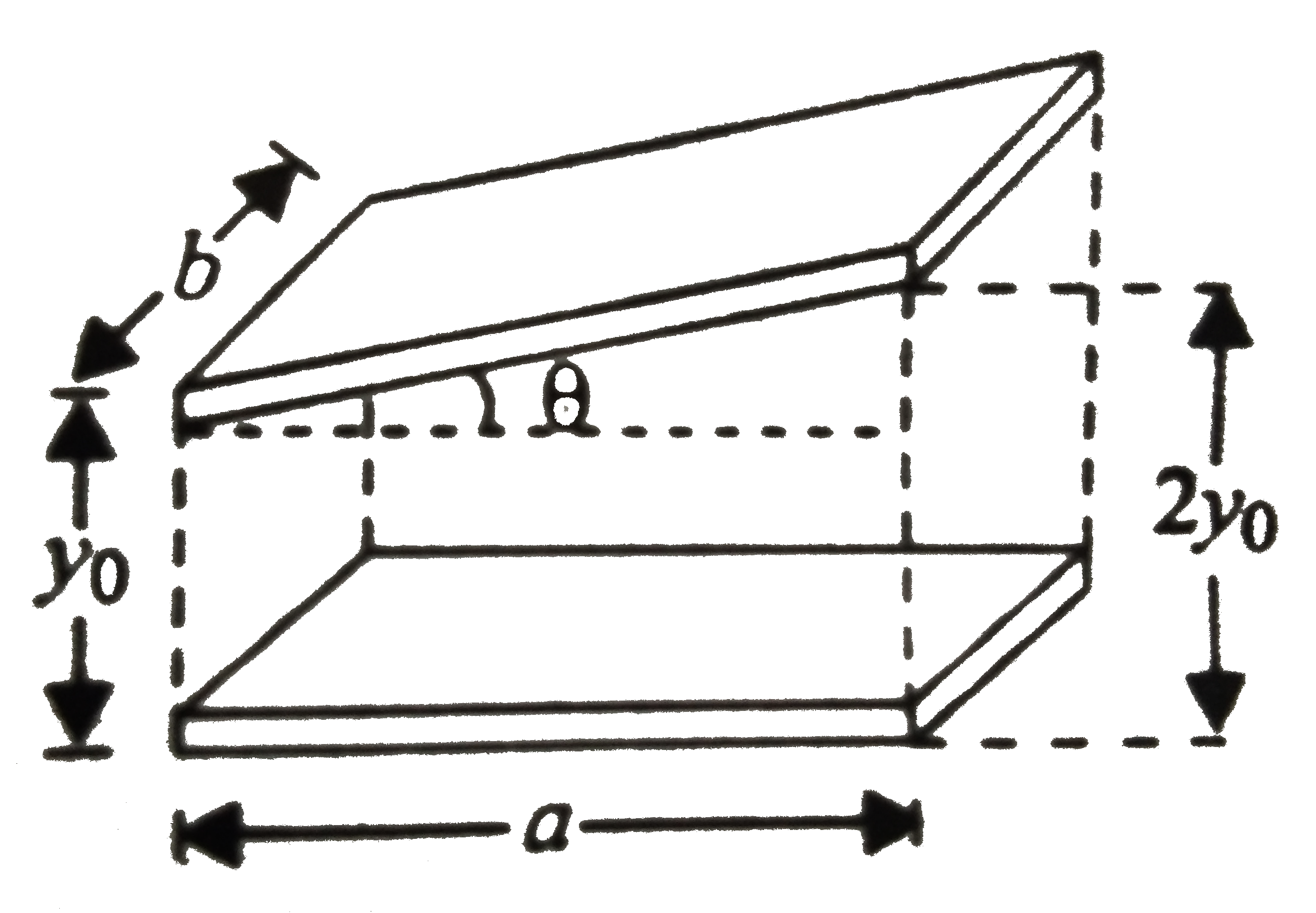 .
.
We consider a differential strip of width dx and length b to approximate a differential capacitor of area bdx and separation
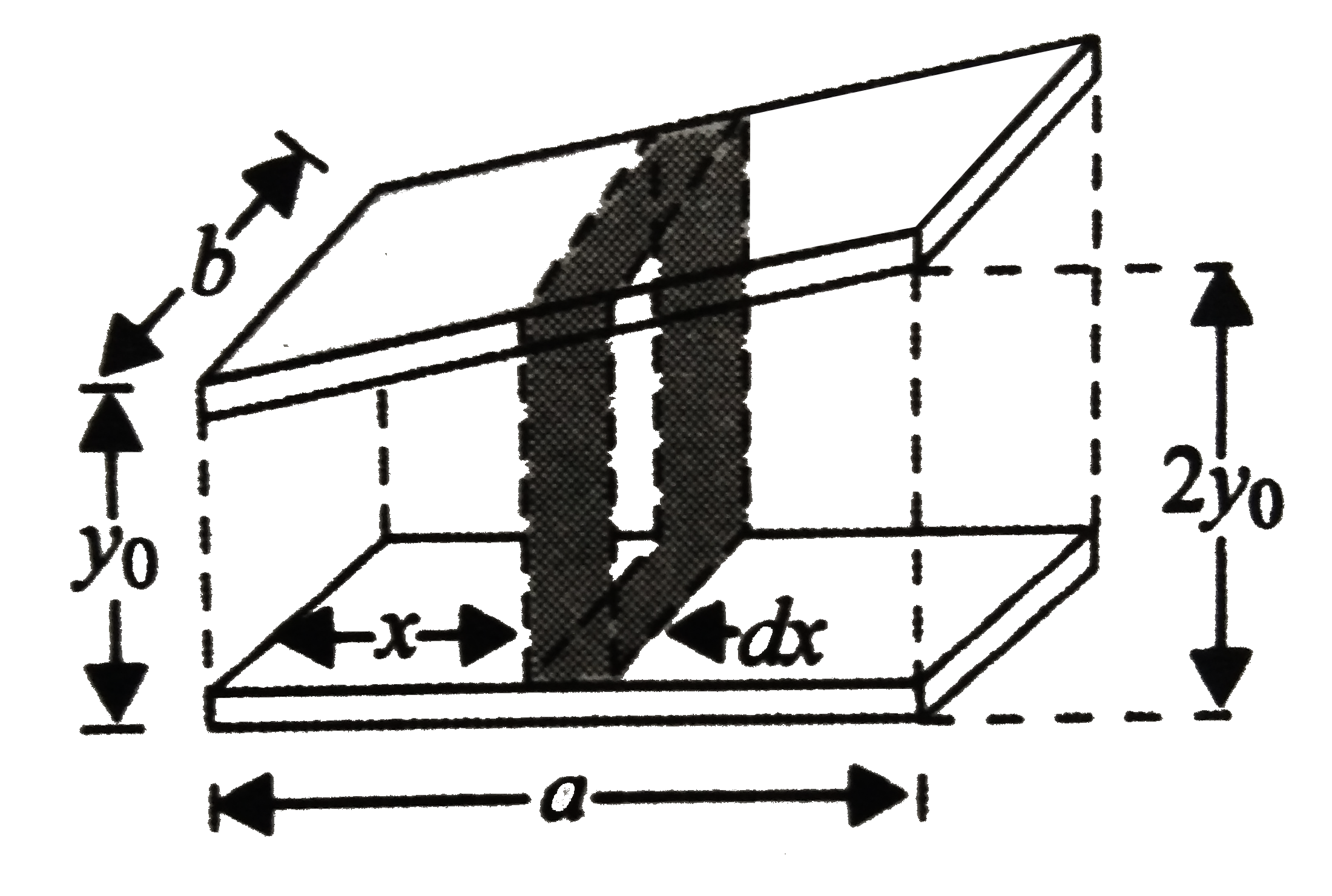
d=y0+(y0a)x.
All such differential capcitors are in parallel arrangement. Thus,
dC=ε0(bdx)y0+(y0a)x or C=∫dC
or C=ε0b∫a0dxy0+y0ax
=ε0b(y⋅0/a)[In(y0+y0a×ay0)]=ε0aby0In2
We can determine the expression for capacity in terms of θ as
d=(y0+xtanθ)
C=∫dC=∫abε0bdxy0+xtanθ
=ε0tanθ In y0+tanθy0
For small θ,
tanθ=θ or C=ε0bθIn(1+aθy0)
Now, we can use the expansion
log(1+x)=x−12x2+...
For x<1, we can negleet higher powers. Thus,
C=ε0bθ[aθy0−12(aθy0)2]=ε0aby0[1−aθ2y0]
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Solved Examples
10 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Exercise 4.1
13 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.3
15 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
Similar Questions
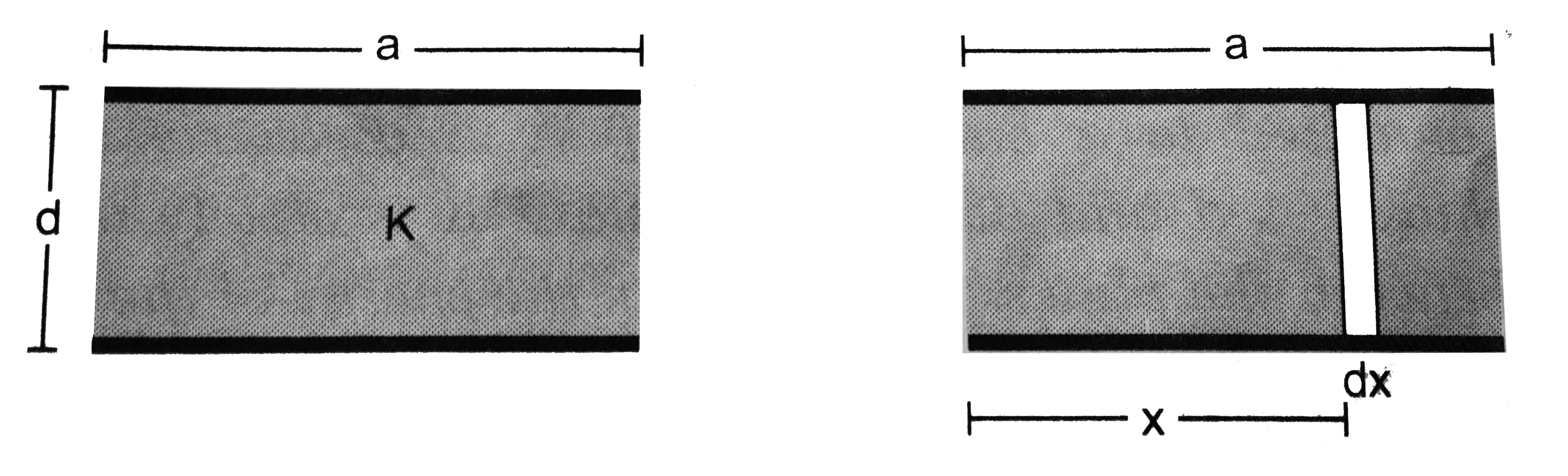
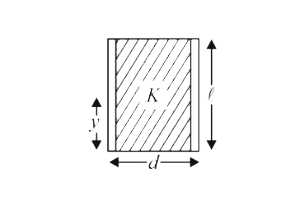
The figure shows a parallel-plate capacitor having square plates of edge a and plate-separation di The gap between the plates is filled with a directric of dielectric constant k which varies as K=K0+αx parallel to an edge as where K and α are constants and x is the distance from the left end. Calaculate the capacitance.

View SolutionA parallel plate capacitor has plate A and separation d between the plates. The capacitor is connected to a battery of emf V. (a) find the charge on the capacitor. (b) the plate separation is decreases to d/2. Find the extra charge given by the battery to the positive plate. (c) work done in reducing separation between plates.
View SolutionFigure shown a parallel-plate capacitor having square plates of edge a and plate-separation d. The gap between the plates is filled with a dielectric of dielectric constant K which varies parallel to an edge as where K and a are constants and x is the distance from the left end. Calculate the capacitance.
View SolutionA capacitance balance is shown in the figure. The balance has a block pulley system attached on one side and a capacitor that has a variable gap width on the other side. Assume upper plate of the capacitor has negligible mass. When the separation between the plate is 1√5 meter, the attractive force between the plates balances the pulley block system. Then find value of V0 in volts (EMF of battery). (Take area of the plate =203ε0m2)

View SolutionA thin rectangular plate with sides of length 'a' and 'b' has mass per unit area varying as α=α0(xyab) where α0 is a constant. The co-ordinated of centre of mass of the plate is
View SolutionA parallel plate capacitor has plates of area A separated by distance ‘d’ between them. It is filled with a dielectric which has dielectric constant that varies as K(y)=k0(1+αy) where ‘y’ is the vertical distance measured from base of the plates. The total capacitance of the system is best given by: (K0 is constant)
View SolutionFour metallic plates each with a surface area of one side A are placed at a distance d from each other as shown. Then, the capacitance of the system between X and Y is

View SolutionA parallel - plate capacitor having plate area 20cm2 and seperation between the plates 1.00mm is connected to a battery of 12.0V. The plates are pulled apart to increase the separation to 2.0mm . (a ) calculate the charge flown through the circuit during the process . (b ) How much energy is absorbed by the battery during the process ? (c ) calculate the stored energy in the electric field before and after the process . (d ) Using the expression for the force between the plates , find the work done by the person polling the plates apart . (e ) Show and justify that no heat is produced during this transfer of charge as the separation is increased.
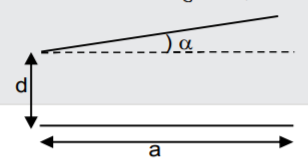
View SolutionTwo square plates of side 'a' are arranged as shown in the figure. The minimum separation between plates is 'd' and one of the plate is inclined at small angle α with plane parallel to another plate. The capacitance of capacitor is (given α is very small)
View SolutionAn air capacitor consists of two parallel plates B as shown in the figure. Plate A is given a change and plate B is given a charge 3Q. P is the median plane of the capacitor. If C0 is the capacitor capacitor, then

View Solution
