Question

Figure shown a parallel-plate capacitor having square plates of edge a and plate-separation d. The gap between the plates is filled with a dielectric of dielectric constant K which varies parallel to an edge as where K and a are constants and x is the distance from the left end. Calculate the capacitance.

Explanation

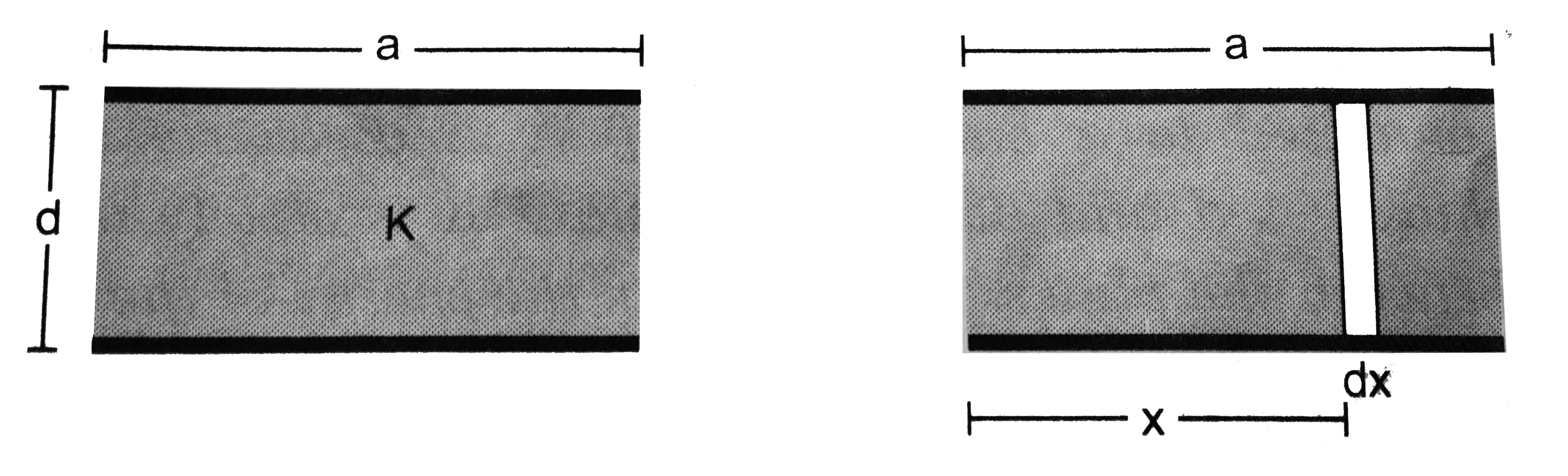
Consider a small strip of width dx at a separation x from the left end. This strip forms a small capacitor of plate area adx. Its capacitance is `
` dC = (K_0 + ax) epsilon_0 adx)/(d)`
` the given capacitor may be divided into such strips with x varying form 0 to a. All these strips are connected in parallel. The capacitance of the given capacitor is, `
` C = int_0 ^a (K_0 + ax) epsilon_0 adx)/(d)`
` `
`= (epsilon_0 a^2)/(d) ((K_0 +(aa)/(2)).