An uncharged parallel plate capacitor having a dielectric of dielectric constant K is connected to a similar air core parallel plate capacitor charged to a potential V0. The two share the charge, and the common potential becomes V. The dielectric constant K is
V0V−1
V0V+1
VV0−1
VV0+1
The correct Answer is:A
To solve the problem, we need to analyze the situation step by step:
Step 1: Understand the initial conditions
We have two parallel plate capacitors:
1. Capacitor 1 (air core) is charged to a potential V0.
2. Capacitor 2 (with dielectric constant K) is initially uncharged.
Step 2: Calculate the initial charge on the air core capacitor
The charge Qi on the air core capacitor can be expressed as:
Qi=CV0
where C is the capacitance of the air core capacitor.
Step 3: Determine the capacitance of the capacitor with dielectric
When the dielectric is inserted into the second capacitor, its capacitance becomes:
C′=KC
where K is the dielectric constant.
Step 4: Apply conservation of charge
When the two capacitors are connected, they will share the charge until they reach a common potential V. According to the conservation of charge:
Qi=Qf
where Qf is the final charge after they share the charge.
Step 5: Express the final charge on both capacitors
The final charge on the capacitor with dielectric is:
Qf=C′V=KCV
The final charge on the air core capacitor is:
Qf=CV
Step 6: Set up the equation using conservation of charge
Equating the initial charge to the final charge gives:
CV0=KCV+CV
Step 7: Factor out C
Since C is common in all terms, we can factor it out:
V0=KV+V
Step 8: Simplify the equation
This can be rewritten as:
V0=(K+1)V
Step 9: Solve for the dielectric constant K
Rearranging the equation gives:
K+1=V0V
Thus,
K=V0V−1
Final Result
The dielectric constant K is:
K=V0V−1
We have two parallel plate capacitors:
1. Capacitor 1 (air core) is charged to a potential V0.
2. Capacitor 2 (with dielectric constant K) is initially uncharged.
The charge Qi on the air core capacitor can be expressed as:
Qi=CV0
where C is the capacitance of the air core capacitor.
When the dielectric is inserted into the second capacitor, its capacitance becomes:
C′=KC
where K is the dielectric constant.
When the two capacitors are connected, they will share the charge until they reach a common potential V. According to the conservation of charge:
Qi=Qf
where Qf is the final charge after they share the charge.
The final charge on the capacitor with dielectric is:
Qf=C′V=KCV
The final charge on the air core capacitor is:
Qf=CV
Equating the initial charge to the final charge gives:
CV0=KCV+CV
Since C is common in all terms, we can factor it out:
V0=KV+V
This can be rewritten as:
V0=(K+1)V
Rearranging the equation gives:
K+1=V0V
Thus,
K=V0V−1
The dielectric constant K is:
K=V0V−1
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Multile Correct11videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Comprhension35videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Subjective24videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.315videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type51videos
CENGAGE PHYSICS DPP
Similar Questions
Parallel Plate Capacitors with and without Dielectric
View SolutionA parallel plate capacitor has a dielectric (of dielectric constant k) between the plates. The plates are charged to a surface charge density σ0. What is the density of the polarized charge that appear on the surface of the dielectric.
View Solution
Knowledge Check
- Question 1 - Select One
A parallel plate air capacitor has a capacitance C. When it is half filled with a dielectric of dielectric constant 5, the percentage increase in the capacitance will be
A400%B66.6%C33.3%D200% - Question 2 - Select One
A parallel plate condenser with a dielectric of dielectric constant K between the plates has a capacity C and is charged to a potential V volt. The dielectric slab is slowly removed from between the plates and then reinserted. The net work done by the system in this process is
AzeroB12(K−1)CV2CCV2(K−1)KD(K−1)CV2
The potential enery of a charged parallel plate capacitor is U0. If a slab of dielectric constant K is inserted between the plates, then the new potential energy will be
View SolutionThe potential enery of a charged parallel plate capacitor is U0. If a slab of dielectric constant K is inserted between the plates, then the new potential energy will be
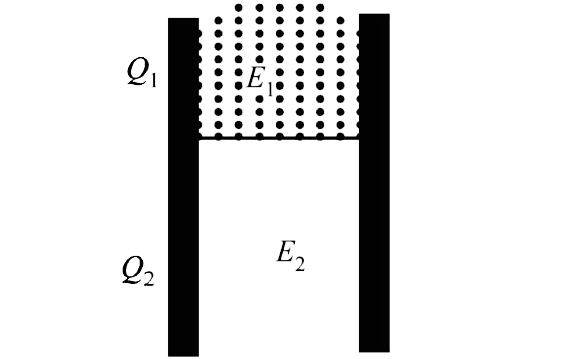
View SolutionA parallel plate capacitor has a dielectric slab of dielectric constant K between its plates that covers 1/3 of the area of its plates, as shown in the figure. The total capacitance of the capacitor is C while that of the portion with dielectric in between is C1. When the capacitor is charged, the plate area covered by the dielectric gets charge Q1 and the rest of the area gets charge Q2. The electric field in the dielectric is E1 and that in the other portion is E2. Choose the correct option/options, ignoring edge effects.
 View Solution
View SolutionIn a parallel-plate capacitor, the region between the plates is filled by a dielectric slab. The capacitor is connected to a cell and the slab is taken out. Then
View SolutionTwo parallel plate capacitors of capacitances C and 2C are connected in parallel and charged to a potential difference V. The battery is then disconnected and the region between the plates of the capacitor C is completely filled with a material of dielectric constant K. The potential differences across the capacitors now becomes...........
View SolutionA parallel plate capacitor has two layers of dielectrics as shown in figure. This capacitor is connected across a battery, then the ratio of potential difference across the dielectric layers is
 View Solution
View Solution

