The current in a simple series cirucit is 5A. When an additional resistance of 2Ω is introduced, the current is reduced to 4A. Calculate the resistance of the original circuit. Assume that the applied potential difference is the same in both the cases.
To solve the problem, we will use Ohm's Law, which states that V=I×R, where V is the voltage, I is the current, and R is the resistance.
1. Identify the Initial Conditions:
- The initial current I1=5A.
- Let the original resistance be R.
- The voltage across the circuit can be expressed as:
V=I1×R=5R
2. Identify the Conditions After Adding Resistance:
- An additional resistance of 2Ω is added.
- The new current I2=4A.
- The total resistance in the circuit now becomes R+2Ω.
- The voltage across the circuit can also be expressed as:
V=I2×(R+2)=4(R+2)
3. Set the Two Voltage Equations Equal:
Since the voltage remains the same in both cases, we can set the two equations equal to each other:
5R=4(R+2)
4. Expand and Simplify the Equation:
Expanding the right side gives:
5R=4R+8
5. Isolate R:
Subtract 4R from both sides:
5R−4R=8
R=8Ω
6. Conclusion:
The original resistance of the circuit is 8Ω.
- The initial current I1=5A.
- Let the original resistance be R.
- The voltage across the circuit can be expressed as:
V=I1×R=5R
- An additional resistance of 2Ω is added.
- The new current I2=4A.
- The total resistance in the circuit now becomes R+2Ω.
- The voltage across the circuit can also be expressed as:
V=I2×(R+2)=4(R+2)
Since the voltage remains the same in both cases, we can set the two equations equal to each other:
5R=4(R+2)
Expanding the right side gives:
5R=4R+8
Subtract 4R from both sides:
5R−4R=8
R=8Ω
The original resistance of the circuit is 8Ω.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Subjective29videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Single Correct72videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Exercise 5.128videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits15videos
ELECTRIC CURRENT & CIRCUITS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
Similar Questions
Find out the value of current through 2Ω resistance for the given circuit.
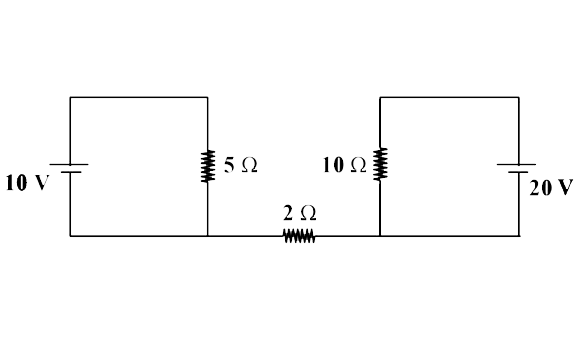 View Solution
View SolutionThe current in a circuit with an external resistance of 3.75Ωis2.5A. When a resistance of 1Ω is introduced into the circuit, the current becomes 0.4A. The emf of the power source is
View Solutionthe current in a circuit containing a battery connected to 2Ω resistance is 0.9A. When ana additional resistance of 8Ω is connected to the same battery the current observed in the circuit is 0.3A. Then the internal resistance of the battery is xΩ. Find value of x.
View SolutionAn electric bulb of resistance 500Ω, draws a current of 0.4 A. Calculate the power of the bulb and the potential difference at its end.
View SolutionIn the given circuit, calculate -
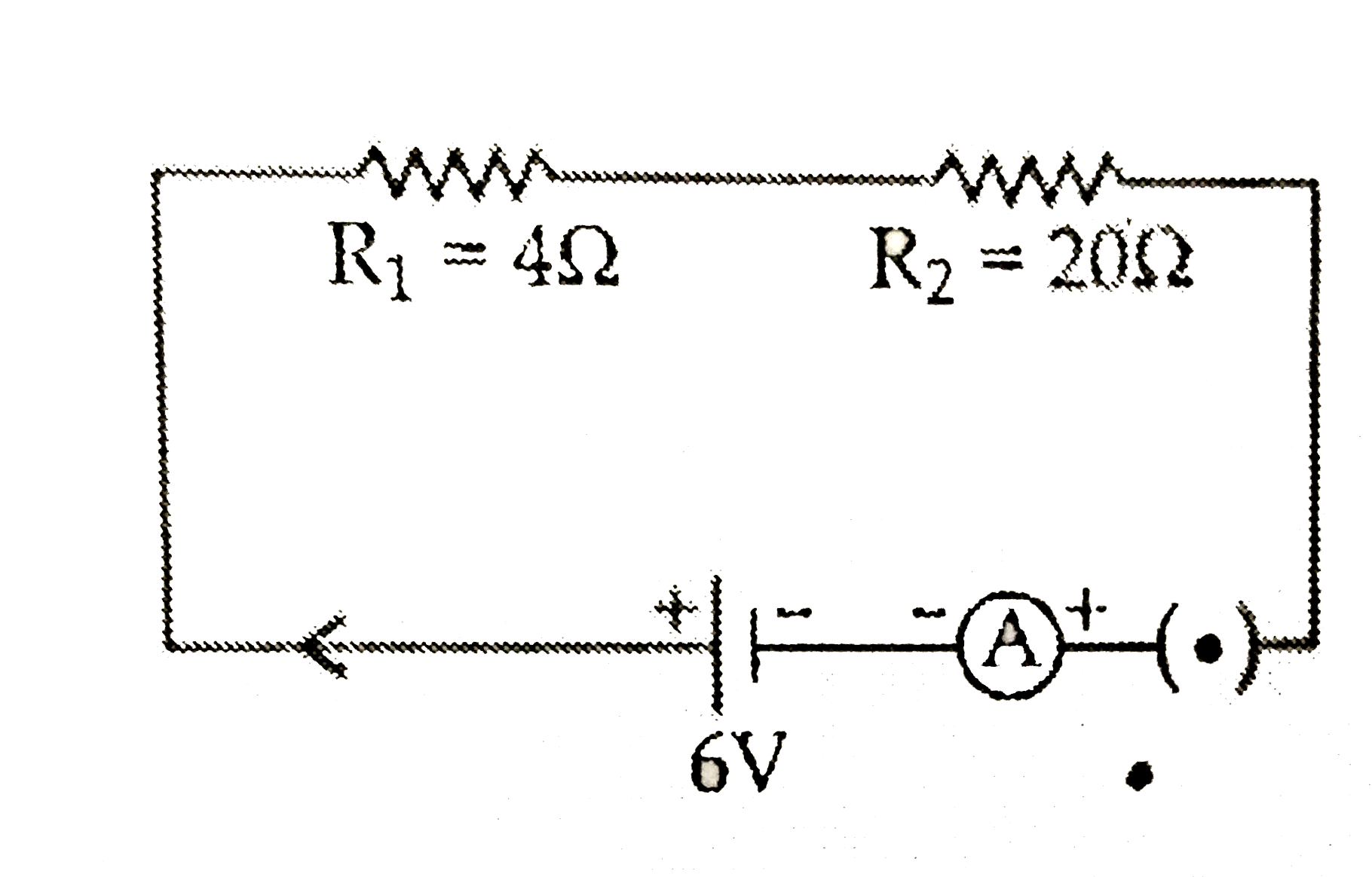
(a) the total resistance of the circuit,
(b) the current through the circuit, and
(c) the potential differences across R1andR2View SolutionA bulb is connected to a battery of p.d. 4 V and internal resistance 2.5 Ω. A steady current of 0.5 A flows through the circuit. Calculate :
the resistance of the bulb,View SolutionA current 0.2A flows in a wire of resistance 15Ω. Find the potential difference across the ends of the wire.
View SolutionTwo batteries of emf 4 V and 8 V with internal resistances 1Ω and 2Ω are connected in a circuit with a resistance of 9Ω as shown in figure. The current and potential difference between the points P and Q are
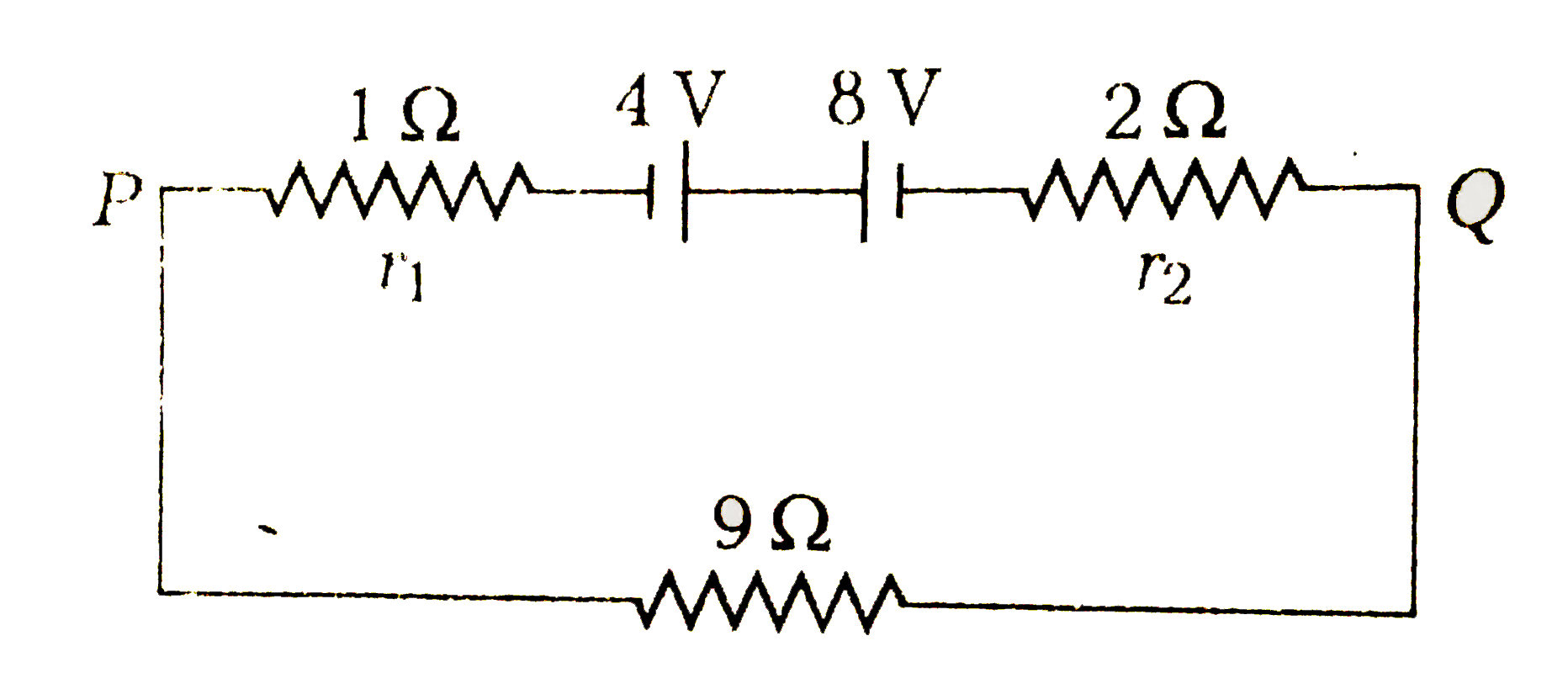 View Solution
View SolutionAn electric lamp of resistance 2Ω and a conductor of resistance 4Ωare connected to a
6V Battery as shown in the circuit. Calculate:
(a) the totak resistance of the circuit,
(b) the current through the circuit,
(c) the potential difference across the (i) electric lamp
and (ii) conductor, and
(d) power of the lamp.
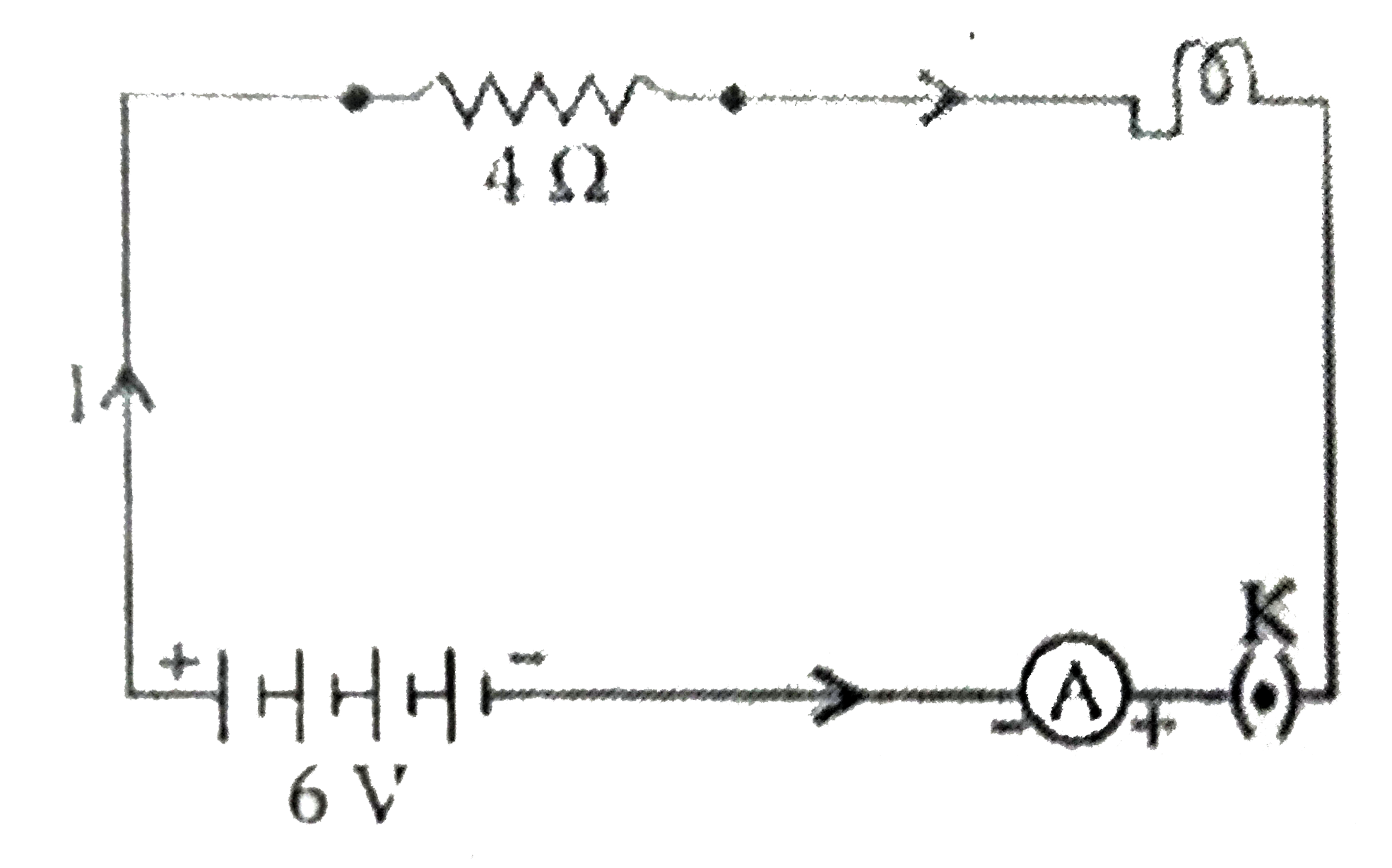 View Solution
View SolutionCalculate the current flows through resistance 2Ω.
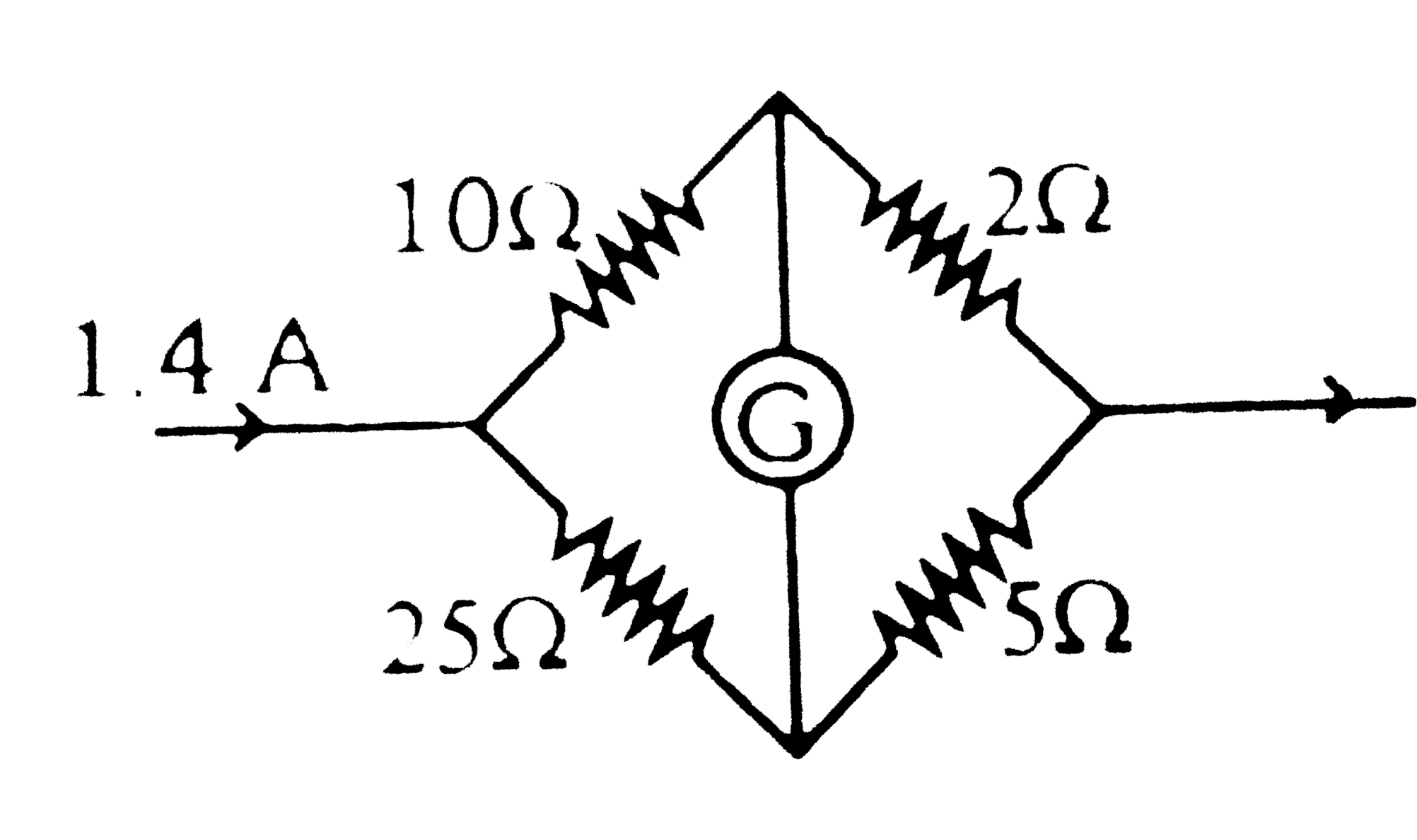 View Solution
View Solution

