An galvanometer deflects full-scale for a potential of . A voltmeter deflcting full-scale of is to be made using this galvanometer. We must connect
a resistance of parallel to the galvanometer
a resistance of in series with the galvanometer
a resistance of parallel to the galvanometer
a resistance of in series with the galvanometer
The correct Answer is:B
To solve the problem of converting an 80-ohm galvanometer that deflects full-scale for a potential of 20 mV into a voltmeter that deflects full-scale at 5 V, we need to determine the required shunt resistance. Here’s a step-by-step solution:
Step 1: Determine the current through the galvanometer at full-scale deflection
The current through the galvanometer (Ig) can be calculated using Ohm's law:
Ig=VgRg
Where:
- Vg=20mV=20×10−3V
- Rg=80Ω
Substituting the values:
Ig=20×10−380=2080×10−3=14×10−3=0.25mA
Step 2: Set up the equation for the voltmeter
When the galvanometer is converted into a voltmeter, we need to find the total resistance (R_total) in the circuit when the voltmeter reads 5 V. The total voltage across the galvanometer and the shunt resistor (S) is equal to the voltmeter reading:
VAB=Ig×(Rg+S)
Where:
- VAB=5V
Step 3: Substitute the known values into the equation
Substituting the known values into the equation:
5=Ig×(Rg+S)
Substituting Ig=0.25×10−3A and Rg=80Ω:
5=0.25×10−3×(80+S)
Step 4: Solve for S
Rearranging the equation gives:
80+S=50.25×10−3
Calculating the right side:
80+S=50.00025=20000
Now, solving for S:
S=20000−80=19920Ω
Step 5: Convert to kilo-ohms
To express S in kilo-ohms:
S=19920Ω=19.92kΩ
Conclusion
The required shunt resistance to convert the galvanometer into a voltmeter that reads 5 V at full-scale deflection is:
S=19.92kΩ
The current through the galvanometer (Ig) can be calculated using Ohm's law:
Ig=VgRg
Where:
- Vg=20mV=20×10−3V
- Rg=80Ω
Ig=20×10−380=2080×10−3=14×10−3=0.25mA
When the galvanometer is converted into a voltmeter, we need to find the total resistance (R_total) in the circuit when the voltmeter reads 5 V. The total voltage across the galvanometer and the shunt resistor (S) is equal to the voltmeter reading:
VAB=Ig×(Rg+S)
Where:
- VAB=5V
Substituting the known values into the equation:
5=Ig×(Rg+S)
Substituting Ig=0.25×10−3A and Rg=80Ω:
5=0.25×10−3×(80+S)
Rearranging the equation gives:
80+S=50.25×10−3
Calculating the right side:
80+S=50.00025=20000
Now, solving for S:
S=20000−80=19920Ω
To express S in kilo-ohms:
S=19920Ω=19.92kΩ
The required shunt resistance to convert the galvanometer into a voltmeter that reads 5 V at full-scale deflection is:
S=19.92kΩ
Topper's Solved these Questions
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Multiple Correct
7 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Assertion-Reasoning
7 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Subjective
18 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - compression type
7 Videos
Similar Questions
A galvanometer of resistance 1000Ω gives full-scale deflection for a current of 1mA. To measure a P.D of 10V, the resistance to be connected with the galvanometer is
View SolutionA galvanometer gives full scale deflection of 1 volt when acting like a volmeter when connected in series with 2kΩ resistance. The same galvanometer gives 500mA, full scale deflection when acting like a ammeter when connected with shunt resistance of value 0.2Ω in parallel. Find out the resistance of galvanometer.
View SolutionA moving coil galvanometer has 50 turns and each turn has an area 210 . The magnetic field produced by the magnet inside the galvanometer is 0.02 . The torsional constant of the suspension wire is 10 . When a current flows through the galvanometer, a full scale deflection occurs if the coil rotates by 0.2 . The resistance of the coil of the galvanometer is 50 . This galvanometer is to be converted into an ammeter capable of measuring current in the range 01.0 . For this purpose, a shunt resistance is to be added in parallel to the galvanometer. The value of this shunt resistance, in , is __________.
View SolutionA galvanometer of resistance 25Ω gives full scale deflection for a current of 10 milliampere , is to be changed into a voltmeter of range 100 V by connecting a resistance of ‘ R ’ in series with galvanometer. The value of resistance R in Ω is
View SolutionA moving coil galvanometer has resistance 50Ω and it indicates full deflection at 4 m A current. A voltmeter is made using this galvanometer and a 5kΩ resistance. The maximum voltmeter, will be close be :
View SolutionWhen a current of 5mA is passed through a galvanometer having a coil of resistance 15Ω, it shows full sacle deflection. The value of the resistance to be put in series with the galvanometer to convert it into a voltmeter of range 0−10V is:
View SolutionWhen 0.005A current flows through a moving coil galvanometer, it gives fullscale deflection. It is converted into a voltmeter to read 5 Volt, using an external resistance of 975Ω. The resistance of galvanometer in ohms is
View Solution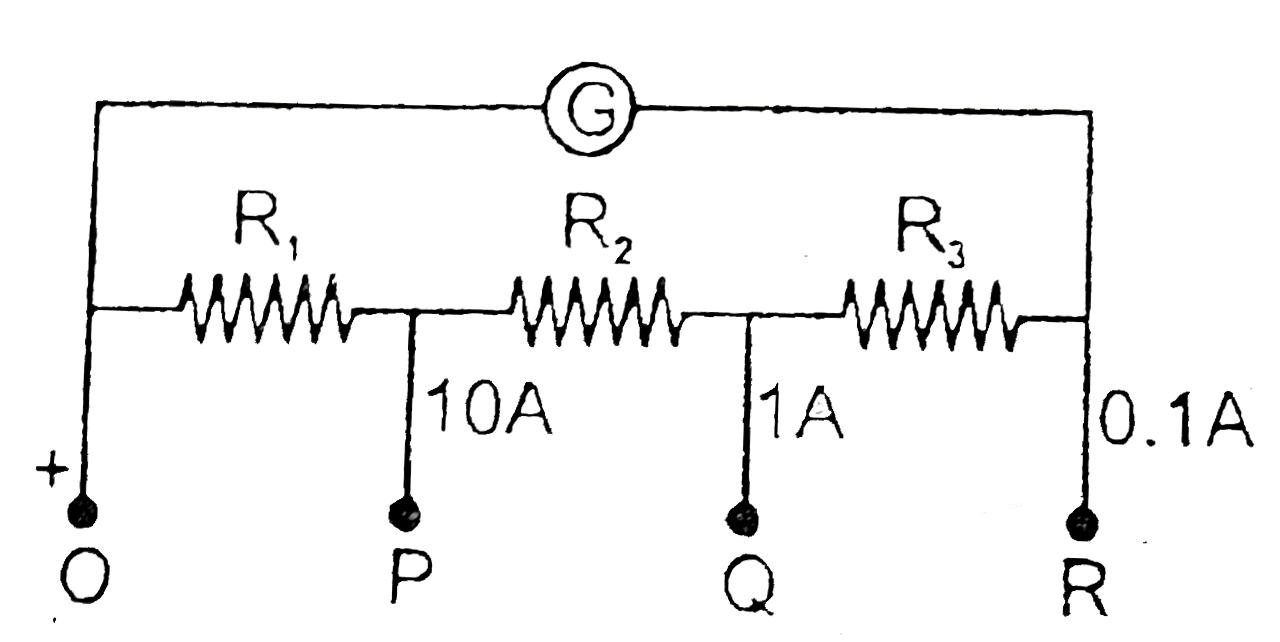
The resistance of the galvanometer G in the circuit's 25Ω. The meter deflects full scale for a current of 10 mA. The meter behaves as an ammeter of three different ranges. The range 0−10 A, if the terminals O and P are taken, range 0−1A between O and Q, range is 0−0.1 A between O and R. Then,
View SolutionA galvanometer coil has a resistance of 50Ω and the meter shows full scale deflection for a current of 5 mA . This galvanometer is converted into a voltmeter of range 0 - 20 V by connecting
View SolutionA galvanometer of resistance 50Ω is connected to a battery of 3V along with resistance of 2950Ω in series. A full scale deflection of 30 divisions is obtained in the galvanometer. In order to reduce this deflection to 20 division the above series resistance should be
View Solution
