A battery is connected to a potentiometer and a balance point is obtained at 84cm along the wire. When its terminals are connected by a 5Ω resistor, the balance point changes to 70cm.
Find the new position of the balance point when 5Ω resistor is changed by 4Ω resistor.
26.5cm
52cm
67.2cm
83.3cm
The correct Answer is:C
To solve the problem step by step, we will use the principles of potentiometers and the relationship between resistance, balance points, and lengths along the wire.
Step 1: Understand the initial conditions
- The initial balance point (L1) when the battery is connected without any load is at 84 cm.
- When a 5 Ω resistor is connected across the battery terminals, the new balance point (L2) is at 70 cm.
Step 2: Use the formula for internal resistance
The internal resistance (R) of the battery can be calculated using the formula:
R=L1−L2L2×R1
Where:
- L1=84cm
- L2=70cm
- R1=5Ω
Step 3: Calculate the internal resistance
Substituting the values into the formula:
R=84−7070×5
R=1470×5=15×5=1Ω
Step 4: Find the new balance point with a 4 Ω resistor
Now, we need to find the new balance point (L3) when the 5 Ω resistor is replaced by a 4 Ω resistor. We will use the same formula:
R=L1−L3L3×R3
Where:
- R3=4Ω
Step 5: Substitute known values into the formula
Using the internal resistance we found (1 Ω):
1=84−L3L3×4
Step 6: Rearranging the equation
Rearranging gives:
L3=84×44+1=3365
Step 7: Calculate L3
Now, calculating L3:
L3=3365=67.2cm
Final Answer
The new position of the balance point when the 5 Ω resistor is replaced by a 4 Ω resistor is 67.2 cm.
---
- The initial balance point (L1) when the battery is connected without any load is at 84 cm.
- When a 5 Ω resistor is connected across the battery terminals, the new balance point (L2) is at 70 cm.
The internal resistance (R) of the battery can be calculated using the formula:
R=L1−L2L2×R1
Where:
- L1=84cm
- L2=70cm
- R1=5Ω
Substituting the values into the formula:
R=84−7070×5
R=1470×5=15×5=1Ω
Now, we need to find the new balance point (L3) when the 5 Ω resistor is replaced by a 4 Ω resistor. We will use the same formula:
R=L1−L3L3×R3
Where:
- R3=4Ω
Using the internal resistance we found (1 Ω):
1=84−L3L3×4
Rearranging gives:
L3=84×44+1=3365
Now, calculating L3:
L3=3365=67.2cm
The new position of the balance point when the 5 Ω resistor is replaced by a 4 Ω resistor is 67.2 cm.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Integer8videos
ELECTRICAL MEASURING INSTRUMENTS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q2videos
ELECTRICAL MEASURING INSTRUMENTS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Assertion-Reasoning7videos
ELECTRICAL MEASURING INSTRUMENTS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.515videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - compression type7videos
ELECTROMAGNETIC INDUCTION
Similar Questions
A battery is connected to a potentiometer and a balance point is obtained at 84cm along the wire. When its terminals are connected by a 5Ω resistor, the balance point changes to 70cm.
Calculate the internal resistance of the cell.View SolutionFor a cell of e.m.f. 2 V , a balance is obtained for 50 cm of the potentiometer wire. If the cell is shunted by a 2Ω resistor and the balance is obtained across 40 cm of the wire, then the internal resistance of the cell is
View SolutionIn a metre bridge, the gaps are closed by two resistance P and Q and the balance point is obtained at 40cm. When Q is shunted by a resistance of 10Ω, the balance point shifts to 50cm. The values of P and Q are.
 .View Solution
.View SolutionA battery of unknown emf connected to a potentiometer has balancing length 560 cm. If a resistor of resistance 10 ohm, is connected in parallel with the cell the balancing length change by 60 cm. If the internal resistance of the cell is n10 ohm, the value of 'n' is
View SolutionIn a potentiometer experiment the balancing length with a cell is 560 cm. When an external resistance of 10Ω is connected in parallel to the cell, the balancing length changes by 60 cm. Find the internal resistance of the cell.
View SolutionWhen the switch is open in lowerpoint loop of a potentiometer, the balance point length is 60cm. When the switch is closed with a known resistance of R=4Ω the balance point length decreases to 40cm. Find the internal resistance of the unknown battery.
View SolutionA potentiometer is connected across Aand B and a balance is obtained at 64.0cm. When the potentiometer lead at B is moved to C, a balance is found at 8.0cm. If the potentiometer is now connected across B and C, a balanced will be found at
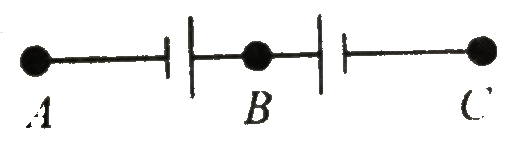 View Solution
View SolutionWhen un known resistance and a resistance of 4Ω are used in the left and right gaps of a meter bridge, the balance point is 50cm. the shift in the balance point if a 4Ω resistance is now connected parallel to the resistor in right gap ______
View Solution
In the figure shown wire AB has a length of 100cm and resistance 8Ω. Find the balance point length l.View SolutionTwo resistances are connected in two gaps of a meter bridge. The balance point is 20cm from the zero end. A resistance of 15Ω is connected in series with the smaller of the two. The null point shifts to 40cm. The value of the smaller resistance in Ω is
View Solution

