A parallel plate capacitor of plate area A and plate separation d is charged to potential difference V and then the battery is disconnected. A slab of dielectric constant K is then inserted between the plates of the capacitor so as to fill the space between the plates. If Q, E and W denote respectively, the magnitude of charge on each plate, the electric field between the plates (after the slab is inserted), and work done on the system, in question, in the process of inserting the slab, then
Q=ε0AVd
Q=ε0KAVd
E=(VKd)
W=ε0AV22d[1−1K]
To solve the problem step by step, we will analyze the situation of a parallel plate capacitor with a dielectric slab inserted after the capacitor has been charged and disconnected from the battery.
Step 1: Calculate the initial charge on the capacitor
The charge Q on the capacitor when connected to a battery with potential difference V is given by:
Q=C⋅V
Where C is the capacitance of the capacitor. The capacitance C for a parallel plate capacitor is given by:
C=εAd
Substituting this into the equation for charge:
Q=εAd⋅V
Step 2: Understand the conservation of charge
Since the battery is disconnected, the charge Q remains constant even after the dielectric slab is inserted. Thus, the charge on the plates after inserting the dielectric remains:
Q=εAVd
Step 3: Calculate the electric field after inserting the dielectric
When the dielectric slab with dielectric constant K is inserted, the capacitance changes to:
C′=K⋅C=K⋅εAd
The new potential difference V′ across the capacitor can be found using the relationship:
Q=C′⋅V′
Substituting for C′:
Q=K⋅εAd⋅V′
Since Q is constant, we can equate:
εAVd=K⋅εAd⋅V′
Cancelling εAd from both sides gives:
V′=VK
The electric field E between the plates is given by:
E=V′d=VK⋅d
Step 4: Calculate the work done in inserting the dielectric
The initial potential energy Ui of the capacitor is:
Ui=12CV2=12⋅εAd⋅V2
The final potential energy Uf after inserting the dielectric is:
Uf=12C′(V′)2=12⋅K⋅εAd⋅(VK)2=12⋅K⋅εAd⋅V2K2=12⋅εAV2dK
Now, the change in potential energy ΔU is:
ΔU=Uf−Ui=(12⋅εAV2dK)−(12⋅εAV2d)
Factoring out common terms:
ΔU=12⋅εAV2d(1K−1)
The work done W on the system is the negative of the change in potential energy:
W=−ΔU=−12⋅εAV2d(1K−1)
Summary of Results
1. Charge Q=εAVd
2. Electric field E=VK⋅d
3. Work done W=12⋅εAV2d(1−1K)
The charge Q on the capacitor when connected to a battery with potential difference V is given by:
Q=C⋅V
Where C is the capacitance of the capacitor. The capacitance C for a parallel plate capacitor is given by:
C=εAd
Substituting this into the equation for charge:
Q=εAd⋅V
Since the battery is disconnected, the charge Q remains constant even after the dielectric slab is inserted. Thus, the charge on the plates after inserting the dielectric remains:
Q=εAVd
When the dielectric slab with dielectric constant K is inserted, the capacitance changes to:
C′=K⋅C=K⋅εAd
The new potential difference V′ across the capacitor can be found using the relationship:
Q=C′⋅V′
Substituting for C′:
Q=K⋅εAd⋅V′
Since Q is constant, we can equate:
εAVd=K⋅εAd⋅V′
Cancelling εAd from both sides gives:
V′=VK
The electric field E between the plates is given by:
E=V′d=VK⋅d
The initial potential energy Ui of the capacitor is:
Ui=12CV2=12⋅εAd⋅V2
The final potential energy Uf after inserting the dielectric is:
Uf=12C′(V′)2=12⋅K⋅εAd⋅(VK)2=12⋅K⋅εAd⋅V2K2=12⋅εAV2dK
Now, the change in potential energy ΔU is:
ΔU=Uf−Ui=(12⋅εAV2dK)−(12⋅εAV2d)
Factoring out common terms:
ΔU=12⋅εAV2d(1K−1)
The work done W on the system is the negative of the change in potential energy:
W=−ΔU=−12⋅εAV2d(1K−1)
1. Charge Q=εAVd
2. Electric field E=VK⋅d
3. Work done W=12⋅εAV2d(1−1K)
Similar Questions
A parallel - plate capacitor of plate area A and plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius r with direction T. On what power of r, will the square of time period depends if the gravitational force of attraction between the planet and the star is proportional to r−5/2 .
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
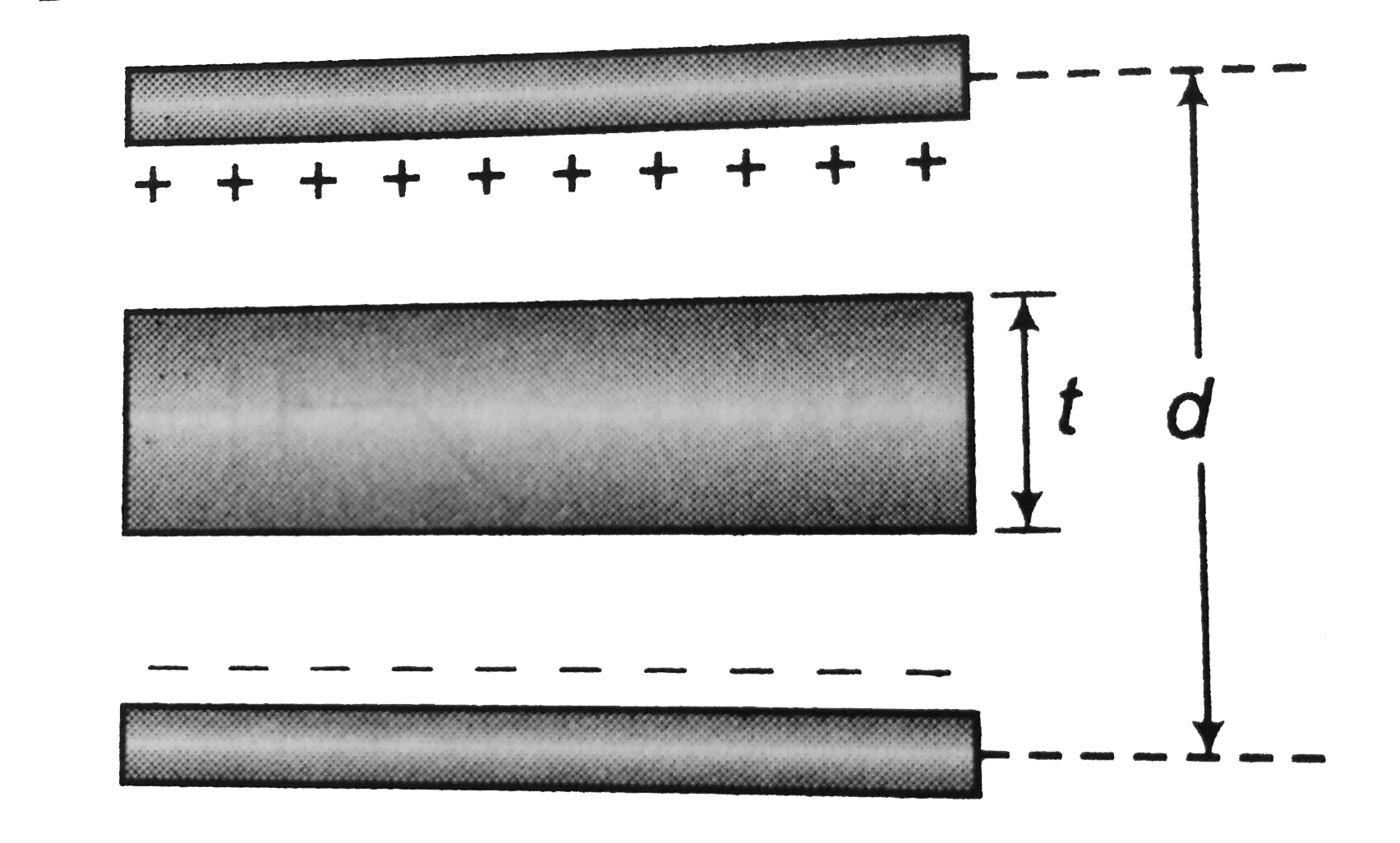
The electric field in the gaps between the plates and the electric slab will be
View SolutionA dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
View SolutionFigue shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
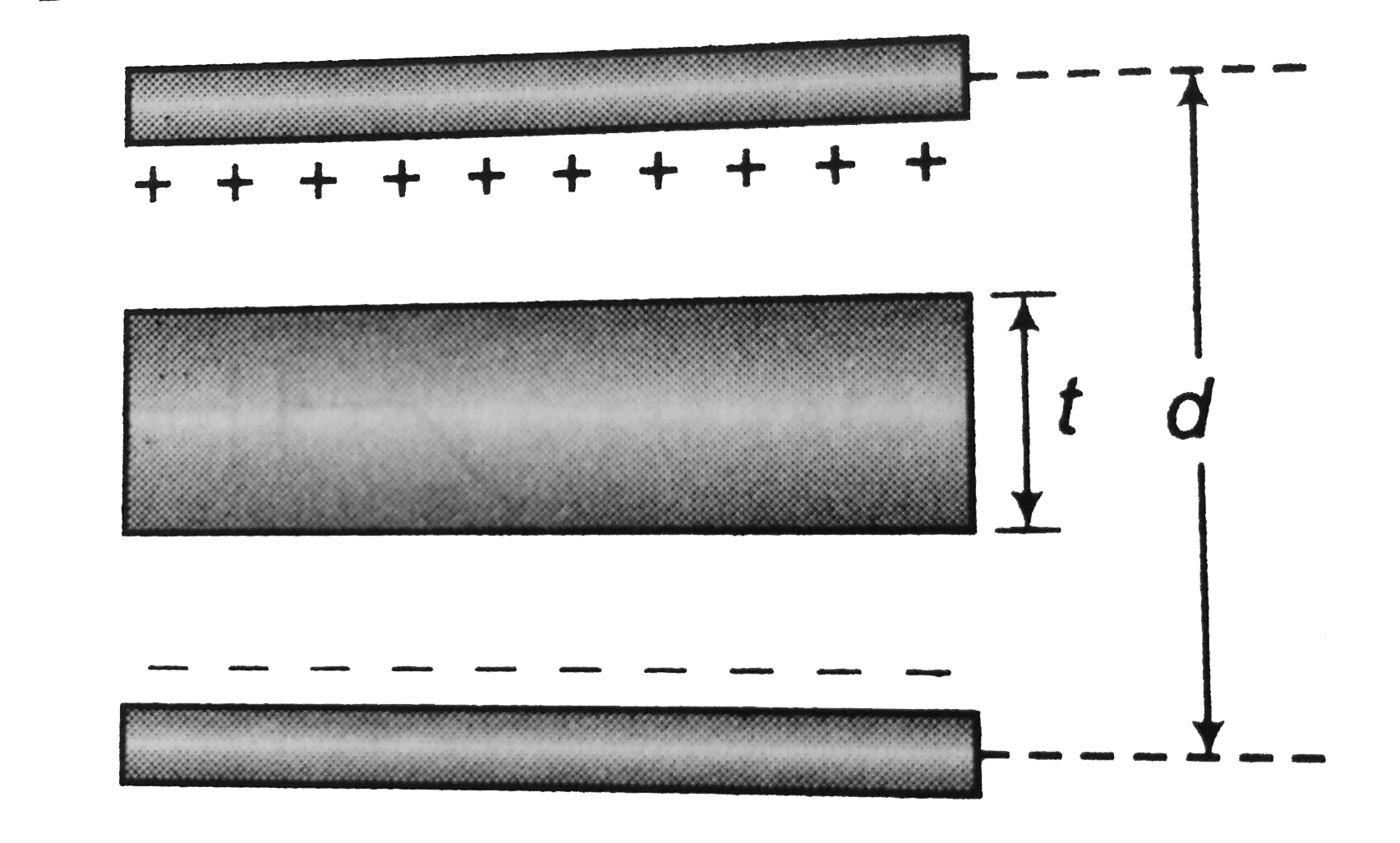
The electric field in the dielectric slab is
View SolutionA parallel plate capacitor of plate area A and plates separation distance d is charged by applying a potential V0 between the plates. The dielectric constant of the medium between the plates is K. What is the uniform electric field E between the plates of the capacitor ?
View SolutionA pararllel plate capacitor has plates of area A and separation d and is charged to potential diference V. The charging battery is then disconnected and the plates are pulle apart until their separation is 2d. What is the work required to separate the plates?
View SolutionA parallel plate capacitor each with plate area A and separation ‘d’ is charged to a potential difference V. The battery used to charge it is then disconnected. A dielectric slab of thickness d and dielectric constant K is now placed between the plates. What change if any, will take place in
(i) charge on the plates
(ii) electric field intensity between the plates,
(iii) capacitance of the capacitor
Justify your answer in each case
View SolutionA parallel plate capacitor, with plate area A and plate separation d, is filled with a dielectric slabe as shown. What is the capacitance of the arrangement ?
View SolutionA parallel plate capacitor has plate area A and plate separation d. The space betwwen the plates is filled up to a thickness x (ltd) with a dielectric constant K. Calculate the capacitance of the system.

View Solution