A convex lens of focal length 10cm is placed 30 cm in front of a second convex lens also of th esame focal length. A plane mirror is placed after the two lenses. Where should a point object be placed in front of the first lens so that it imagees on to itself?
To solve the problem step by step, we need to determine where a point object should be placed in front of the first convex lens so that the image formed by the system (two lenses and a plane mirror) appears at the same location as the object.
Step 1: Understand the System
We have two convex lenses with a focal length f=10cm each, separated by a distance of d=30cm. A plane mirror is placed after the second lens. We need to find the object distance u1 for the first lens such that the final image coincides with the object.
Step 2: Determine the Image Position from the First Lens
The image formed by the first lens will act as the object for the second lens. According to the lens formula:
1f=1v−1u
where:
- f is the focal length of the lens,
- v is the image distance from the lens,
- u is the object distance from the lens.
Step 3: Set Up the Equation for the First Lens
Let v1 be the image distance from the first lens. The focal length f=10cm. Rearranging the lens formula gives:
1u1=1v1−1f
Step 4: Relate the Image Distance of the First Lens to the Second Lens
The distance between the two lenses is 30cm. Therefore, the image distance v1 from the first lens will be related to the object distance u2 for the second lens as follows:
u2=30−v1
The image formed by the first lens must be at the focal point of the second lens for the rays to be parallel after passing through the second lens. Thus, we have:
v2=f=10cm
Step 5: Use the Lens Formula for the Second Lens
Using the lens formula for the second lens:
1f=1v2−1u2
Substituting v2=10cm and u2=30−v1:
110=110−130−v1
Step 6: Solve for v1
Rearranging gives:
130−v1=0⇒30−v1=∞
This implies v1=20cm.
Step 7: Substitute Back to Find u1
Now substituting v1=20cm back into the lens formula for the first lens:
1u1=120−110
Finding a common denominator:
1u1=120−220=−120
Thus, u1=−20cm.
Conclusion
The point object should be placed 20cm in front of the first lens.
We have two convex lenses with a focal length f=10cm each, separated by a distance of d=30cm. A plane mirror is placed after the second lens. We need to find the object distance u1 for the first lens such that the final image coincides with the object.
The image formed by the first lens will act as the object for the second lens. According to the lens formula:
1f=1v−1u
where:
- f is the focal length of the lens,
- v is the image distance from the lens,
- u is the object distance from the lens.
Let v1 be the image distance from the first lens. The focal length f=10cm. Rearranging the lens formula gives:
1u1=1v1−1f
The distance between the two lenses is 30cm. Therefore, the image distance v1 from the first lens will be related to the object distance u2 for the second lens as follows:
u2=30−v1
The image formed by the first lens must be at the focal point of the second lens for the rays to be parallel after passing through the second lens. Thus, we have:
v2=f=10cm
Using the lens formula for the second lens:
1f=1v2−1u2
Substituting v2=10cm and u2=30−v1:
110=110−130−v1
Rearranging gives:
130−v1=0⇒30−v1=∞
This implies v1=20cm.
Now substituting v1=20cm back into the lens formula for the first lens:
1u1=120−110
Finding a common denominator:
1u1=120−220=−120
Thus, u1=−20cm.
The point object should be placed 20cm in front of the first lens.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Subjective
Explore 26 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Single Correct
Explore 221 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Exercise1.6
Explore 15 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSExercise:dpp 3.3
Explore 15 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICS ENGLISHChapter:HEATING EFFECT OF CURRENTExercise:Thermal Power in Resistance Connected in Circuit
Explore 27 Videos
Similar Questions
A convex lens of focal length 20cm is placed 10cm in front of a convex mirror or radius of curvature 15cm. Where should a point object be placed in front of the lens so that it images on to itself?
View SolutionA glass slab of thickness 2cm and refractive index 2 is placed in contact with a biconvex lens of focal length 20cm. The refractive index of the material of the lens 1.5 . The far side of the lens is silvered. Where should an object be placed in front of the slab so that it images on to itself?
View Solution
Knowledge Check
A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens. The image is
Areal, at 30 cm in front of the mirrorBreal, at 30 cm behind the mirrorCreal, at 10 cm in front of the mirrorDvirtual, at 10 cm behind the mirror
A convex lens of focal length 40 cm is in contact with a concave lens of focal length 25 cm. The power of the combination is
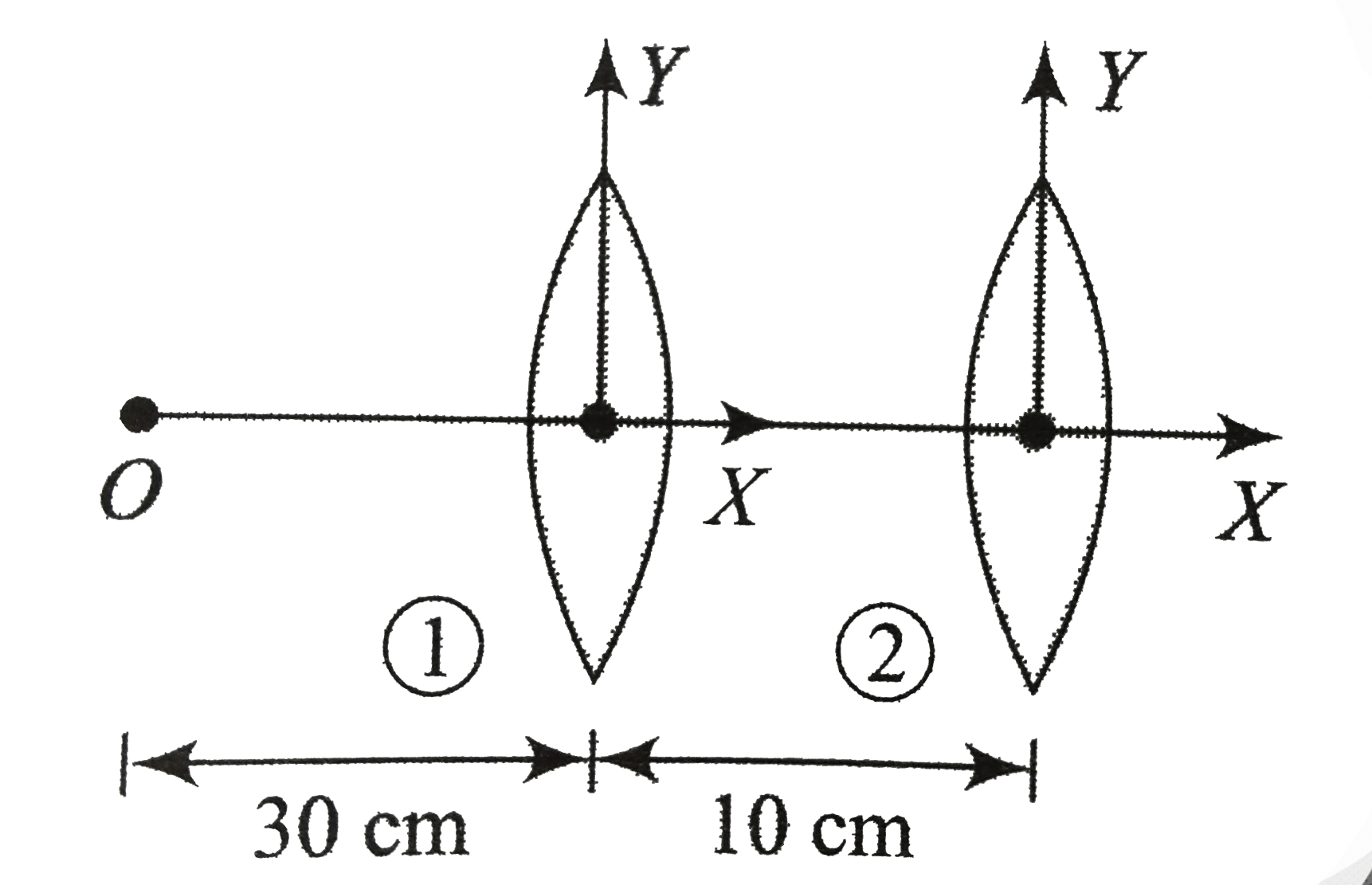
View SolutionA convex lens of focal length 20cm is placed 10cm away from a second convex lens of focal length 25 cm. What wil be the location of the image of an object at 30 cm in front of the first lens?
View SolutionAn optical system consists of a thin convex lens of focal length 30cm and a plane mirror placed 15cm behind the lens. An object is placed 15cm in front of the lens. The distance of the final image from the object is
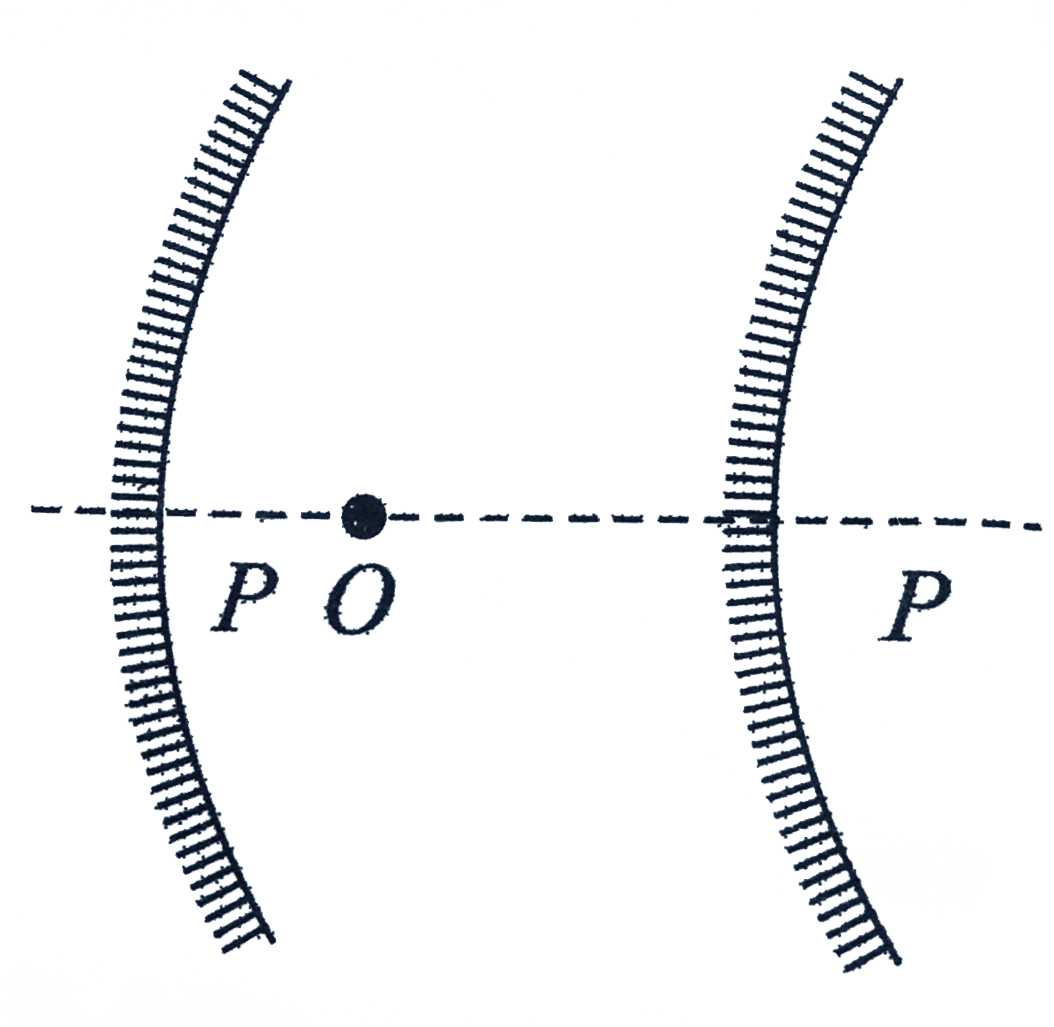
View SolutionA concave mirror of focal length 10cm is placed in front of a convex mirror of focal length 20 cm. The distance between the two mirrors is 20cm. A point object is placed 5cm from the concave mirror. Discuss the formation of image.
View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
View SolutionTwo plano-convex lenses of focal lengths 20cm and 30cm are placed together to form a double convex lens. The final focal length will be
View SolutionA biconvex lens of focal length f1=10cm is placed 40 cm in front of a concave mirror of focal length f2=7.50cm, as shown in the figure. An object, 2 cm high, is placed 20 cm to the left of the lens.
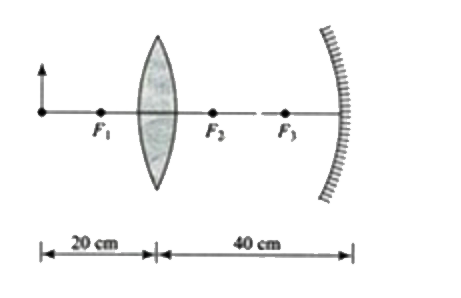
The image formed by the lens, as rays travel to the right, is
View Solution