A point object is placed at a distance 25cm from a convex lens of focal length 20cm. If a glass slab of thickness t annd refractive index 1.5 is inserted between the lens and object, image is formed at . Thickness t is found to be K times of 5cm. Fink K.
The correct Answer is:3
To solve the problem step by step, we will follow the logic presented in the video transcript while elaborating on the calculations involved.
Step 1: Understand the Setup
We have a point object placed at a distance of 25 cm from a convex lens with a focal length of 20 cm. When a glass slab of thickness t and refractive index μ=1.5 is inserted between the lens and the object, the image is formed at infinity.
Step 2: Determine the Condition for Image Formation at Infinity
For the image to be formed at infinity, the object must be at the focal point of the lens. The focal length f of the lens is 20 cm, so the effective object distance u must be equal to the focal length when the glass slab is inserted.
Step 3: Calculate the Shift Due to the Glass Slab
The original object distance is 25 cm. When the glass slab is inserted, the object effectively shifts towards the lens. The shift S caused by the slab can be calculated using the formula:
S=t(1−1μ)
where t is the thickness of the slab and μ is the refractive index of the slab.
Step 4: Substitute Values into the Shift Formula
Given μ=1.5:
S=t(1−11.5)=t(1−23)=t(13)
Step 5: Relate the Shift to the Object Distance
Since the object is originally at 25 cm and must shift to the focal length of 20 cm, the shift S is:
S=25 cm−20 cm=5 cm
Step 6: Set Up the Equation
Now we can set up the equation using the shift we calculated:
5=t(13)
Step 7: Solve for Thickness t
Rearranging the equation gives:
t=5×3=15 cm
Step 8: Relate Thickness t to K
The problem states that the thickness t is found to be K times of 5 cm:
t=K×5 cm
Substituting t=15 cm:
15=K×5
Step 9: Solve for K
Dividing both sides by 5:
K=155=3
Final Answer
Thus, the value of K is 3.
---
We have a point object placed at a distance of 25 cm from a convex lens with a focal length of 20 cm. When a glass slab of thickness t and refractive index μ=1.5 is inserted between the lens and the object, the image is formed at infinity.
For the image to be formed at infinity, the object must be at the focal point of the lens. The focal length f of the lens is 20 cm, so the effective object distance u must be equal to the focal length when the glass slab is inserted.
The original object distance is 25 cm. When the glass slab is inserted, the object effectively shifts towards the lens. The shift S caused by the slab can be calculated using the formula:
Given μ=1.5:
Since the object is originally at 25 cm and must shift to the focal length of 20 cm, the shift S is:
Now we can set up the equation using the shift we calculated:
Rearranging the equation gives:
The problem states that the thickness t is found to be K times of 5 cm:
Dividing both sides by 5:
Thus, the value of K is 3.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Archives
7 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - True/False
5 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A point object is placed at a diatance of 25 cm from a convex lens of focal length 20 cm. If a glass slab of thickness t and refractive index 1.5 is inserted between the lens and the object, the image is formed at infinity. The thickness t is
View SolutionA point object is placed at a distance of 25cm from a convex lens. Its focal length is 22cm. A glass slab of refractive index 1.5 is inserted between the lens and the object, then the image is formed at infinity. Find the thickness of the glass slab (in cm).
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the image
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the image
View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
View SolutionA point object is placed at a distance of 20cm from a thin plano-convex lens of focal length 15cm(μ=1.5). The curved surface is silvered. The image will form at

View SolutionA point object is placed at a distance of 20cm from a thin plano-convex lens of focal length 15cm(μ=1.5). The curved surface is silvered. The image will form at

View SolutionAn object is put at a distance of 5cm from the first focus of a convex lens of focal length 10cm. If a real image is formed, its distance from the lens will be
View SolutionAn object is kept at a distance of 15 cm from a convex lens of focal length 10 cm. Calculate the image distance and state the characteristics of the image formed.
View SolutionA point object O is placed in front of a concave mirror of focal length 10cm. A glass slab of refractive index μ=3/2 and thickness 6cm is inserted between the object and mirror.
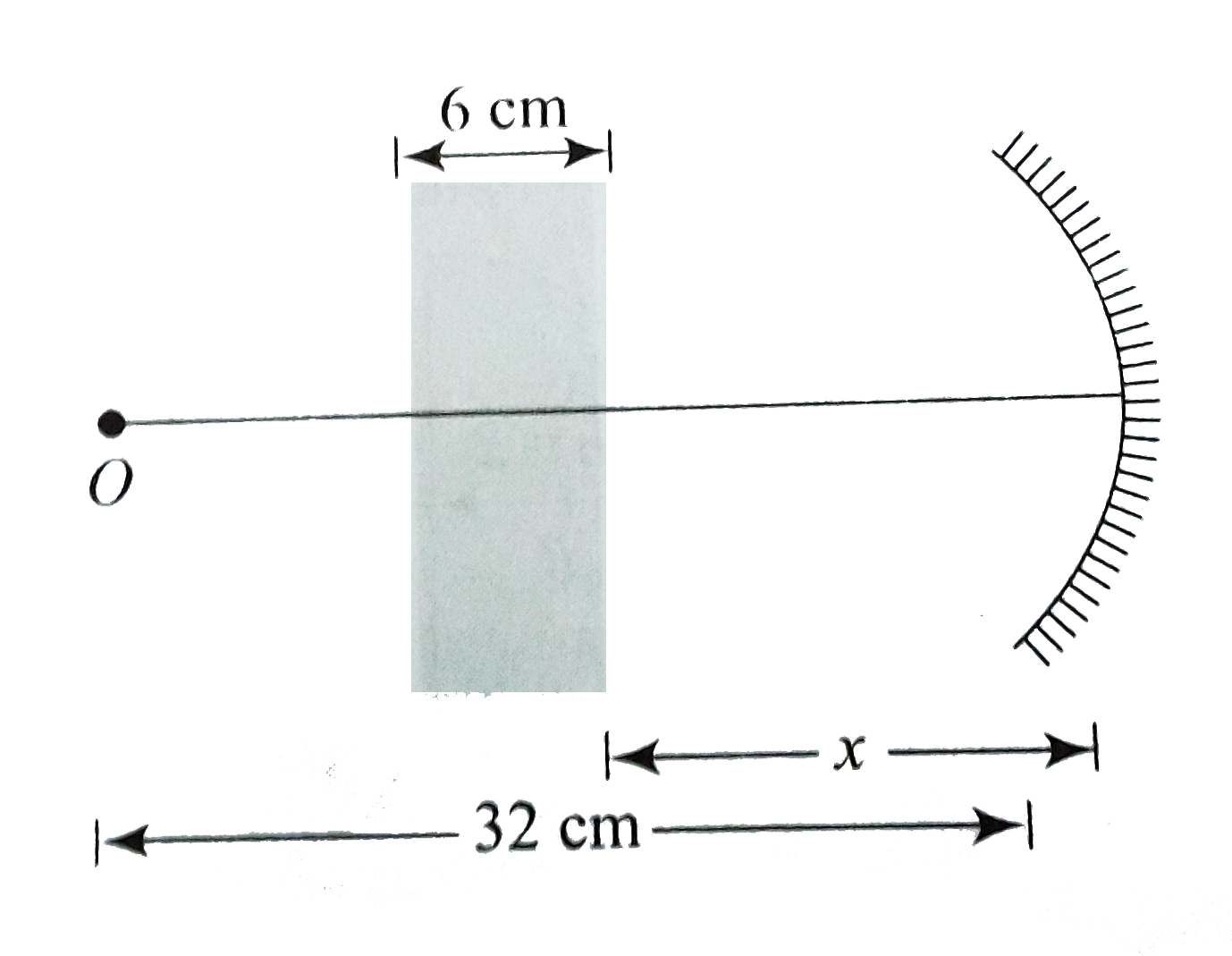
Q. Find the position and nature of the final image when the distance x shown in figure, is 5cm.
View Solution
