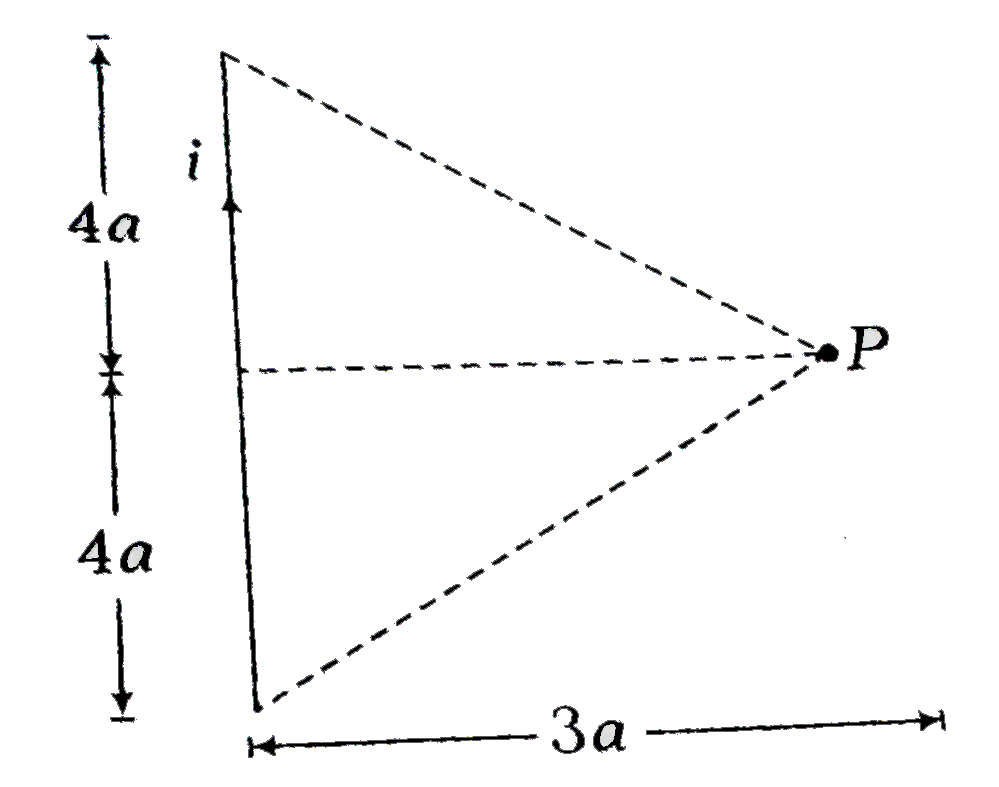
Two semicircles shown in Fig. have radii a and b. Calculate the net magnetic field (magnitude and direction) that the current in the wires produces at point P.
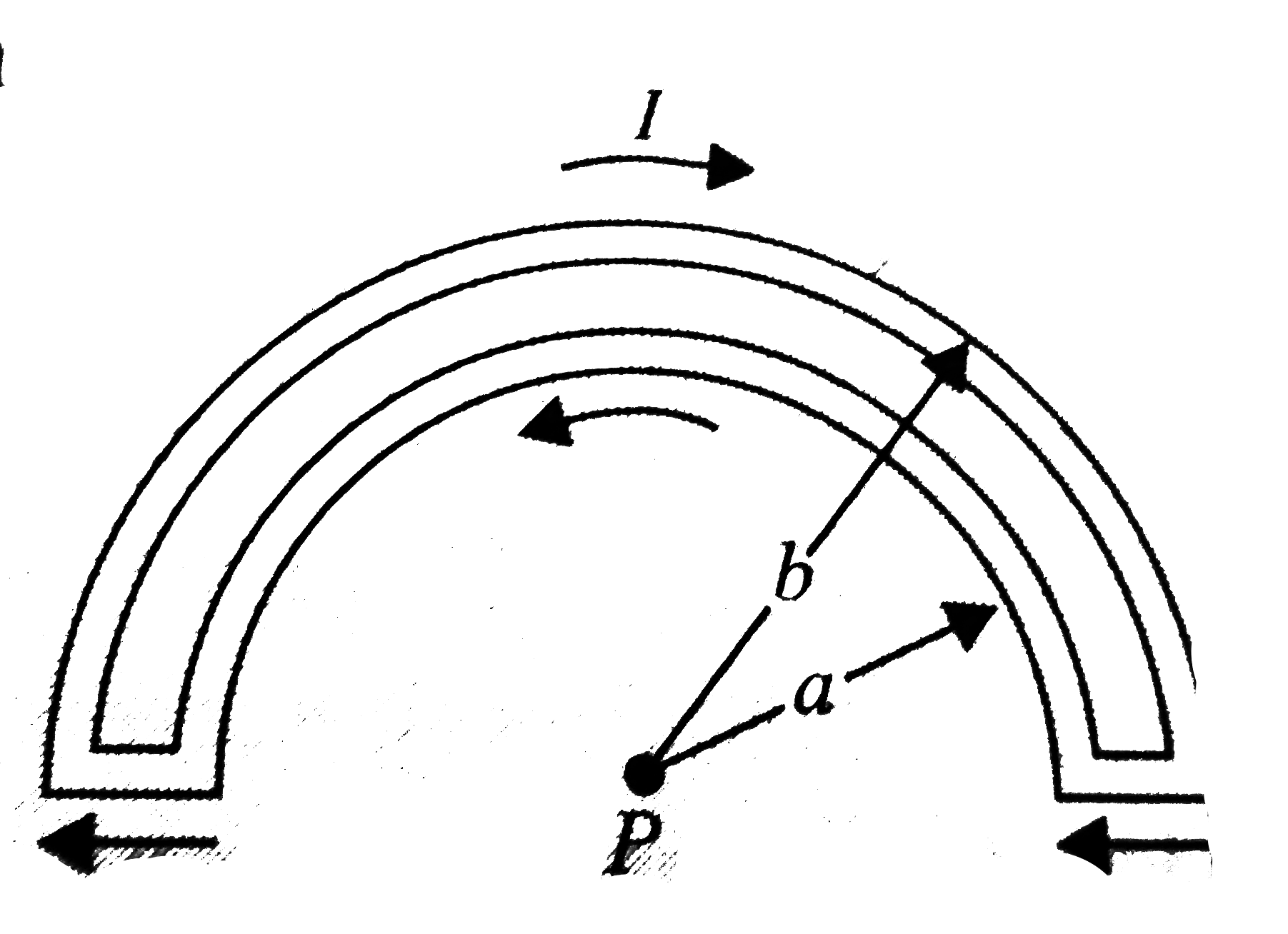
B=(Ba−Bb)=12(μ0I2)(1a−1b)
B=μ0I4a(1−ab), out of the page.
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Concept Exercise 2.2
10 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (subjective )
10 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Solved Example
20 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type
14 Videos
Similar Questions
What is the net magnetic field at point O for the current distribution shown here?
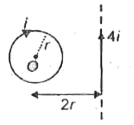
View SolutionThe magnetic field at O due to current in the infinite wire forming a loop as a shown in Fig.
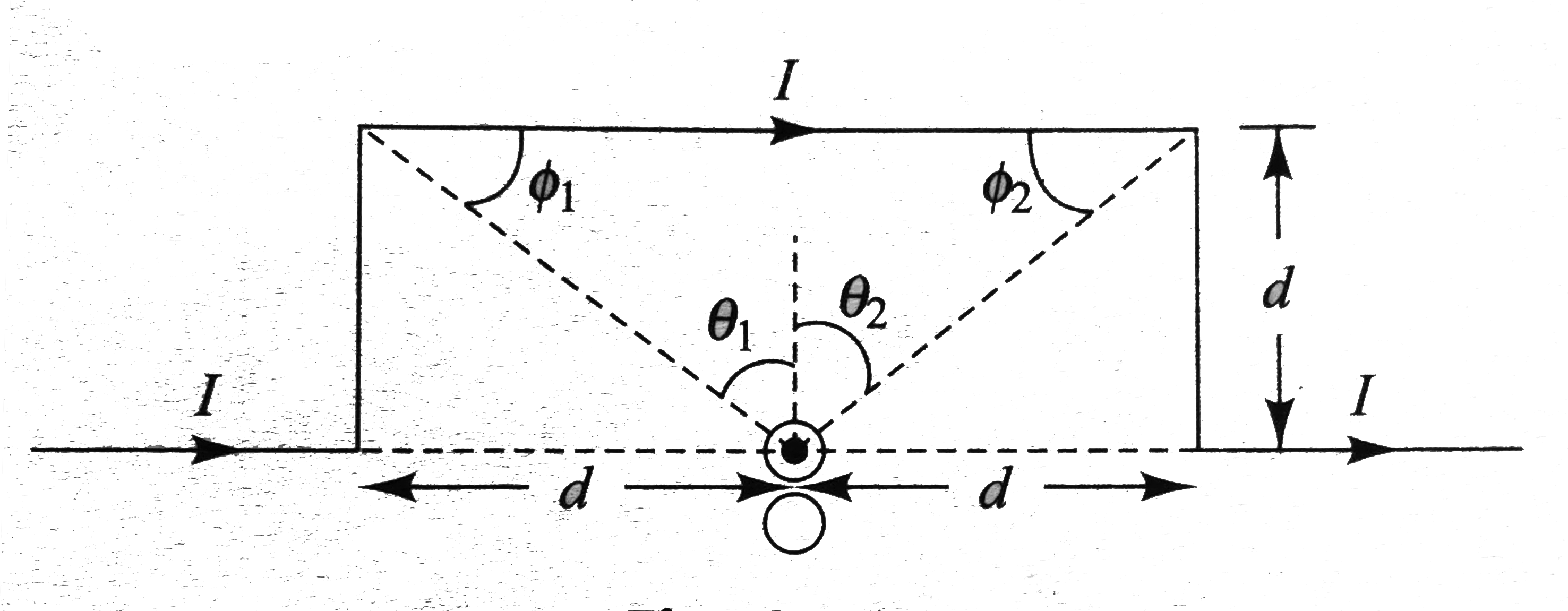
View SolutionFour long wires each carrying current I as shown in Fig. are placed at points A, B, C and D. Find the magnitude and direction of

Force per metre acting on wire at point D
View SolutionFind the magnitude and direction of magnetic field at point P due to the current carrying wire as shown in

View SolutionA long, circular pipe, with an outside radius R, carries a (uniformly distributed) current i0 (into the paper as shown in Fig.) A wire runs parallel to the pipe at a distance 3R from centre to centre. Calculate the magnitude and direction of the current in the wire that would cause the resultant magnetic field at point P to have the same magnitude, but the opposite direction, as the resultant field at the centre of the pipe.

View SolutionA straight peice of conducting wire with mass M and length L is placed on a frictionless incline tilited at an angle θ from the horizontal (as shown in fig) There is a uniform. Vertical magnetic field at all points (produced by an arrangement of magnets not shown in fig. To keep the wire from sliding down the incline, a voltage source is

attached to the ends of the wire. When just the right amount of current flows through the wire, the wire remains at rest. Determine the magnitude and direction of the current in the wire that will cause the wire to remain at rest.
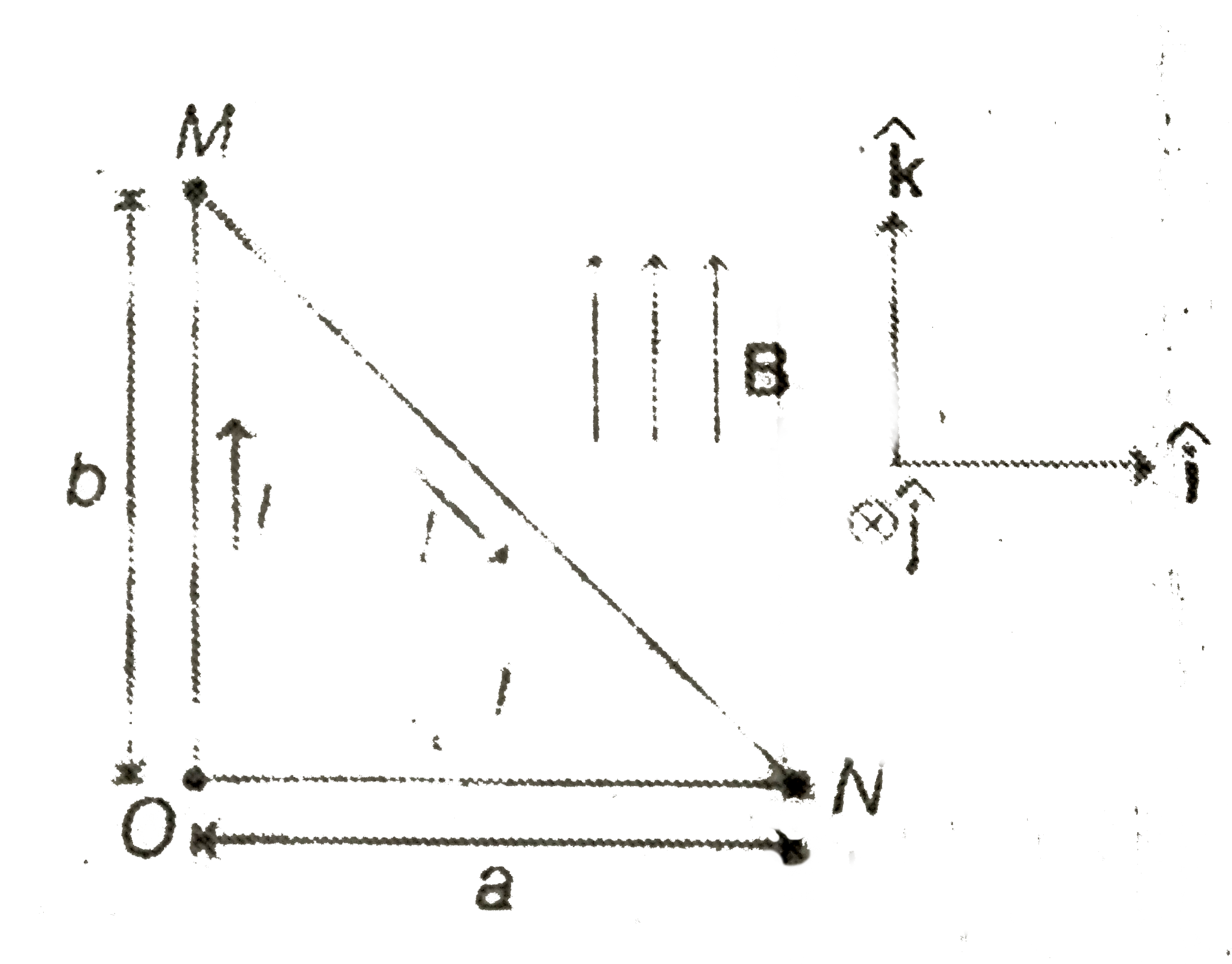
View Solutionconsider a triangular loop of wire with sides a and b. The loop carries a current I in the direction shown and is placed in a uniform magnetic field that has magnitude B and points in the same direction as the current in side OM of the loop. At the moment shown in the figure the torque on the current loop is τ. Find the value of IabB/τ
View SolutionEvaluate magnitude and direction of magnetic field at a point P in the following cases

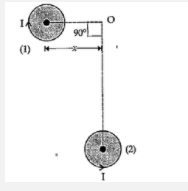
View SolutionTwo very small identical circular loops (1)and(2) carrying equal currents I are placed vertically (w.r.t. the plane of the paper) with their geometrical axes perpendicualr to each other as shown in figure. Find the magnitude and direction of the net magnetic field produced at point O.
View SolutionFour long, parallel conductors carry equal currents of 5.0. The direction of the currents is into the pge at points. A and B and out of the page at C and D. Calculate the magnitude and directionof the mgnetic field at point P, located at the centre of the square.

View Solution
