Charge Q is uniformly distributed on a thin insulating ring of mass m which is initially at rest. To what angular velocity will the ring be accelerated when a magnetic field B, perpendicular to the plane of the ring, is switched on ?
(a) QB2m
(b) 3QB2m
( c) QBm
(d) QB4m
The correct Answer is:A
To solve the problem, we need to analyze the situation when a magnetic field B is applied perpendicular to the plane of a thin insulating ring with charge Q uniformly distributed on it. The ring has a mass m and is initially at rest.
1. Understand the Setup:
- We have a thin insulating ring of radius r with charge Q uniformly distributed.
- The ring is initially at rest, and we apply a magnetic field B perpendicular to its plane.
2. Induced Electric Field:
- When the magnetic field is switched on, it changes with time, which induces an electric field E in the ring due to Faraday's law of electromagnetic induction.
- The induced electric field E around the ring can be expressed as:
E=−dΦBdt
- The magnetic flux ΦB through the ring is given by:
ΦB=B⋅A=B⋅πr2
- Therefore, the induced electric field becomes:
E=−d(B⋅πr2)dt=−πr2dBdt
3. Force on the Charge:
- The force F on the charge Q due to the electric field E is given by:
F=Q⋅E=Q⋅(−πr2dBdt)=−Qπr2dBdt
4. Torque on the Ring:
- The torque τ acting on the ring due to this force is given by:
τ=r⋅F=r⋅(−Qπr2dBdt)=−Qπr3dBdt
5. Angular Acceleration:
- The angular acceleration α of the ring can be related to the torque by using the moment of inertia I of the ring:
τ=I⋅α
- For a thin ring, the moment of inertia I is given by:
I=mr2
- Thus, we have:
−Qπr3dBdt=mr2α
- Rearranging gives:
α=−QπrmdBdt
6. Finding Angular Velocity:
- The angular velocity ω can be found by integrating the angular acceleration over time:
ω=α⋅t=−QπrmdBdt⋅t
- Therefore, the final expression for the angular velocity when the magnetic field is switched on is:
ω=−QπrmdBdt⋅t
Final Answer:
The angular velocity ω of the ring when the magnetic field B is switched on is given by:
ω=−QπrmdBdt⋅t
- We have a thin insulating ring of radius r with charge Q uniformly distributed.
- The ring is initially at rest, and we apply a magnetic field B perpendicular to its plane.
- When the magnetic field is switched on, it changes with time, which induces an electric field E in the ring due to Faraday's law of electromagnetic induction.
- The induced electric field E around the ring can be expressed as:
E=−dΦBdt
- The magnetic flux ΦB through the ring is given by:
ΦB=B⋅A=B⋅πr2
- Therefore, the induced electric field becomes:
E=−d(B⋅πr2)dt=−πr2dBdt
- The force F on the charge Q due to the electric field E is given by:
F=Q⋅E=Q⋅(−πr2dBdt)=−Qπr2dBdt
- The torque τ acting on the ring due to this force is given by:
τ=r⋅F=r⋅(−Qπr2dBdt)=−Qπr3dBdt
- The angular acceleration α of the ring can be related to the torque by using the moment of inertia I of the ring:
τ=I⋅α
- For a thin ring, the moment of inertia I is given by:
I=mr2
- Thus, we have:
−Qπr3dBdt=mr2α
- Rearranging gives:
α=−QπrmdBdt
- The angular velocity ω can be found by integrating the angular acceleration over time:
ω=α⋅t=−QπrmdBdt⋅t
- Therefore, the final expression for the angular velocity when the magnetic field is switched on is:
ω=−QπrmdBdt⋅t
The angular velocity ω of the ring when the magnetic field B is switched on is given by:
ω=−QπrmdBdt⋅t
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Multiple Correct
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Asserton - Reasoning
8 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Subjective
13 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
Similar Questions
Charge Q is uniformly distributed on the rim of a thin insulating disc of mass m which is initially at rest and placed on a smooth horizontal surface. What will be the angular velocity of the disc if a magnetic field B, perpendicular to the plane of the disc is switched on ?
View SolutionCharge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View Solution
Knowledge Check
A conducting ring is placed in a uniform magnetic field with its plane perpendicular to the field . An emf is induced in the ring if
Ait is rotated about its axis.Bit is rotated about its diameter.Cit is not moved.Dit is given translational motion in the field.
A positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The

View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.

View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionA conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.

View SolutionA conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.

View SolutionA charge q is unifomly distrybuted over a nonconducting ring of radius R. The ring is rotated about an axis passing through its centre and perpendicular to the plane of the ring with frequency f. The ratio of electric potential to the magnetic field at the centre of the ring depends on.
View SolutionA charge Q is uniformly distributed over a ring which is rotating with constant angular velocity ω about an axis passing through its centre and perpendicular to the plane. A wire which carries a current I is lying perpendicular to the plane of the irng along its axis having one end at its centre. Find resultant magnetic force on the wire by the ring.
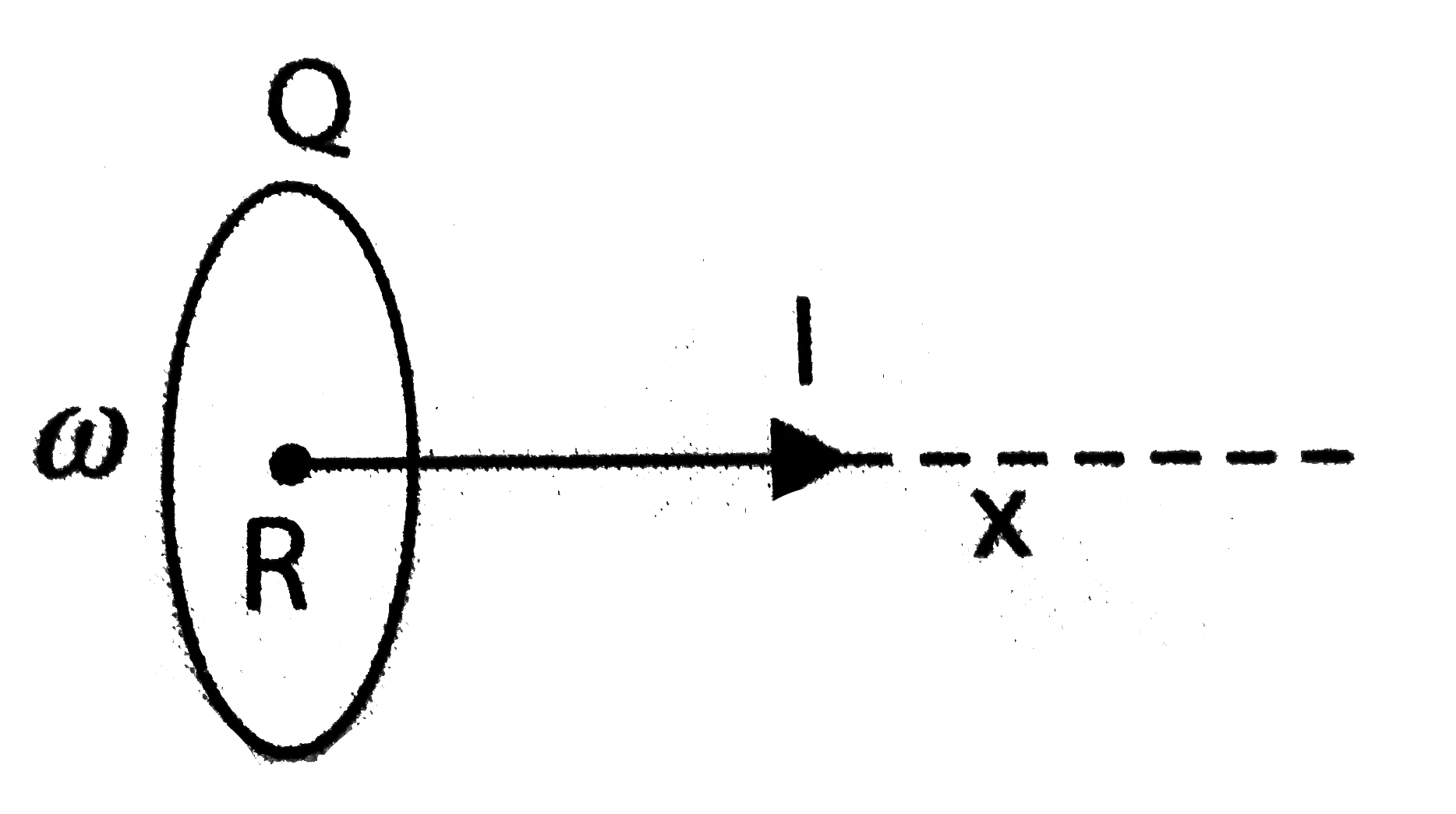
View Solution