A ball of amss m is connected to two rubber bands of length L, each under tension T as shown in figure . The ball is diplaced by a small distance y perpendicular to the length of the rubber band. Assuming the tension does not change , shown that
(a) the restoring force is −(2T/L)y
(b) the sysytem exbibites simple harmonic motion with an angular frequency ω=√2T/mLs.

a. If we displace the block upward the net tension towards mean position will proved the restoration force on the block.
∑¯¯¯F=−2Tsinθ, where θ=tan−1(y/L)
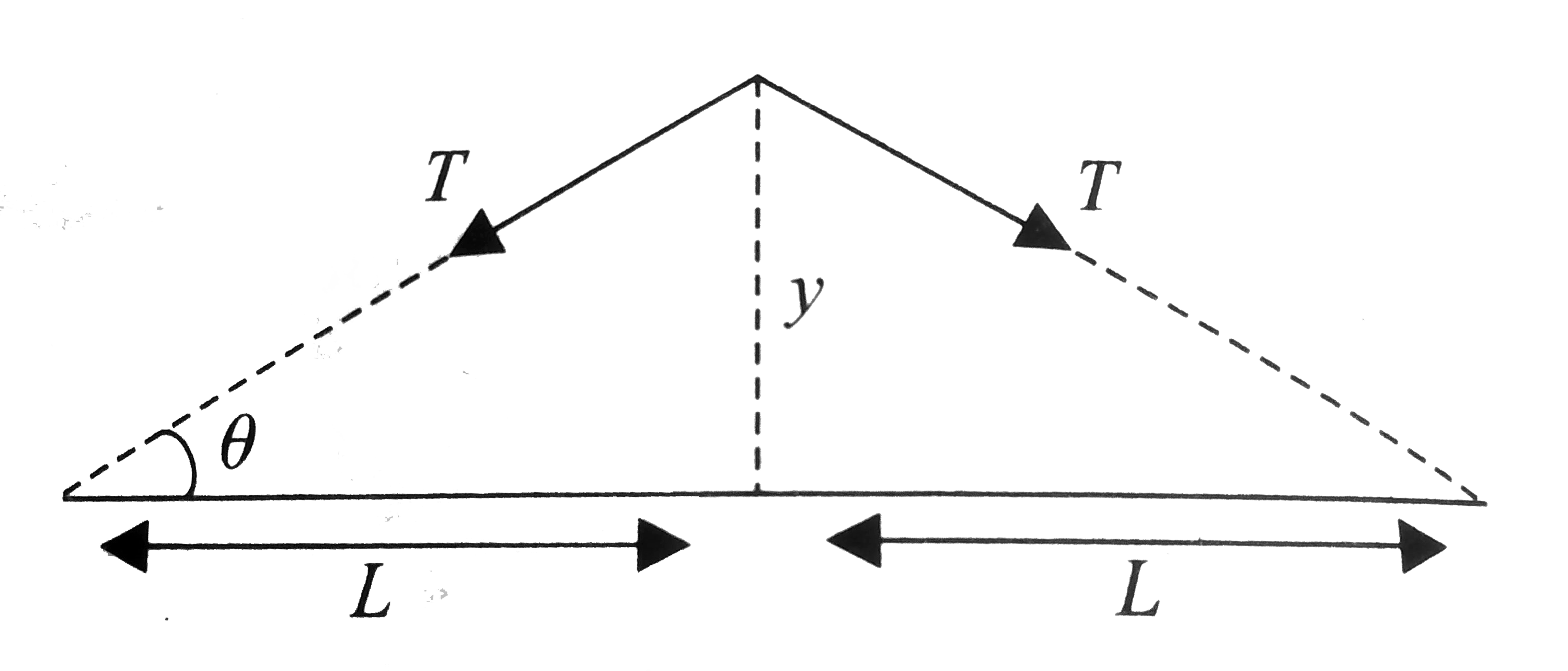
Since for a small displacement.
sinθ=tanθ=yL
and the resultant is
∑¯¯¯F=(−2TyL)
b. Since there is a restoring force that is proportional to the position , it cause the system to move with simple harmonic motion like a block-spring system . Thus, we can think of
ω=√km=sqer(2TmL)
Not enything with a stable equilirium position will exercute simple harmonic motion when disturb and released, but a lot of things do when the disturbance is small.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Subjective21videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct107videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.123videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger11videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type3videos
MISCELLANEOUS KINEMATICS
Similar Questions
The tension T in the string shown in figure is
 View Solution
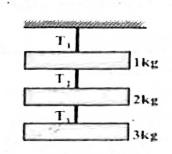
View SolutionFind the tension T2 in the system shown in the figure.
 View Solution
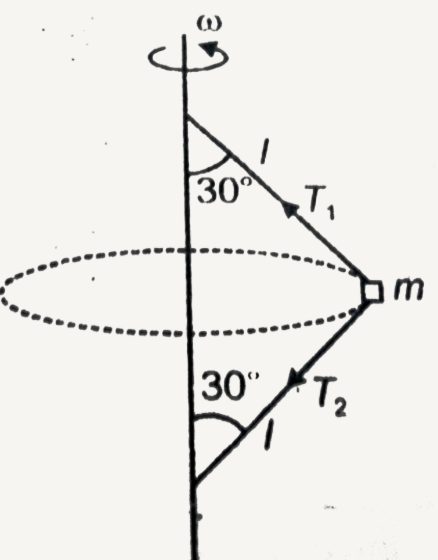
View SolutionA particle of mass m is whirled in horizontal circle with the help of two threads of length l each as shown in figure . Angular velocity equals ω, then
 View Solution
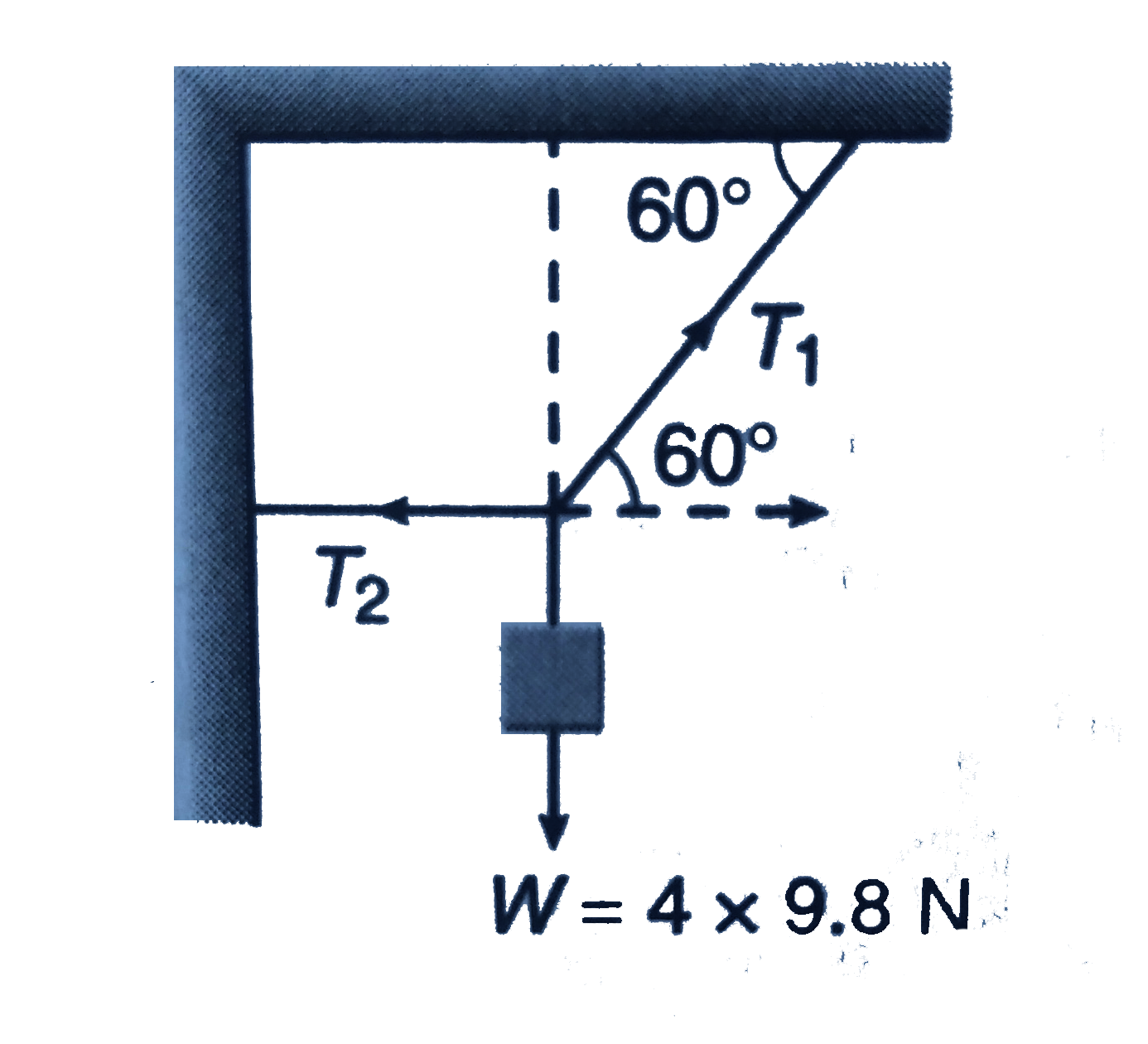
View SolutionDetermine the tensions T1 and T2 in the string as shown in figure.
 View Solution
View SolutionDetermine the tensions T1 and T2 in the string as shown in figure.
 View Solution
View SolutionA rod of length L and mass M is acted on by two unequal force F1andF2(<F1) as shown in the following figure.

The tension in the rod at a distance y from the end a is given byView SolutionA particle of mass m is whirled in horizontal circle with the help of two threads of length l each as shown in figure . Angular velocity equals ω, then
T1 equalsView SolutionThree balls each of mass 1 kg are attached with three strings each of length 1 m as shown in figure. They are rotated in a horizontal circle with angular velocity ω=4rad s−1 about point O.find the tensions .
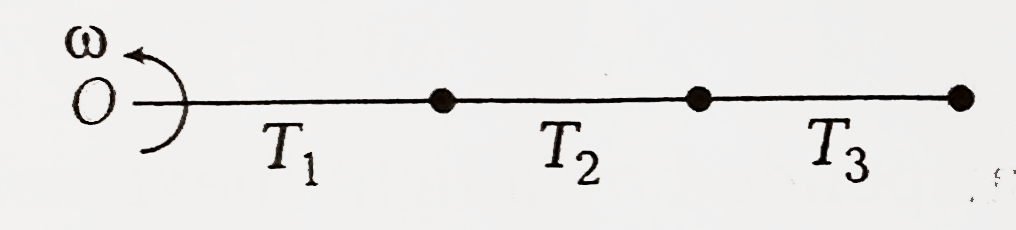 View Solution
View SolutionTwo uniform thin rods each of length L and mass m are joined as shown in the figure. Find the distance of centre of mass the system from point O
 View Solution
View SolutionA small ball B of mass m is suspended with light inelastic string of length L from a block A of same mass in which can move on smooth horizontal surface as shown in the figure. The ball is displaced by angle θ from equilibrium position and then released.
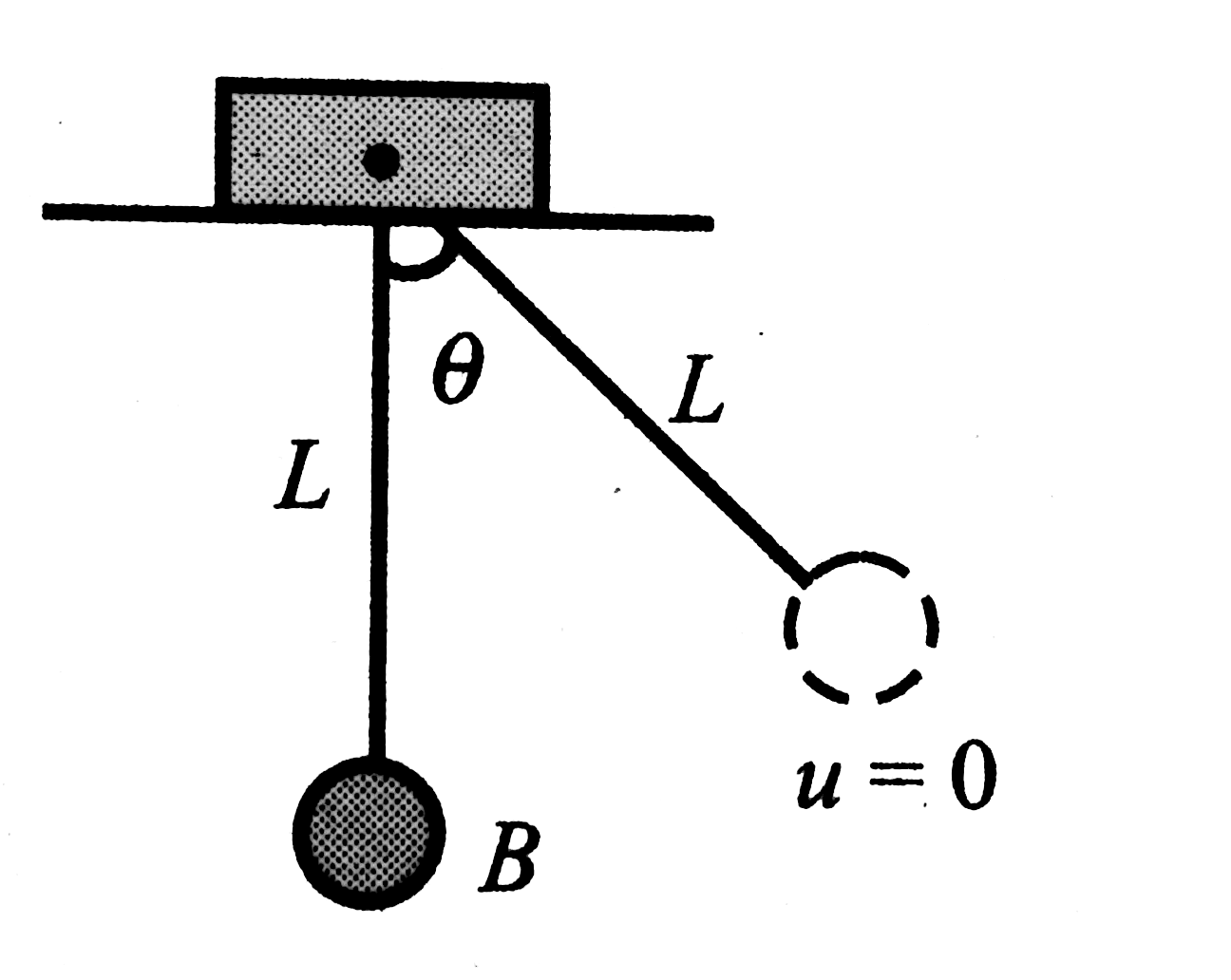
Tension in string when it is vertical, isView Solution

