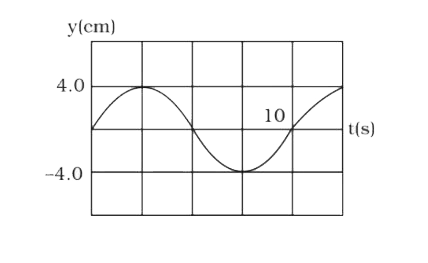
A simple harmonic plane wave propagatees along x-axis in a medium. The displacement of the particle as a function of time is shown in figure, for x=0(curve 1) and x=7 (curve 2)
The two particle are with a span of onewavelength.
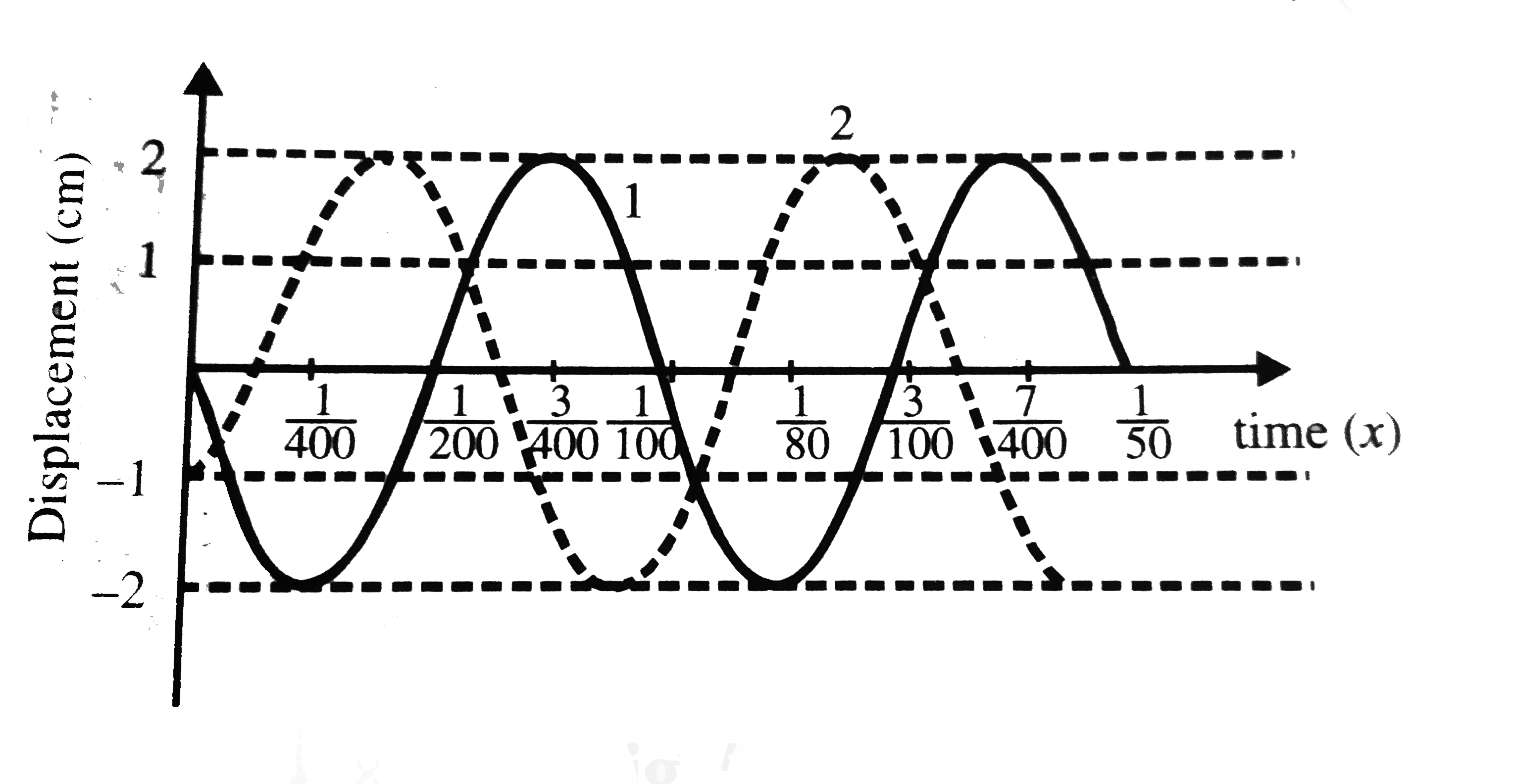
The wavelength of the wave is
6cm
24cm
12cm
16cm
The correct Answer is:c
Let general wave equation be y=Asin(ωt−kx+ϕ)
v=dydt=Aωcos(ωt−kx+ϕ)
For curve (1),x=0
at t=0,x=0, we have y=0
⇒0=Asin[ϕ]⇒sinϕ=0
⇒ϕ=0 or π
here ϕ=π (because velocity is negative) for curve (2),×=7cm
t=0,x=7cm,y=−1
−1=sin(−k×7+π)
⇒sin(−7k+π)=−1/2
⇒−7k+π=2nπ+7π6 or 2nπ+11π6
here ⇒−7k+π=2nπ+11π6
(because at t=0, velocity is positive)
⇒−7(2πλ)=2nπ+5π6
implieslammbda=(-14pi)/((5pi)/(6)+2npi)<br>
⇒λ=−8412n+5
for n=−1,λ=12cm
for n=−2,λ=8419cm (not possible)
because λ>7cm
v=fλ=100×12100=12m/s
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer9videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning6videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A simple harmonic plane wave propagatees along x-axis in a medium. The displacement of the particle as a function of time is shown in figure, for x=0(curve 1) and x=7 (curve 2)
The two particle are with a span of onewavelength.
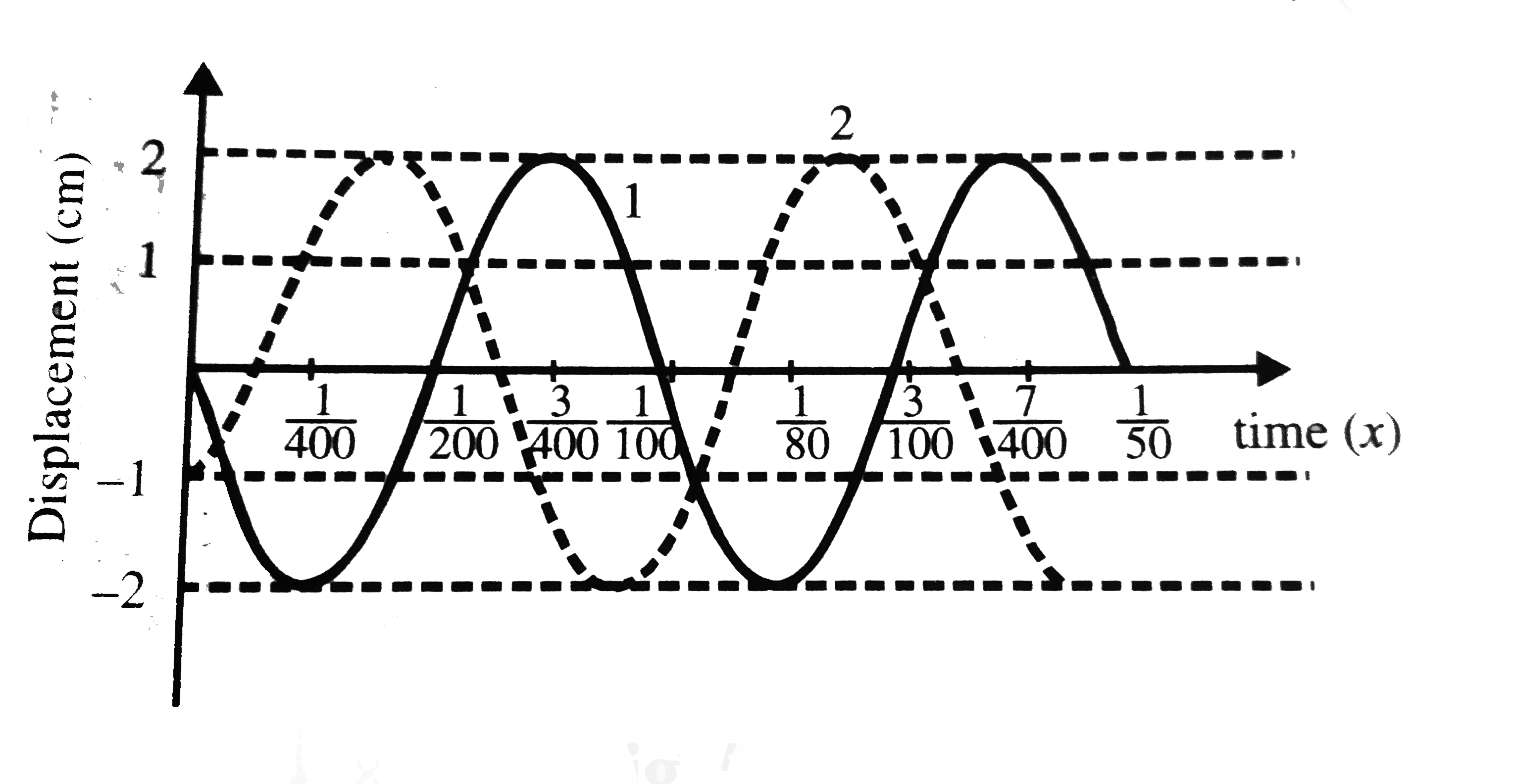
The speed of the wave isView SolutionA mechanical wave propagastes in a medium along the X-axis. The particles of the medium
View SolutionA transverse wave travels along x-axis . The particles of medium move
View SolutionFigure shows the displacement of a particle going along the X-axis as a function of time. The force acting on the particle is zero in the region
 View Solution
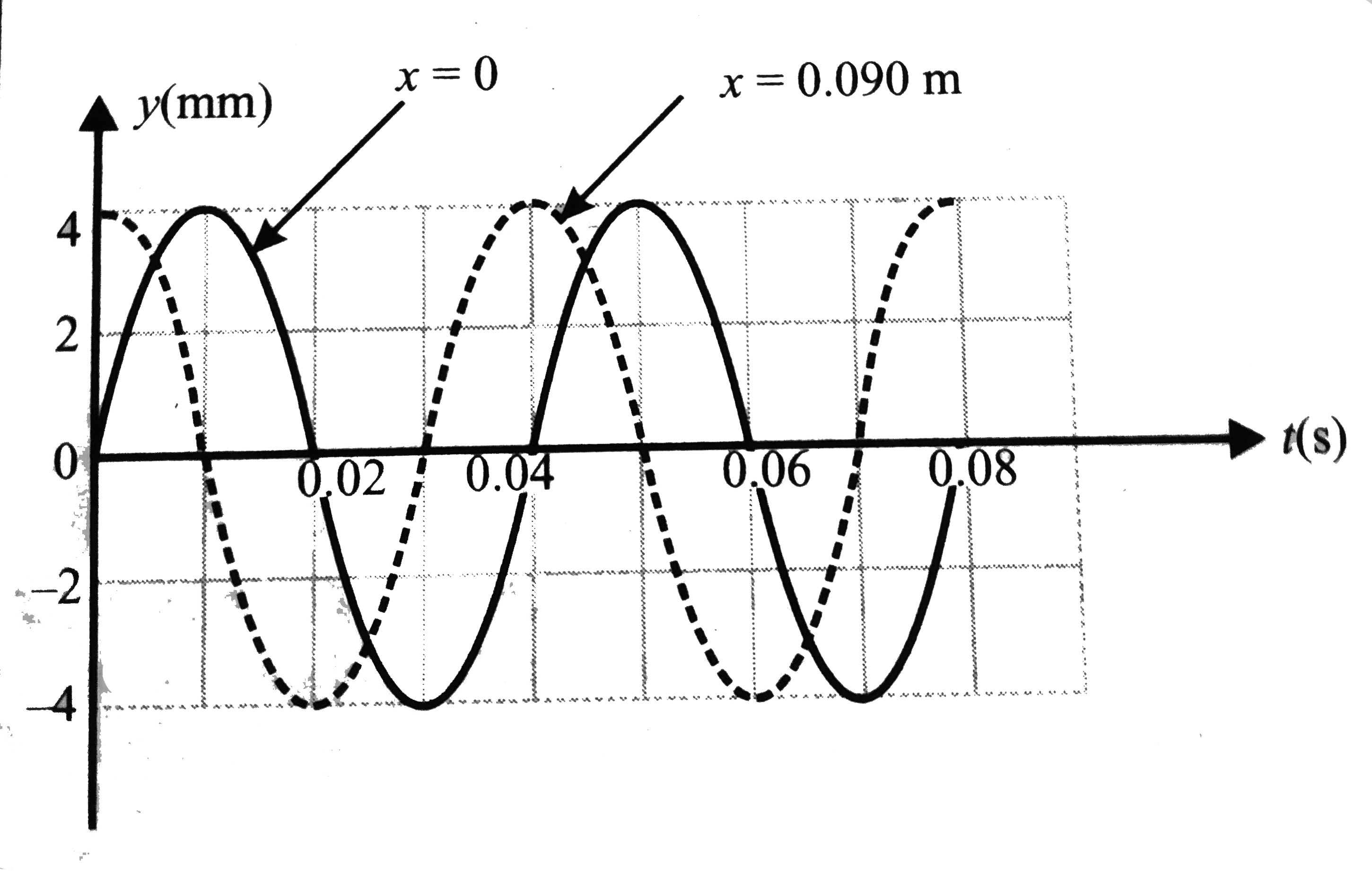
View SolutionA sinusoidal wave is propagating along a streched string that lies along the x-axis. The displacement of the string as a function of time is graphed in for particles at x-0 and at x=0.0900 m. (a) what is the amplitude of the wave? (b)what is the period of the wave? (c ) you are told that the two points x=0 and x=0.0900 m are within one wavelength of each other. if the wave is moving in the +x-direction, determine the wavelength and the wave speed. (d) if instead the wave is moving in the -x-direction, determine the wavelength and the wave speed. (e) would it be possible to derermine definitely the wavelength in parts (c ) and (d) if you were not told that the two points were within one wavelength of each other? why ot why not?
 View Solution
View SolutionSound waves are trqvelling along positive x - direction. Displacement of particle at any time t is as shows in figure. Select the correct statement.
 View Solution
View SolutionFigure shows the displacement time graph of a particle moving on the X-axis
 View Solution
View SolutionFigure shows the displacement-time graph of a particle moving on the X-axis
 View Solution
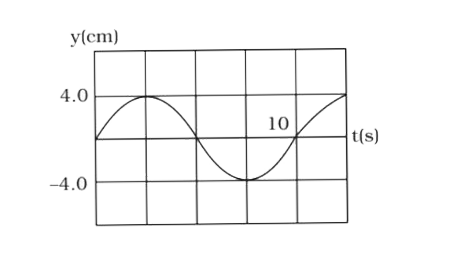
View SolutionA transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. the transverse displacement of the string particle at x=0 as a function of time is shown in figure.
Q. The velocity of propagation of the wave is ?View SolutionA transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. The transverse displacement of the string particle at as a function of time is shown in Figure.
The equation of the wave with all the constants evaluated is:
 View Solution
View Solution

