A wire of diameter 0.04cm and made of steel of density 8000kg/m3 is under a tension of 80N. A fixed length of 50cm is set into transverse vibrations . How would you cause vibrations of frequency 840Hz to predominate in intensity ?
To solve the problem, we need to determine how to achieve a frequency of 840 Hz in a steel wire under tension. Here’s a step-by-step breakdown of the solution:
Step 1: Calculate the Cross-Sectional Area of the Wire
Given the diameter of the wire is 0.04cm, we first convert this to meters:
Diameter=0.04cm=0.0004m
The radius r is half of the diameter:
r=0.00042=0.0002m
The cross-sectional area A of the wire is given by:
A=πr2=π(0.0002)2=π(4×10−8)≈1.2566×10−7m2
Step 2: Calculate the Mass per Unit Length (μ)
The mass per unit length μ can be calculated using the density ρ and the cross-sectional area A:
μ=ρ⋅A=8000kg/m3×1.2566×10−7m2≈1.0053×10−3kg/m
Step 3: Calculate the Velocity of the Transverse Wave
The velocity v of the transverse wave in the wire is given by the formula:
v=√Tμ
Where T is the tension in the wire. Given T=80N:
v=√801.0053×10−3≈√79663.15≈282.5m/s
Step 4: Relate Frequency, Wavelength, and Length of the Wire
The frequency f of a vibrating string is related to its wavelength λ and the length L of the string. For a string fixed at both ends, the relationship is given by:
f=n⋅v2L
Where n is the harmonic number. Given L=0.5m and we want f=840Hz:
840=n⋅282.52⋅0.5
This simplifies to:
840=n⋅282.5
Solving for n:
n=840282.5≈2.97≈3
Step 5: Determine the Position to Strike the Wire
Since n=3, this corresponds to the third harmonic. The wavelength λ for the third harmonic is:
λ=2Ln=2×0.53=13m≈33.33cm
To achieve this harmonic, you should strike the wire at a distance of:
L6=0.56≈0.0833m≈8.33cm
from one end.
Final Answer
To cause vibrations of frequency 840 Hz to predominate in intensity, strike the wire at approximately 8.33cm from one end.
Given the diameter of the wire is 0.04cm, we first convert this to meters:
Diameter=0.04cm=0.0004m
The radius r is half of the diameter:
r=0.00042=0.0002m
The cross-sectional area A of the wire is given by:
A=πr2=π(0.0002)2=π(4×10−8)≈1.2566×10−7m2
The mass per unit length μ can be calculated using the density ρ and the cross-sectional area A:
μ=ρ⋅A=8000kg/m3×1.2566×10−7m2≈1.0053×10−3kg/m
The velocity v of the transverse wave in the wire is given by the formula:
v=√Tμ
Where T is the tension in the wire. Given T=80N:
v=√801.0053×10−3≈√79663.15≈282.5m/s
The frequency f of a vibrating string is related to its wavelength λ and the length L of the string. For a string fixed at both ends, the relationship is given by:
f=n⋅v2L
Where n is the harmonic number. Given L=0.5m and we want f=840Hz:
840=n⋅282.52⋅0.5
This simplifies to:
840=n⋅282.5
Solving for n:
n=840282.5≈2.97≈3
Since n=3, this corresponds to the third harmonic. The wavelength λ for the third harmonic is:
λ=2Ln=2×0.53=13m≈33.33cm
To achieve this harmonic, you should strike the wire at a distance of:
L6=0.56≈0.0833m≈8.33cm
from one end.
To cause vibrations of frequency 840 Hz to predominate in intensity, strike the wire at approximately 8.33cm from one end.
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Subjective
24 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Single Correct
144 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
Similar Questions
A steel wire 0.72 m long has a mass of 5.0×10−3 kg . If the wire is under a tension of 60 N, what is the speed of transverse waves on the wire ?
View SolutionA wire of length 2.00 m is stretched to a tension of 160 N. If the fundamental frequency of vibration is 100 Hz, find its linear mass density.
View Solution
Knowledge Check
A string of mass 3 kg is under tension of 400 N. the length of the stretched string is 25 cm. if the transverse jerk is stuck at one end of the string how long does the disturbance take to reach the other end?
A0.043sB4.33SC0.055 sD0.034 s
A wire having a linear density 0.1kg/m is kept under a tension of 490N. It is observed that it resonates at a frequency of 400 Hz. The next higher frequency is 450 Hz. Find the length of the wire.
View SolutionTwo wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is 1.5m and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.
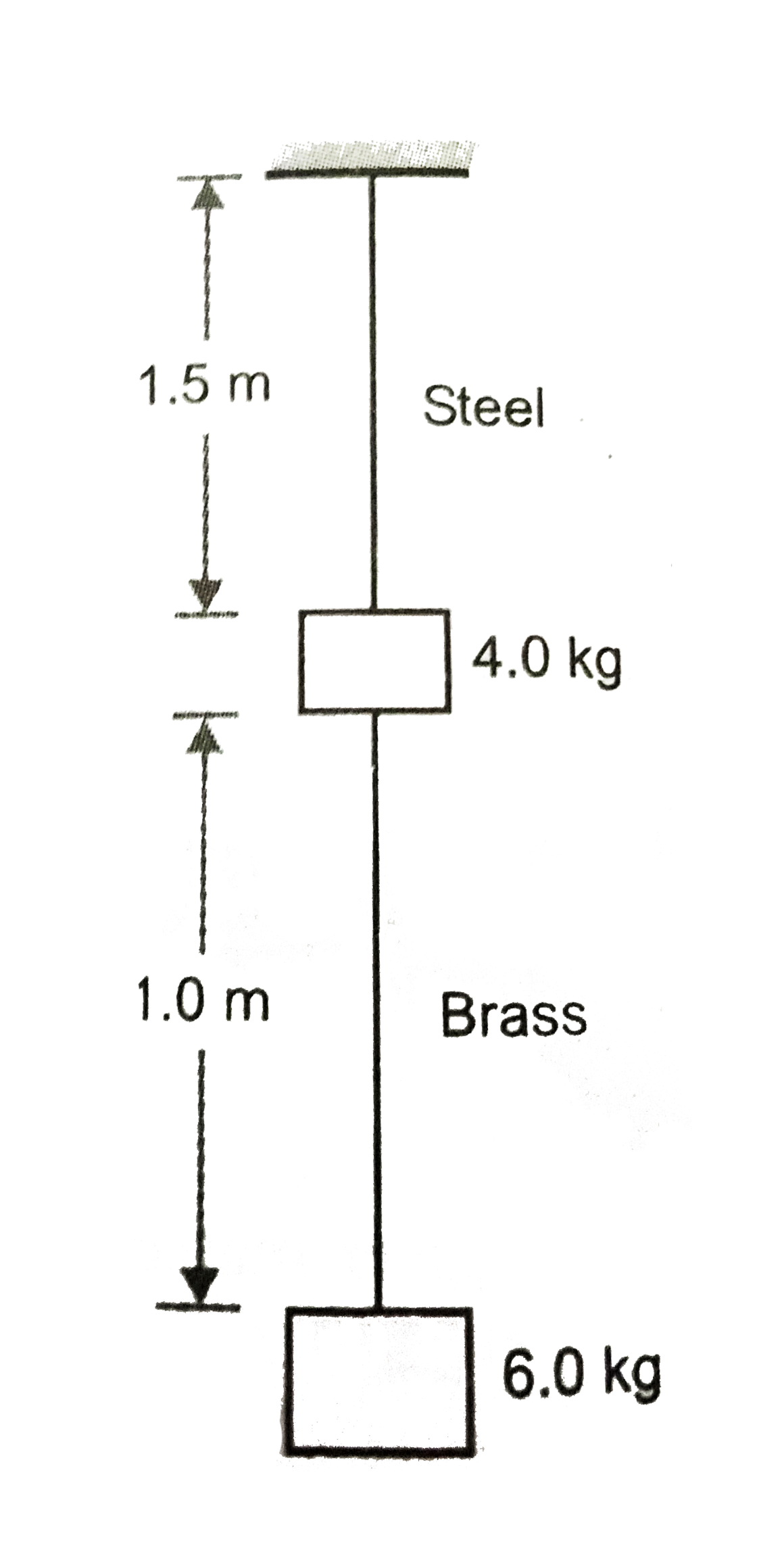
View SolutionA long string having a cross- sectional area 0.80mm2 and density 12.5gcm−3 is subjected to a tension of 64 N along the X-axis. One end of this string is attached to a vibrator moving in transverse direction at a frequency fo 20Hz. At t = 0. the source is at a maximujm displacement y= 1.0 cm. (a) Write the equation for the wave. (b) What is the displacement of the particle of the string at x = 50 cm at time t = 0.05 s ? (c) What is the velocity of this particle at this instant ?
View SolutionA string of length 1 m and mass 5 g is fixed at both ends. The tension in the string is 8.0 N. The string is set into vibration using an external vibrator of frequency 100 Hz. The separation between successive nodes on the string is close to :
View SolutionA steel wire 75cm long has mass of 5.5 10−3kg. If the wire is under a tension of 65N, what is the speed of transverse waves on the wire?
View SolutionA stretched string of length 1m fixed at both ends , having a mass of 5×10−4kg is under a tension of 20N. It is plucked at a point situated at 25cm from one end . The stretched string would vibrate with a frequency of
View SolutionA stretched string of length 1m fixed at both ends , having a mass of 5×10−4kg is under a tension of 20N. It is plucked at a point situated at 25cm from one end . The stretched string would vibrate with a frequency of
View Solution
