A 150 m long metal wire connects points A and B. The electric potential at point B is 50V less than that at point A. If the conductivity of the metal is 60×106mho/m then magnitude of the current density in the wire is equal to:
11×10−4A/m2
5.5×10−3A/m2
4×107A/m2
20×106A/m2
The correct Answer is:D
To solve the problem, we need to find the magnitude of the current density in the wire connecting points A and B. Let's break down the solution step by step.
Step 1: Understand the given information
We have:
- Length of the wire, L=150m
- Potential difference, V=50V (the potential at point B is 50 V less than at point A)
- Conductivity of the metal, σ=60×106mho/m
Step 2: Calculate resistivity
The resistivity ρ is the reciprocal of conductivity:
ρ=1σ=160×106Ω⋅m
Step 3: Calculate resistance of the wire
The resistance R of the wire can be calculated using the formula:
R=ρLA
Substituting the values we have:
R=(160×106)150A
Step 4: Simplify the resistance expression
To simplify:
R=15060×106⋅A=2.5106⋅AΩ
Step 5: Calculate the current using Ohm's Law
Using Ohm's Law, the current I can be calculated as:
I=VR
Substituting the values:
I=502.5106⋅A=50⋅106⋅A2.5
I=20×106A
Step 6: Calculate current density
Current density J is defined as:
J=IA
Substituting the expression for current:
J=20×106⋅AA=20×106A/m2
Final Answer
The magnitude of the current density in the wire is:
20×106A/m2
We have:
- Length of the wire, L=150m
- Potential difference, V=50V (the potential at point B is 50 V less than at point A)
- Conductivity of the metal, σ=60×106mho/m
The resistivity ρ is the reciprocal of conductivity:
ρ=1σ=160×106Ω⋅m
The resistance R of the wire can be calculated using the formula:
R=ρLA
Substituting the values we have:
R=(160×106)150A
To simplify:
R=15060×106⋅A=2.5106⋅AΩ
Using Ohm's Law, the current I can be calculated as:
I=VR
Substituting the values:
I=502.5106⋅A=50⋅106⋅A2.5
I=20×106A
Current density J is defined as:
J=IA
Substituting the expression for current:
J=20×106⋅AA=20×106A/m2
The magnitude of the current density in the wire is:
20×106A/m2
Topper's Solved these Questions
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Combination of Resistance 1
14 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Combination of Resistance 2
14 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type
22 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
Similar Questions
A copper wire has a square cross section of 6 mm on a side. The wire is 10 m long and carries a current of 3.6A. The density of free electrons id 8.5x1028/m3.Find the magnitude of (a) the current density in the wire , (b) the electric field in the wire. (c ) How much time is required for an electron to travel the length of the wire? (rh, electrical resistivity, is1.72×10−8Ωm.)
View SolutionCalculate the conductivity for a wire of length 60 cm with current density 1×10−7 and potential difference of 3V.
View Solution
Knowledge Check
If a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
A5.34×10−5TB8.34×10−5TC9.6×10−5TD10.2×10−5T
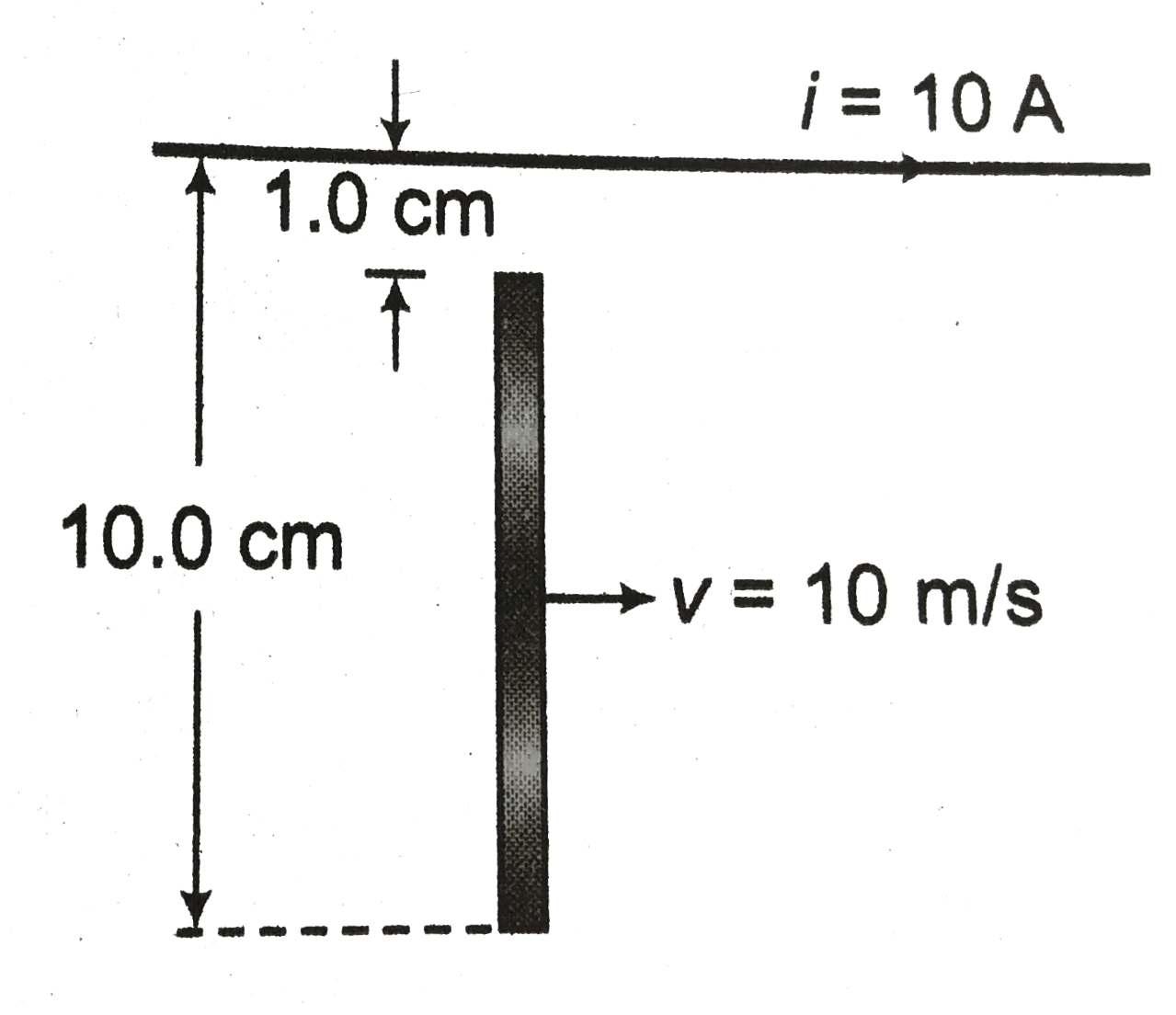
In figure, a wire perpendicular to a long straight wire is moving parallel to the later with a speed v=10m/s in the direction of the current flowing in the later. The current is 10A. What is the magnitude of the potential difference between the ends of the moving wire?
View SolutionThe electric potential at a point, due to a positive charge of 100 µC distance of 9m, is (a) 10^4 V (b) 10^6 V (c) 10^5 V (d) 10^7 V
View SolutionThe electric potential at a point, due to a positive charge of 100 µC distance of 9m, is (a) 10^4 V (b) 10^6 V (c) 10^5 V (d) 10^7 V
View Solution(a) Define the term 'conductivity' of a metallic wire. Write its SI unit.
(b) Using the concept of free electrons in a conductor, derive the expression for the conductivity of a wire in te'rms of number density and relaxation time. Hence obtain the relation between current density and the applied electric field E.
View SolutionTwo long wires are placed parallel to each other with a distance 1 m between them. The wires carry equal current 1 A in the same direction. The magnetic field intensity at a point P which is equidistant from the wires is 1.6×10−7T. Then, the distance of this point from any one of the wires is _________ m.
[Permeability of vacuum, μ0=4π×10−7T−m/A]
View SolutionIn the circuit shown if a conducting wire is connected between points A and B, the current in this wire will :-

View SolutionIn the circuit shown if a conducting wire is connected between points A and B, the current in this wire will :-
View Solution
