A thermally insulated container is divided into two parts by a screen. In one part the pressure and temperature are P and T for an ideal gas filled. In the second part it is vacuum. If now a small hole is created in the screen, then the temperature of the gas will
The correct Answer is:Remain same
To solve the problem step-by-step, we can follow these logical steps:
Step 1: Understand the System
We have a thermally insulated container divided into two parts. One side contains an ideal gas at pressure P and temperature T, while the other side is a vacuum.
Hint: Identify the key elements of the system: the ideal gas, vacuum, and the thermally insulated nature of the container.
Step 2: Analyze the Process
When a small hole is created in the screen, the gas will rush into the vacuum. This process is known as free expansion, which occurs without any external pressure acting on the gas.
Hint: Recall the characteristics of free expansion: it is spontaneous and occurs without doing work on the surroundings.
Step 3: Apply the First Law of Thermodynamics
The First Law of Thermodynamics states:
ΔU=Q−W
where ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system.
In this case:
- The container is thermally insulated, so Q=0 (no heat transfer).
- Since the gas expands into a vacuum, no work is done, so W=0.
Thus, we have:
ΔU=0−0=0
Hint: Remember that in a thermally insulated system with no work done, the internal energy remains constant.
Step 4: Relate Internal Energy to Temperature
For an ideal gas, the internal energy U is a function of temperature T only. Therefore, if the internal energy does not change (ΔU=0), it implies that the temperature must also remain constant.
Hint: Recall that for an ideal gas, internal energy is solely dependent on temperature, not on pressure or volume.
Step 5: Conclude the Result
Since the internal energy of the gas remains constant during the free expansion, we conclude that the temperature of the gas after it expands into the vacuum will also remain the same as it was initially.
Final Answer: The temperature of the gas will remain T.
---
We have a thermally insulated container divided into two parts. One side contains an ideal gas at pressure P and temperature T, while the other side is a vacuum.
When a small hole is created in the screen, the gas will rush into the vacuum. This process is known as free expansion, which occurs without any external pressure acting on the gas.
The First Law of Thermodynamics states:
ΔU=Q−W
where ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system.
- The container is thermally insulated, so Q=0 (no heat transfer).
- Since the gas expands into a vacuum, no work is done, so W=0.
ΔU=0−0=0
For an ideal gas, the internal energy U is a function of temperature T only. Therefore, if the internal energy does not change (ΔU=0), it implies that the temperature must also remain constant.
Since the internal energy of the gas remains constant during the free expansion, we conclude that the temperature of the gas after it expands into the vacuum will also remain the same as it was initially.
Topper's Solved these Questions
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 19
1 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 20
1 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 17
1 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
Similar Questions
A container of volume V is divided into two equal parts by a screen. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the screen is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA container with insulating walls is divided into two equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated . If the valve is suddenly opened, the pressure and temperature of the gas will be
View SolutionA container with insulating walls is divided into two equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated . If the valve is suddenly opened, the pressure and temperature of the gas will be
View SolutionA vessel of volume V is divided into two parts by a partition. The pressure of the gas in one part is p1 and that in the other part is p2. If the partition is removed find the pressure of the gas in the vessel.
View SolutionAn insulated container is divided into two equal portions. One portion contains as ideal gas at pressure P and tenperature T. The othe portion is a perfect vaccume. If a hole is opened between the two portions, claculate
a. the change in internal energy.
b. the change in temperature.
View SolutionAn ideal gas is found to obey the law V2T=constant. The initial pressure and temperature are P0 and T0. If gas expands such that its pressure becomes P04, then the final temperature of the gas will be
View SolutionA container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
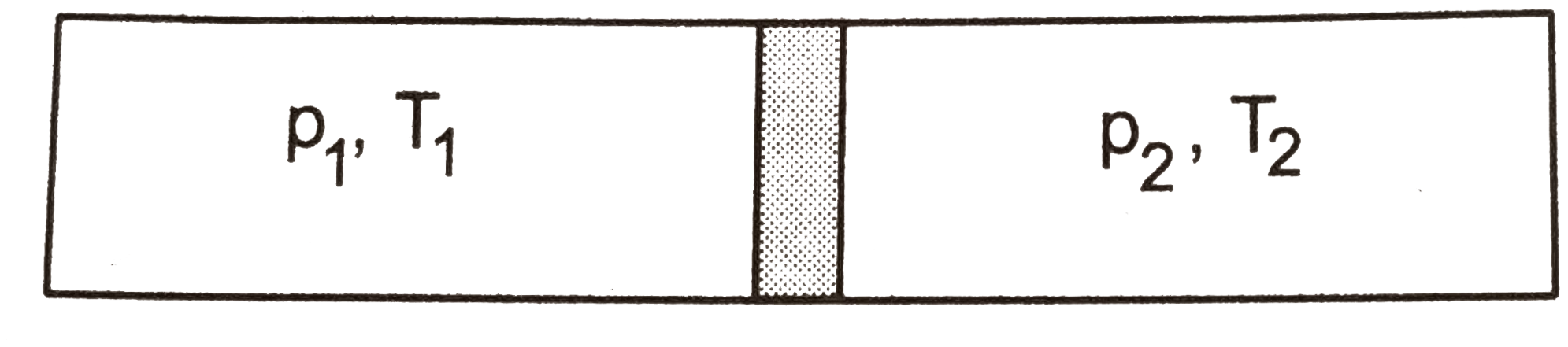
View SolutionFig shows a cylindrical tube of volume V with adiabatic walls containing an ideal gas. The internal energy of this ideal gas is given by 1.5nRT. The tube is divided into two equal parts by a fixed diathermic wall. Initially, the pressure and the temperature are p1,T1 on the left and p2,T2 on the right. the system is left for sufficient time so that the temperature becomes equal on the two sides. (a) how much work has been done by the gas on the left part ? (b) find the final pressures on the two sides. (c ) find the final equilibrium temperature. (d) how much heat has flown from the gas on the right to the gas on the left ?
View SolutionA closed gas cylinder is divided into two parts by a piston held tight. The pressure and volume of gas in two parts respectively are (P, 5V) and (10P, V). If now the piston is left free and the system undergoes isothermal process, then the volumes of the gas in two parts respectively are
View Solution
