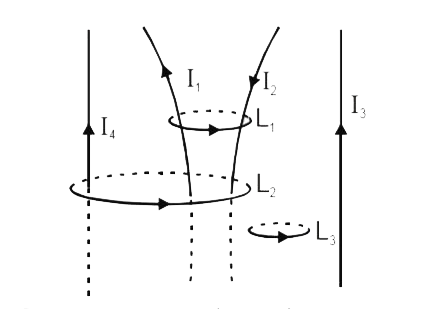
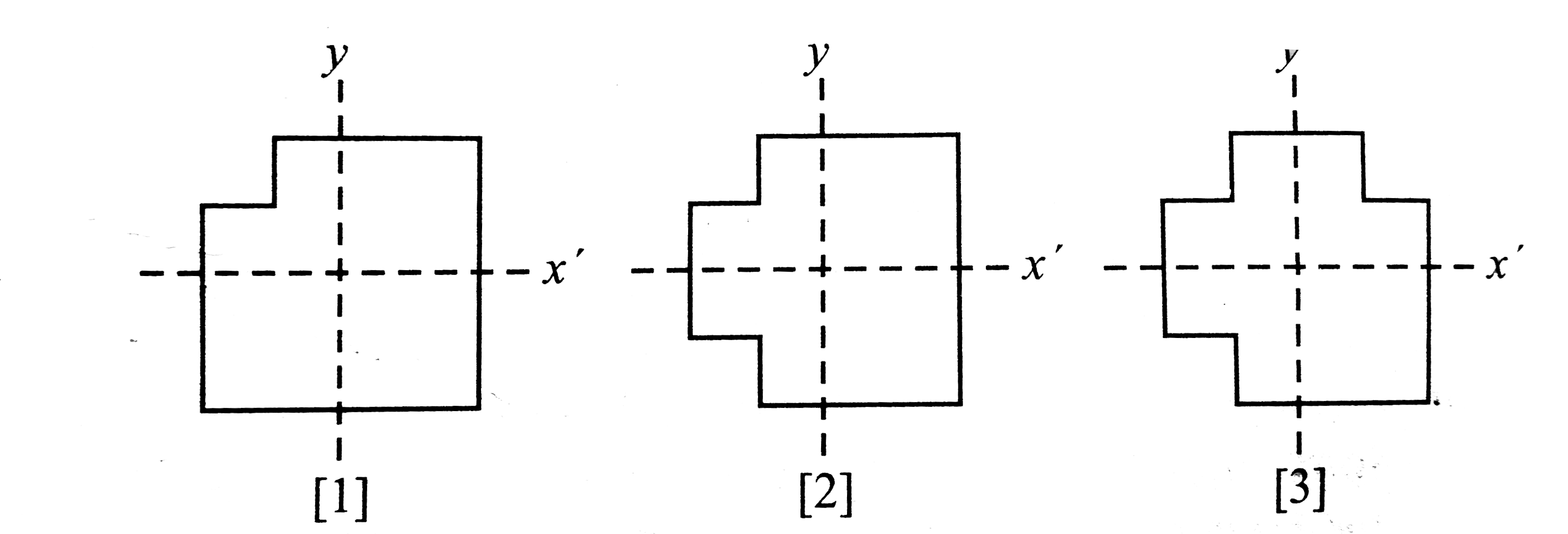
Rank the value of ∮→B→dl for the closed paths shown in figure from the smallest to largest.
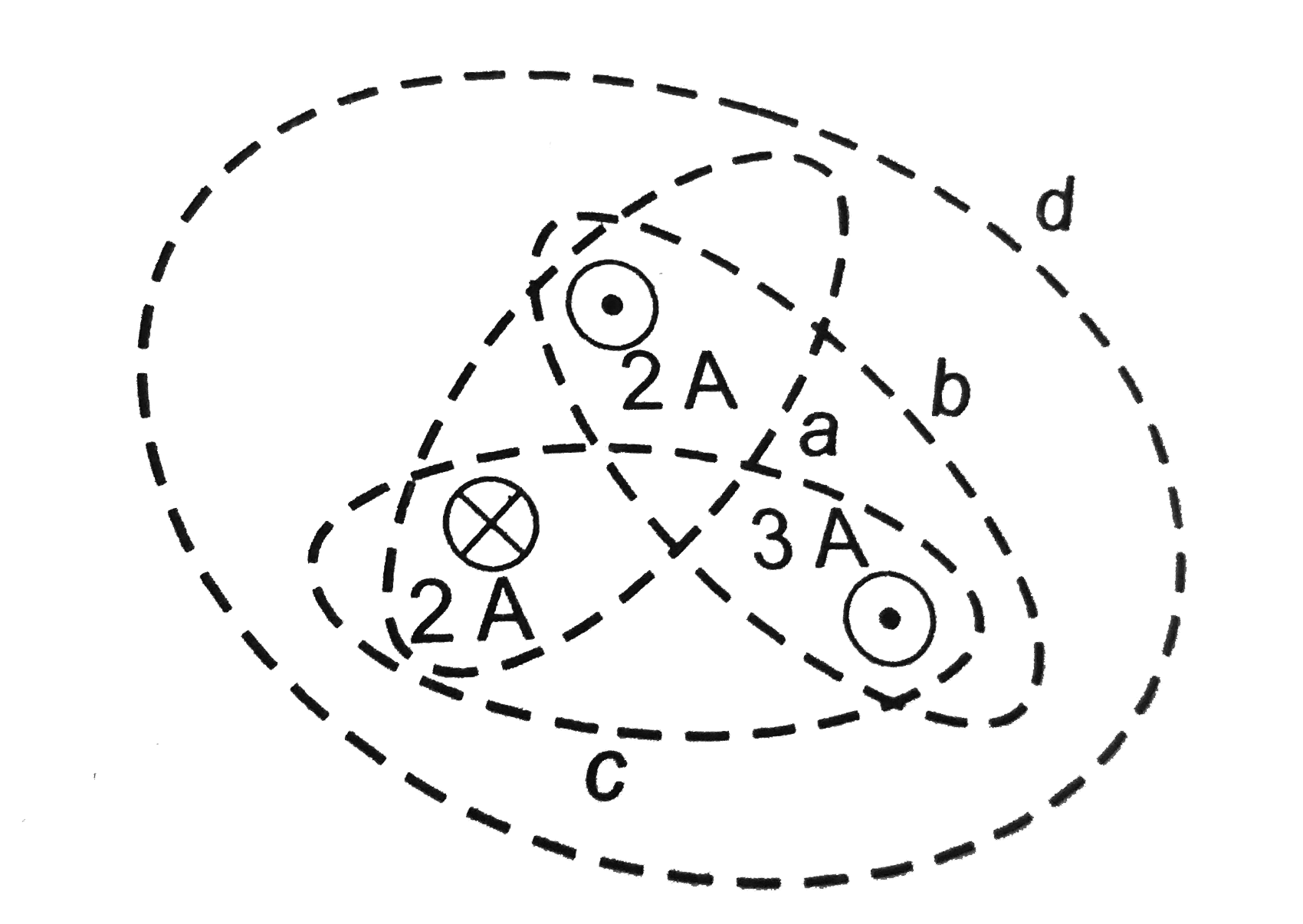
a,b,c,d
a,c,b,d,
a,d,c,d
a,c,b,d
The correct Answer is:B
Step by step video & image solution for Rank the value of ointvec(B)vec(dl) for the closed paths shown in figure from the smallest to largest. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Answer Type
35 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type
14 Videos
Similar Questions
Knowledge Check
Rank the bond dissociation energies of the bonds indicated with the arrows. (from smallest to largest).
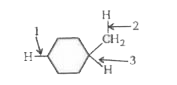 A1<2<3B3<2<1C2<3<1D3<1<2
A1<2<3B3<2<1C2<3<1D3<1<2
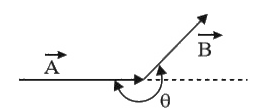
What is the angle between the vector →A and →B as shown in figure ?
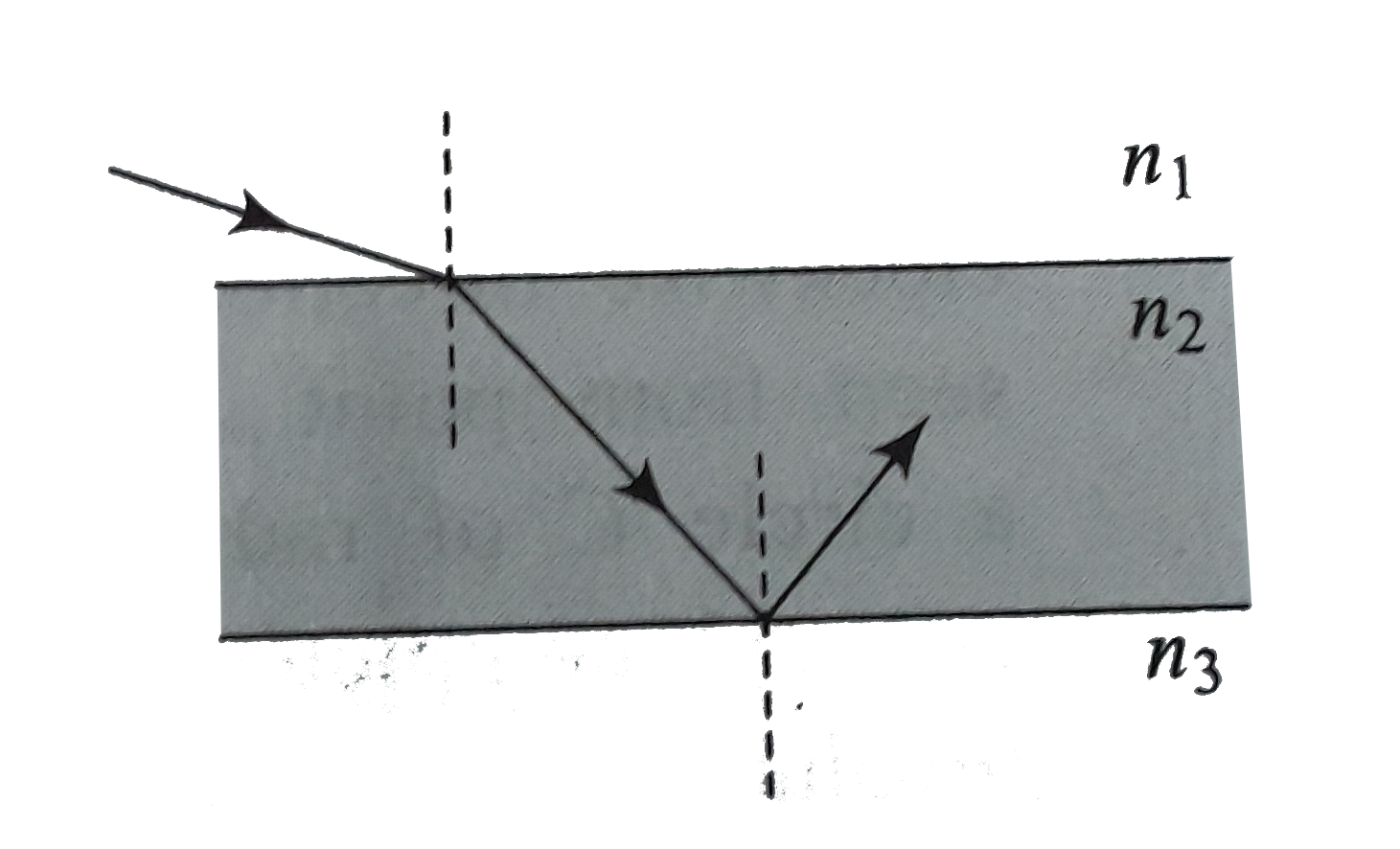
View SolutionLight traveling trhought three transparent substances follows the path shown in figure. Arrange the indices of refraction in order from smallest to largest. Not the total internal reflection does occur on the bottom surface of medium 2.
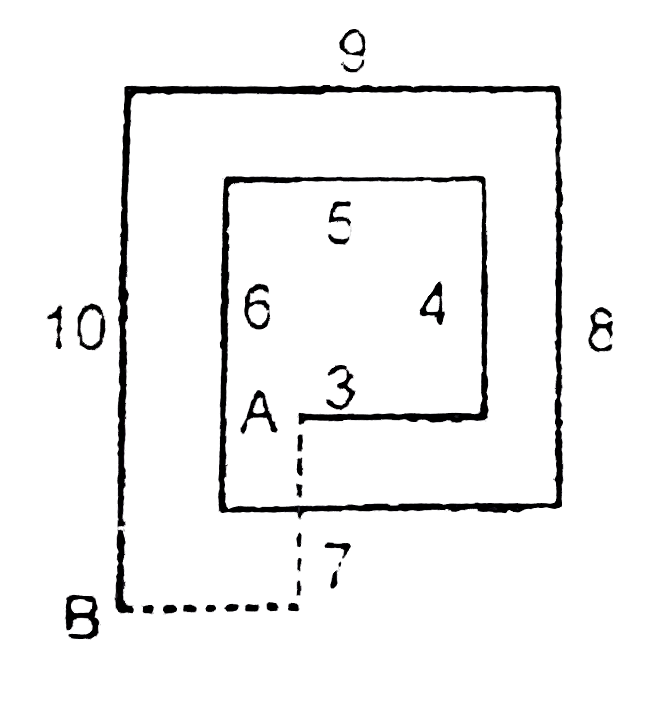
View SolutionA particle travels from A to B path shown in figure, then the displacement of particle is :
 .
.
View SolutionCurrent I0 is flowing through a bent wire a→c→b→d in z−x plane as shown in figure, then ∮→B.→dl over the loop PQRS lying in the X-Y plane as shown in figure, due to the bent wire acbd

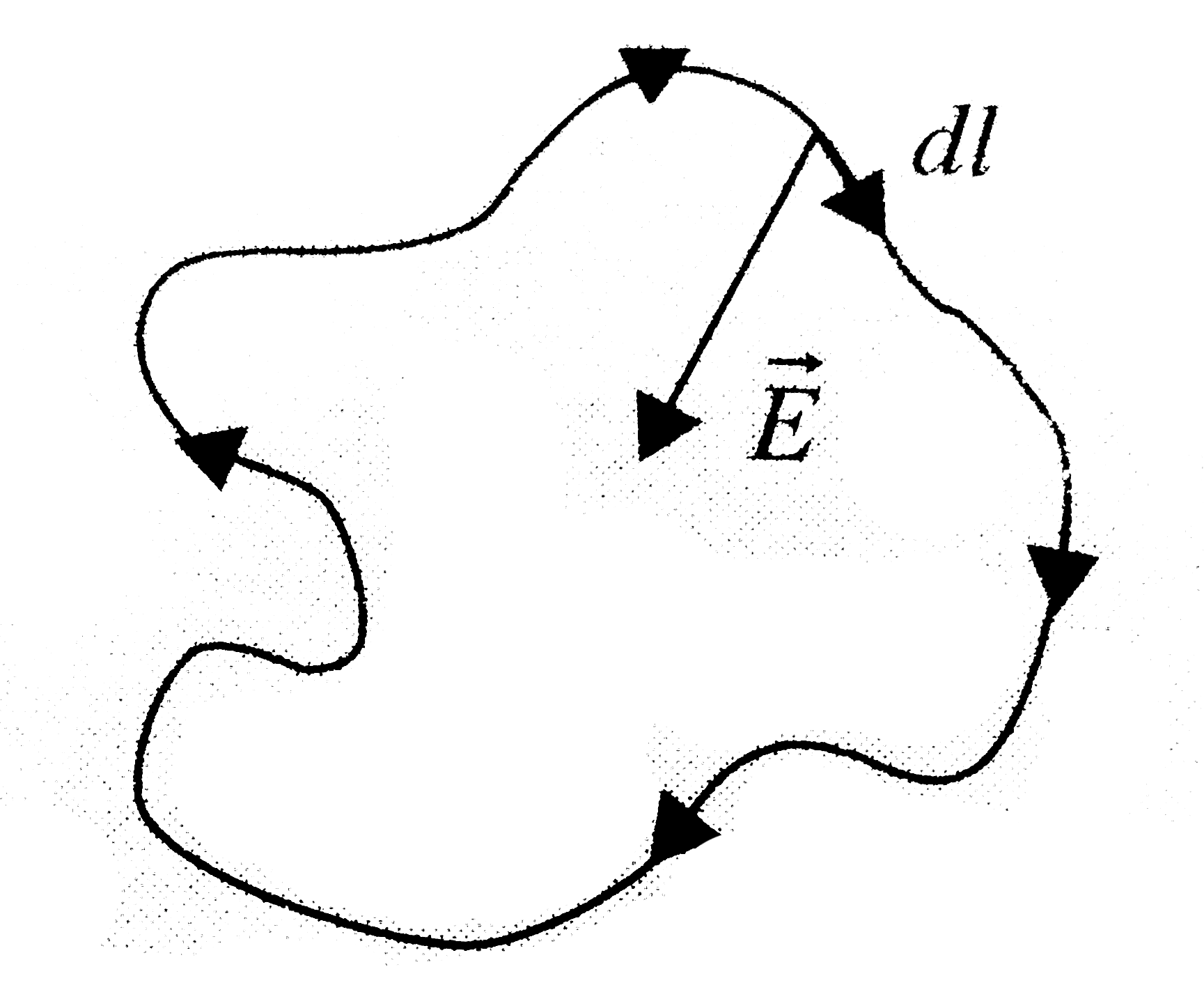
View SolutionIf you carry out the integral of the electric field ∫→E.→dl for a closed path like that as shown in (Fig. 3.40), the integral will always be equal tom zero, independent of the shape of the path and independent of where charges may be located relative to the path. Explain why.
 .
.
View SolutionA machinist starts with three identical square plates but cuts one corner from one of them, two corners from the second and three corners from the third. Rank the three according to the x-coordinate of their centre of mass, from smallest to largest.
View SolutionA: In any magnetic field region the line integral ∮→B.→dl along a closed loop is always zero.
R: The magnetic field →B in the expressioin ∮→B.→dl is due to the currents enclosed only by the loop.
View Solution