A dye obsorbs light of λ=4530Å and then flaorescences light of 5000Å .Assuming that uinder given condition 47% of the obserbed energy is re-emited out so flaurescence , calculate the ratio of quants emitted out to the number of quanta obserbed
To solve the problem step by step, we will follow these calculations:
Step 1: Understand the Relationship Between Energy and Wavelength
The energy of a photon can be calculated using the formula:
E=hcλ
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- c is the speed of light (3×108m/s),
- λ is the wavelength of the light in meters.
Step 2: Calculate the Energy Absorbed
The dye absorbs light of wavelength λabsorbed=4530Å=4530×10−10m.
Using the energy formula:
Eabsorbed=hcλabsorbed
Step 3: Calculate the Energy Emitted
The dye fluoresces light of wavelength λemitted=5000Å=5000×10−10m.
Using the energy formula:
Eemitted=hcλemitted
Step 4: Relate the Energies with the Number of Quanta
Let n1 be the number of quanta absorbed and n2 be the number of quanta emitted. The total energy absorbed can be expressed as:
Eabsorbed=n1⋅Eabsorbed
The total energy emitted can be expressed as:
Eemitted=n2⋅Eemitted
Step 5: Use the Given Information
According to the problem, 47% of the absorbed energy is re-emitted. Therefore:
Eemitted=0.47⋅Eabsorbed
Step 6: Set Up the Equation
From the above relationships, we can write:
n2⋅Eemitted=0.47⋅n1⋅Eabsorbed
Step 7: Substitute the Energy Expressions
Substituting the expressions for Eabsorbed and Eemitted:
n2⋅hcλemitted=0.47⋅n1⋅hcλabsorbed
Step 8: Cancel Out Common Terms
Since hc appears on both sides, we can cancel it out:
n2⋅1λemitted=0.47⋅n1⋅1λabsorbed
Step 9: Rearrange to Find the Ratio
Rearranging gives:
n2n1=0.47⋅λemittedλabsorbed
Step 10: Substitute the Wavelengths
Substituting the values:
n2n1=0.47⋅5000Å4530Å
Step 11: Calculate the Ratio
Calculating the numerical value:
n2n1=0.47⋅50004530≈0.518
Final Answer
Thus, the ratio of quanta emitted out to the number of quanta absorbed is approximately:
n2n1≈0.518
---
The energy of a photon can be calculated using the formula:
E=hcλ
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- c is the speed of light (3×108m/s),
- λ is the wavelength of the light in meters.
The dye absorbs light of wavelength λabsorbed=4530Å=4530×10−10m.
Using the energy formula:
Eabsorbed=hcλabsorbed
The dye fluoresces light of wavelength λemitted=5000Å=5000×10−10m.
Using the energy formula:
Eemitted=hcλemitted
Let n1 be the number of quanta absorbed and n2 be the number of quanta emitted. The total energy absorbed can be expressed as:
Eabsorbed=n1⋅Eabsorbed
The total energy emitted can be expressed as:
Eemitted=n2⋅Eemitted
According to the problem, 47% of the absorbed energy is re-emitted. Therefore:
Eemitted=0.47⋅Eabsorbed
From the above relationships, we can write:
n2⋅Eemitted=0.47⋅n1⋅Eabsorbed
Substituting the expressions for Eabsorbed and Eemitted:
n2⋅hcλemitted=0.47⋅n1⋅hcλabsorbed
Since hc appears on both sides, we can cancel it out:
n2⋅1λemitted=0.47⋅n1⋅1λabsorbed
Rearranging gives:
n2n1=0.47⋅λemittedλabsorbed
Substituting the values:
n2n1=0.47⋅5000Å4530Å
Calculating the numerical value:
n2n1=0.47⋅50004530≈0.518
Thus, the ratio of quanta emitted out to the number of quanta absorbed is approximately:
n2n1≈0.518
Topper's Solved these Questions
- ATOMIC STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercises (Subjective)
52 Videos
- ATOMIC STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercises Linked Comprehension
50 Videos
- APPENDIX - INORGANIC VOLUME 1BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - APPENDIX - INORGANIC VOLUME 1EXERCISE - chapter-7 Single correct answer
1 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective
15 Videos
Similar Questions
A certain day absorbs lights of λ=400 nm and then fluorescence light of wavelength 500 mn. Assuming that under given condition 40% of the absorbed energy is re-emitted as fluorescence, calculate the ratio of quanta obserbed to number of quanta emitted out.
View SolutionA certain dye absorbs light of cerain wavelength and then fluorescence light of wavelength 5000 Å.Assuming that under given conditions , 50% of the absorbed energy is re-emitted out as fluorescence and the ratio of number of quanta emitted out to the number of quanta absorbed is 5:8, find the wavelength of absorbed light (in Å) : [hc=12400 eVÅ]
View SolutionA certain dye absorbs 4000 Å and fluoresces at 8000 Å. These being wavelengths of maximum absorption that under given conditions 50 % of the absorbed energy is emitted. Calculate the ratio of the no. of quanta emitted to the number absorbed.
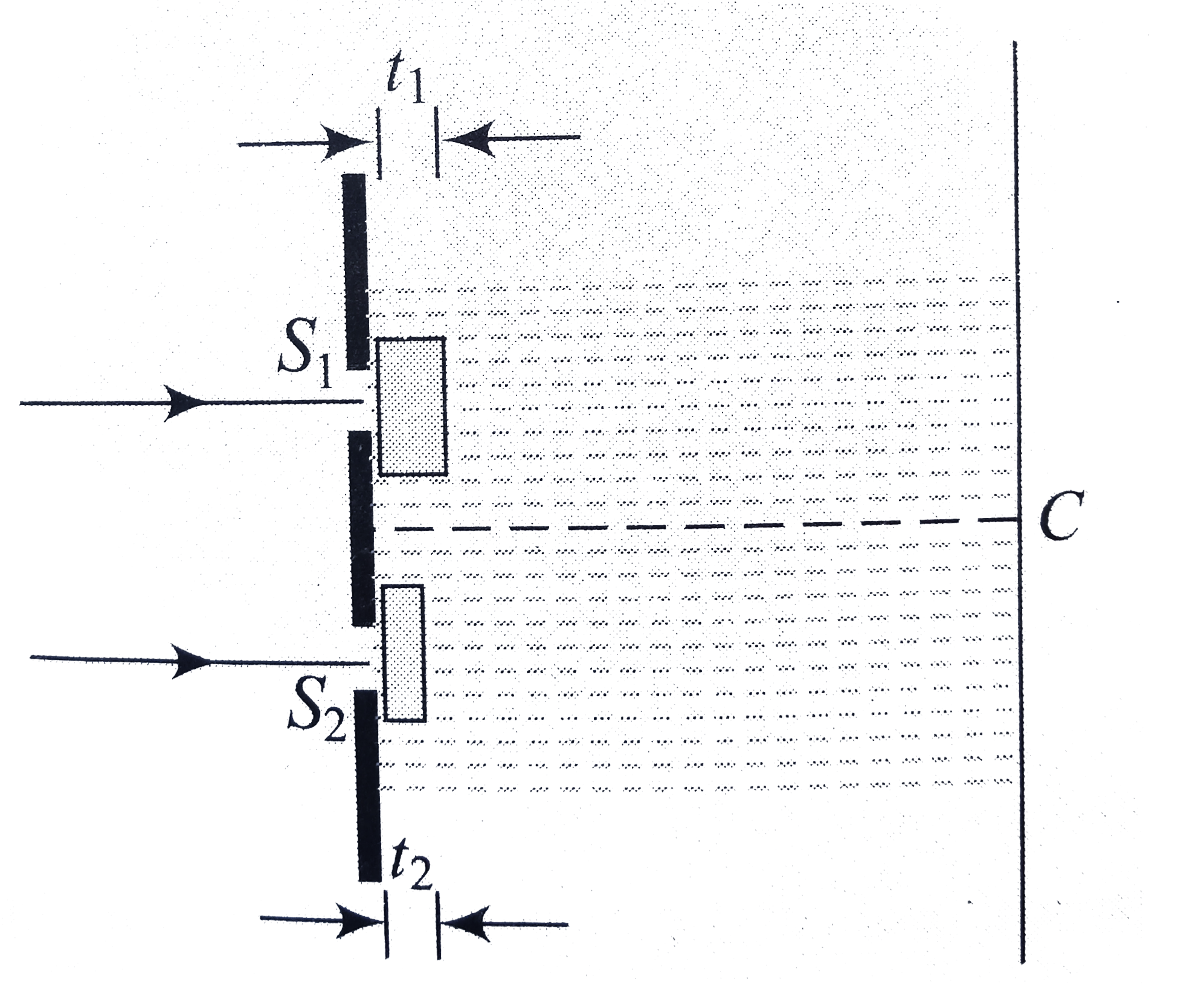
View SolutionA screen is at distance D=80 cm form a diaphragm having two narrow slits S1 and S2 which are d=2 mm apart.
Slit S1 is covered by a transparent sheet of thickness
t1=2.5μm slit S2 is covered by another sheet of thickness
t2=1.25μm as shown if Fig. 2.52.
Both sheets are made of same material having refractive index μ=1.40
Water is filled in the space between diaphragm and screen. A monochromatic light beam of wavelength λ=5000Å is incident normally on the diaphragm.
Assuming intensity of beam to be uniform, calculate ratio of intensity of C to maximum intensity of interference pattern obtained on the screen (μw=4/3)
View SolutionLight of wavelength 5000 Å and intensity of 3.96×10−3wa/cm2 is incident on the surface of photo metal . If 1% of the incident photons only emit photo electrons , then the number of electrons emitted per unit area per second from the surface will be
View SolutionThe only element in the hydrogen atom resides under ordinary condition on the first orbit .When energy is supplied the element move to hjgher energy ornbit depending on the lower of energy absioerbed .When this electron to may of the electron return to any of the lower orbits, it emit energy Lyman series is formed when the electron to the lowest orbit white Balmer series ids formed when the electron returns to the second orbit similar Paschen Brackett, and Pfund series are formed when electron return to the third fourth , and fifth arbit from highest energy orbits, respectively
Maximum number of liner produced is equal when as electron jumps from nth level to ground level is equal to n(n−1)2If teh electron comes back from the energy level having energy E2 to the energy level having energy E1 then the difference may be expresent in terms of energy of photon as E2−E1=ΔE,λ=hc/ΔE Since h and c are constants ΔE coresponding to definite energy , thus , each transition from one energy level to unother will produce a light of definite wavelem=ngth .This isd actually observed as a line in the spectrum of hydrogen atom Wave number of line is given by the formula ¯v=RZ2(1n21−1n212)Where R is a Rydherg constant
The wave number of electromagnetic radiation emitted during the transition of electron in between the two levels of Li2+ ion whose pricipal quantum numbner sum is 4 and difference is 2 is
View SolutionThe only element in the hydrogen atom resides under ordinary condition on the first orbit .When energy is supplied the element move to hjgher energy ornbit depending on the lower of energy absioerbed .When this electron to may of the electron return to any of the lower orbits, it emit energy Lyman series is formed when the electron to the lowest orbit white Balmer series ids formed when the electron returns to the second orbit similar Paschen Brackett, and Pfund series are formed when electron return to the third fourth , and fifth arbit from highest energy orbits, respectively
Maximum number of liner produced is equal when as electron jumps from nth level to ground level is equal to n(n−1)2If teh electron comes back from the energy level having energy E2 to the energy level having energy E1 then the difference may be expresent in terms of energy of photon as E2−E1=ΔE,λ=hc/ΔE Since h and c are constants ΔE coresponding to definite energy , thus , each transition from one energy level to unother will produce a light of definite wavelem=ngth .This isd actually observed as a line in the spectrum of hydrogen atom Wave number of line is given by the formula ¯v=RZ2(1n21−1n212)Where R is a Rydherg constant
Its a single isolated atom, an electrons make transition from fifth excited state is second thern maximum number of different type of photon observed is
View SolutionThe only element in the hydrogen atom resides under ordinary condition on the first orbit .When energy is supplied the element move to hjgher energy ornbit depending on the lower of energy absioerbed .When this electron to may of the electron return to any of the lower orbits, it emit energy Lyman series is formed when the electron to the lowest orbit white Balmer series ids formed when the electron returns to the second orbit similar Paschen Brackett, and Pfund series are formed when electron return to the third fourth , and fifth arbit from highest energy orbits, respectively
Maximum number of liner produced is equal when as electron jumps from nth level to ground level is equal to n(n−1)2If teh electron comes back from the energy level having energy E2 to the energy level having energy E1 then the difference may be expresent in terms of energy of photon as E2−E1=ΔE,λ=hc/ΔE Since h and c are constants ΔE coresponding to definite energy , thus , each transition from one energy level to unother will produce a light of definite wavelem=ngth .This isd actually observed as a line in the spectrum of hydrogen atom Wave number of line is given by the formula ¯v=RZ2(1n21−1n212)Where R is a Rydherg constant
If the ionisation potential for hydrogen -like atom in a sample is 122.4V then the series limit of the paschen series for this atom is
View SolutionThe only element in the hydrogen atom resides under ordinary condition on the first orbit .When energy is supplied the element move to hjgher energy ornbit depending on the lower of energy absioerbed .When this electron to may of the electron return to any of the lower orbits, it emit energy Lyman series is formed when the electron to the lowest orbit white Balmer series ids formed when the electron returns to the second orbit similar Paschen Brackett, and Pfund series are formed when electron return to the third fourth , and fifth arbit from highest energy orbits, respectively
Maximum number of liner produced is equal when as electron jumps from nth level to ground level is equal to n(n−1)2If teh electron comes back from the energy level having energy E2 to the energy level having energy E1 then the difference may be expresent in terms of energy of photon as E2−E1=ΔE,λ=hc/ΔE Since h and c are constants ΔE coresponding to definite energy , thus , each transition from one energy level to unother will produce a light of definite wavelem=ngth .This isd actually observed as a line in the spectrum of hydrogen atom Wave number of line is given by the formula ¯v=RZ2(1n21−1n212)Where R is a Rydherg constant
The difference in the wavelength of the second line is Lyman series and last line of breaker series is a hydrogen sample is
View SolutionCalculate the number of photons emitted per second by a 10 W sodium vapour lamp. Assume that 60% of the consumed energy is converted into light. Wavelengths of sodium light =590nm.
View Solution
