If w1.w2,w3 and w4 are work done in isothermal, adiabatic, isobaric, and isochoric reversible expansion for an ideal gas, respectively, then
w3>w1
w1>w2
w2>w4
w4>w2
To solve the problem regarding the work done in different types of reversible expansions for an ideal gas, we can analyze each process step by step.
1. Understanding Work Done in Thermodynamic Processes:
The work done w in a thermodynamic process can be represented as the area under the pressure-volume (P-V) curve for that process. The type of process determines the shape of the P-V curve and thus the area (work done).
2. Isothermal Process (w1):
In an isothermal process, the temperature remains constant. For an ideal gas, the work done during isothermal expansion can be calculated using the formula:
w1=nRTln(VfVi)
where n is the number of moles, R is the universal gas constant, T is the temperature, Vf is the final volume, and Vi is the initial volume.
3. Adiabatic Process (w2):
In an adiabatic process, no heat is exchanged with the surroundings. The work done can be expressed as:
w2=PiVi−PfVfγ−1
where γ is the heat capacity ratio (Cp/Cv). The area under the adiabatic curve is less than that of the isothermal curve because the gas does work without heat input.
4. Isobaric Process (w3):
In an isobaric process, the pressure remains constant. The work done is given by:
w3=PΔV=P(Vf−Vi)
This process typically covers the largest area under the P-V curve since the pressure is constant while the volume changes.
5. Isochoric Process (w4):
In an isochoric process, the volume remains constant, meaning no work is done:
w4=0
This results in the smallest work done, as there is no area under the curve.
6. Comparing Work Done:
Now, we can compare the work done in each process:
- w3 (isobaric) > w1 (isothermal) > w2 (adiabatic) > w4 (isochoric).
- Therefore, the order of work done is:
w3>w1>w2>w4
Final Answer:
The correct order of work done in the processes is:
w3>w1>w2>w4
The work done w in a thermodynamic process can be represented as the area under the pressure-volume (P-V) curve for that process. The type of process determines the shape of the P-V curve and thus the area (work done).
In an isothermal process, the temperature remains constant. For an ideal gas, the work done during isothermal expansion can be calculated using the formula:
w1=nRTln(VfVi)
where n is the number of moles, R is the universal gas constant, T is the temperature, Vf is the final volume, and Vi is the initial volume.
In an adiabatic process, no heat is exchanged with the surroundings. The work done can be expressed as:
w2=PiVi−PfVfγ−1
where γ is the heat capacity ratio (Cp/Cv). The area under the adiabatic curve is less than that of the isothermal curve because the gas does work without heat input.
In an isobaric process, the pressure remains constant. The work done is given by:
w3=PΔV=P(Vf−Vi)
This process typically covers the largest area under the P-V curve since the pressure is constant while the volume changes.
In an isochoric process, the volume remains constant, meaning no work is done:
w4=0
This results in the smallest work done, as there is no area under the curve.
Now, we can compare the work done in each process:
- w3 (isobaric) > w1 (isothermal) > w2 (adiabatic) > w4 (isochoric).
- Therefore, the order of work done is:
w3>w1>w2>w4
The correct order of work done in the processes is:
w3>w1>w2>w4
Topper's Solved these Questions
- THERMODYNAMICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Multiple correct Answer Type
2 Videos
- THERMODYNAMICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Exercises (Single Correct)
62 Videos
- THERMODYNAMICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Exercises (Linked Comprehension)
74 Videos
- STOICHIOMETRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective
33 Videos
Similar Questions
The pressure-volume of varies thermodynamic process is shown in graphs:
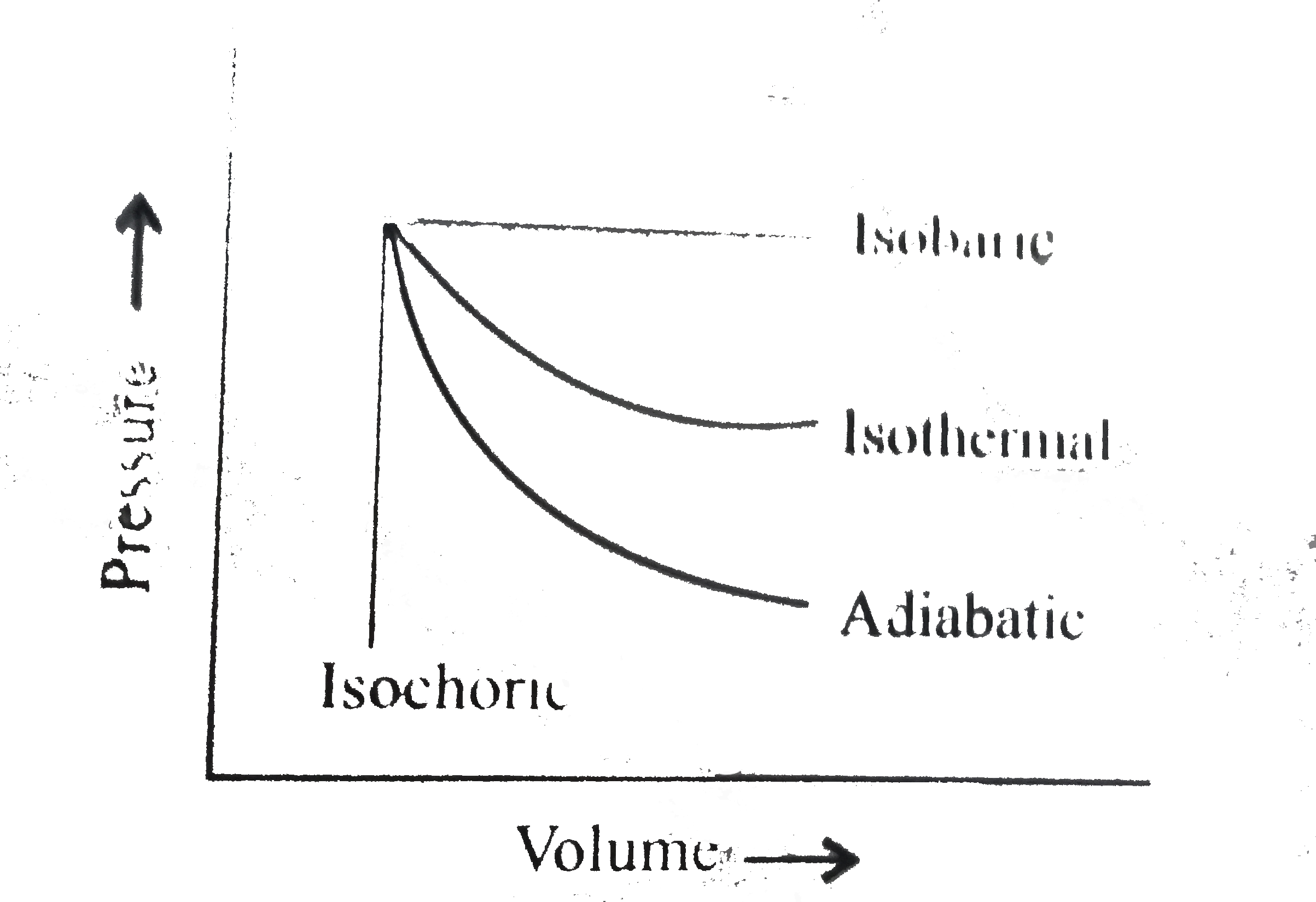
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
If w1,w2,w3 and w4 are work done in isothermal, adiabatic, isobaric, and isochoric reversible processes, respectively then the correct sequence (for expansion) would be
View SolutionIf w1,w2,w3 and w4 for an ideal gas are magnitude of work done in isothermal, adiabatic, isobaric and isochoric reversible expansion processes, the correct order will be :
View SolutionIn reversible isothermal expansion of an ideal gas :
View SolutionThe pressure-volume of varies thermodynamic process is shown in graphs:
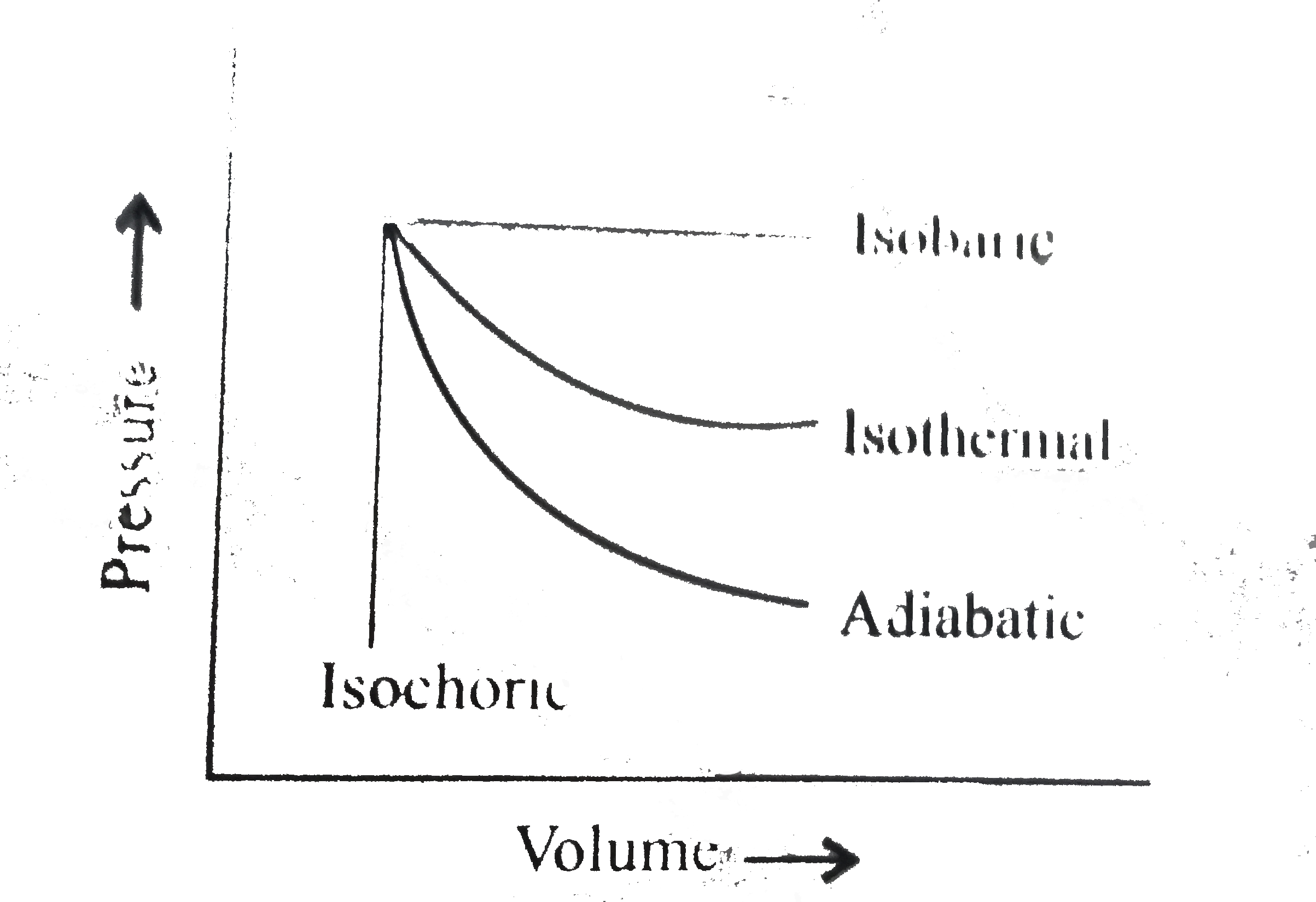
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
The q value and work done in isothermal reversible expansion of one mole of an ideal gas from initial pressure of 1 bar to final pressure of 0.1 bar at constant temperature 273K are:
View SolutionCalculate the work done during isothermal reversible expansion expansion of one mol of an ideal gas from 10 atm to 1 atm at 300K.
View SolutionCalculate the work done during isothermal reversible expansion expansion of one mol of an ideal gas from 10 atm to 1 atm at 300K.
View SolutionIf W1W2 and W3 represent the work done in moving a particle from A to B along three different paths 1.2 and3 respectively (asshown ) in the gravitational fieled of a point mass m, find the correct relation between W_(1) W_(2)andW_(3)
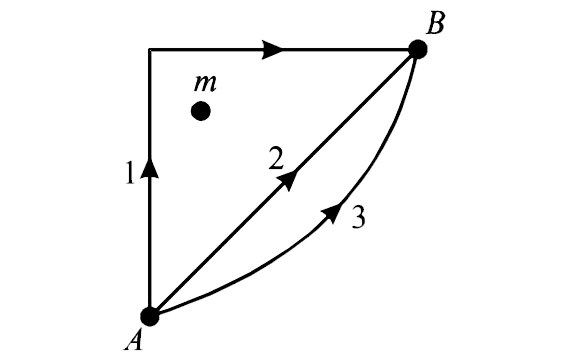
View SolutionIf W1W2 and W3 represent the work done in moving a particle from A to B along three different paths 1.2 and3 respectively (asshown ) in the gravitational fieled of a point mass m, find the correct relation between W_(1) W_(2)andW_(3)

View SolutionThe work done by 100 calorie of heat in isothermal expansion of ideal gas is :-
View SolutionWork done in the isothermal reversible expansion of a gas is always greater than that done in isothermal irreversible expansion of the gas.
View Solution
