In which of the following systeams interfacial angles but ?
Monoclinic
Rhombohedral
Triclinic
Hexagonal
The correct Answer is:A, B
To solve the question, we need to analyze the interfacial angles of different crystal systems and identify which one satisfies the conditions given: α = γ = 90° and β ≠ 90°.
1. Understanding Crystal Systems:
We need to recall the definitions of the interfacial angles in different crystal systems. The main systems to consider are monoclinic, rhombohedral, triclinic, and hexagonal.
2. Monoclinic System:
- In the monoclinic system, the interfacial angles are defined as:
- α = 90°
- β ≠ 90°
- γ = 90°
- This satisfies the condition α = γ = 90° and β ≠ 90°.
3. Rhombohedral System:
- In the rhombohedral system, the interfacial angles are:
- α = β = γ ≠ 90°
- This does not satisfy the condition since all angles are equal and not equal to 90°.
4. Triclinic System:
- In the triclinic system, the interfacial angles are:
- α ≠ β ≠ γ
- None of the angles are equal to 90°, which does not satisfy the condition.
5. Hexagonal System:
- In the hexagonal system, the interfacial angles are:
- α = β = 90°
- γ = 120°
- This does not satisfy the condition since γ is not equal to 90°.
6. Conclusion:
- After analyzing all the systems, the only one that satisfies the condition α = γ = 90° and β ≠ 90° is the monoclinic system.
Final Answer:
The correct answer is monoclinic.
We need to recall the definitions of the interfacial angles in different crystal systems. The main systems to consider are monoclinic, rhombohedral, triclinic, and hexagonal.
- In the monoclinic system, the interfacial angles are defined as:
- α = 90°
- β ≠ 90°
- γ = 90°
- This satisfies the condition α = γ = 90° and β ≠ 90°.
- In the rhombohedral system, the interfacial angles are:
- α = β = γ ≠ 90°
- This does not satisfy the condition since all angles are equal and not equal to 90°.
- In the triclinic system, the interfacial angles are:
- α ≠ β ≠ γ
- None of the angles are equal to 90°, which does not satisfy the condition.
- In the hexagonal system, the interfacial angles are:
- α = β = 90°
- γ = 120°
- This does not satisfy the condition since γ is not equal to 90°.
- After analyzing all the systems, the only one that satisfies the condition α = γ = 90° and β ≠ 90° is the monoclinic system.
The correct answer is monoclinic.
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Single Correct)72videos
SOLID STATE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Assertion-Reasoning)19videos
SOLID STATE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Linked Comprehension)13videos
SOLID STATE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSEXERCISE - SUBJECTIVE TYPE4videos
REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDS
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Ex 2.3 (Objective)9videos
SOLUTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The crystal system for which a≠b≠candα=β=γ=90∘ is said to be
ACubicBTetragonalCOrthorhombicDHexagonal
The unit cell with crystallographic dimensions, a≠b≠c,α=γ=90∘andβ≠90∘ is :
View Solutionif a=b≠c and α=β=γ=90∘, the crystal system is
View SolutionExample of unit cell with crystallographic dimensions a≠b≠c,α=γ=90∘,β≠90∘ is
View SolutionAssertion (A) : Graphite is an example of tetragonal crystal system.
Reason (R ) : For a tetragonal system, a=b≠c and α=β=90∘,γ=120∘.View SolutionIf cosα+cosβ+cosγ=0=sinα+sinβ+sinγ, then which of the following is/are true:- (a)cos(α−β)+cos(β−γ)+cos(γ−δ)=−32 (b)cos(α−β)+cos(β−γ)+cos(γ−δ)=−12 (c)∑cos2α+2cos(α+β)+2cos(β+γ)+2cos(γ+α)=0 (d)∑sin2α+2sin(α+β)+2sin(β+γ)+2sin(γ+α)=0
View SolutionThe following diagram shows the arrangement of lattice points with a=b=c and α=β=γ=90∘. Choose the correct options.
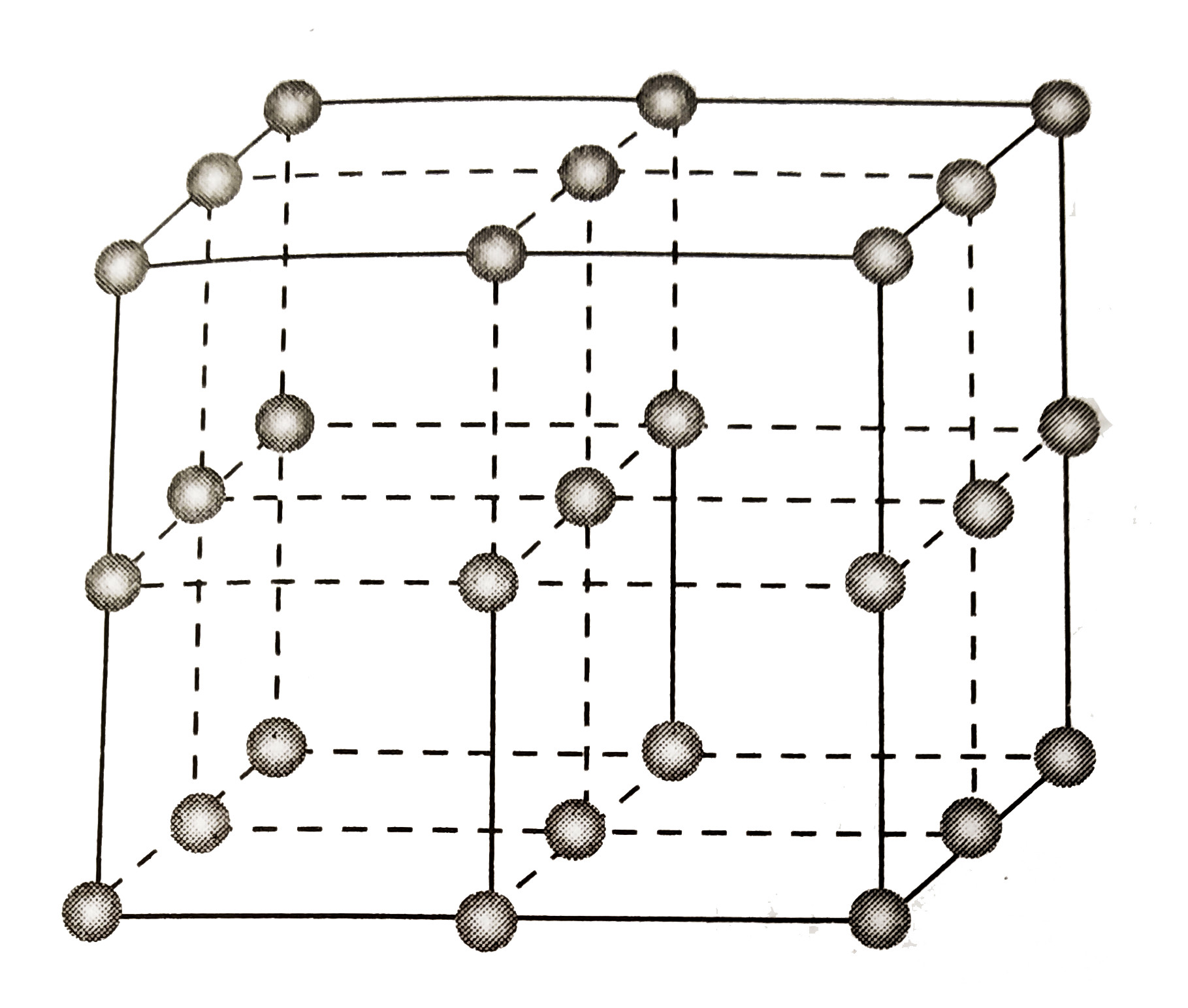 View Solution
View SolutionIf α,βandγ the roots of the equation x3+3x2−4x−2=0.
then find the values of the following expressions:
(i) α2+β2+γ2
(ii)α3+β3+γ3
(iii) 1α+1β+1γView Solution

