How many kinds of space lattices are possible in a crystal?
23
7
230
14
The correct Answer is:D
To determine how many kinds of space lattices are possible in a crystal, we can categorize them based on their crystal systems. Here’s a step-by-step solution:
Step 1: Identify the Crystal Systems
There are seven types of crystal systems in crystallography:
1. Triclinic
2. Monoclinic
3. Orthorhombic
4. Tetragonal
5. Rhombohedral
6. Hexagonal
7. Cubic
Step 2: Count the Space Lattices for Each Crystal System
Now, we will count the number of distinct space lattices for each of these crystal systems:
- Triclinic: 1 space lattice
- Monoclinic: 2 space lattices
- Orthorhombic: 4 space lattices
- Tetragonal: 2 space lattices
- Rhombohedral: 1 space lattice
- Hexagonal: 1 space lattice
- Cubic: 3 space lattices
Step 3: Sum the Total Number of Space Lattices
Now, we will add the number of space lattices from each crystal system:
- Triclinic: 1
- Monoclinic: 2
- Orthorhombic: 4
- Tetragonal: 2
- Rhombohedral: 1
- Hexagonal: 1
- Cubic: 3
Total = 1 + 2 + 4 + 2 + 1 + 1 + 3 = 14
Conclusion
Thus, the total number of kinds of space lattices possible in a crystal is 14.
There are seven types of crystal systems in crystallography:
1. Triclinic
2. Monoclinic
3. Orthorhombic
4. Tetragonal
5. Rhombohedral
6. Hexagonal
7. Cubic
Now, we will count the number of distinct space lattices for each of these crystal systems:
- Triclinic: 1 space lattice
- Monoclinic: 2 space lattices
- Orthorhombic: 4 space lattices
- Tetragonal: 2 space lattices
- Rhombohedral: 1 space lattice
- Hexagonal: 1 space lattice
- Cubic: 3 space lattices
Now, we will add the number of space lattices from each crystal system:
- Triclinic: 1
- Monoclinic: 2
- Orthorhombic: 4
- Tetragonal: 2
- Rhombohedral: 1
- Hexagonal: 1
- Cubic: 3
Thus, the total number of kinds of space lattices possible in a crystal is 14.
Topper's Solved these Questions
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Assertion-Reasoning)
19 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Interger)
9 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Multiple Correct)
39 Videos
- REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSEXERCISE - SUBJECTIVE TYPE
4 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Ex 2.3 (Objective)
9 Videos
Similar Questions
Define space lattice.
View SolutionDefine term space lattice ?
View SolutionHow many types of Bravais lattices can occur in crystalline solids?
View SolutionHow many resonance structures are possible for allyl carbocation?
View SolutionHow many geometrical isomers are possible for

View SolutionHow many resonance structures are possible for allyl carbocation?
View SolutionHow many kinds of nitrogenous bases are present in nucleic acids?
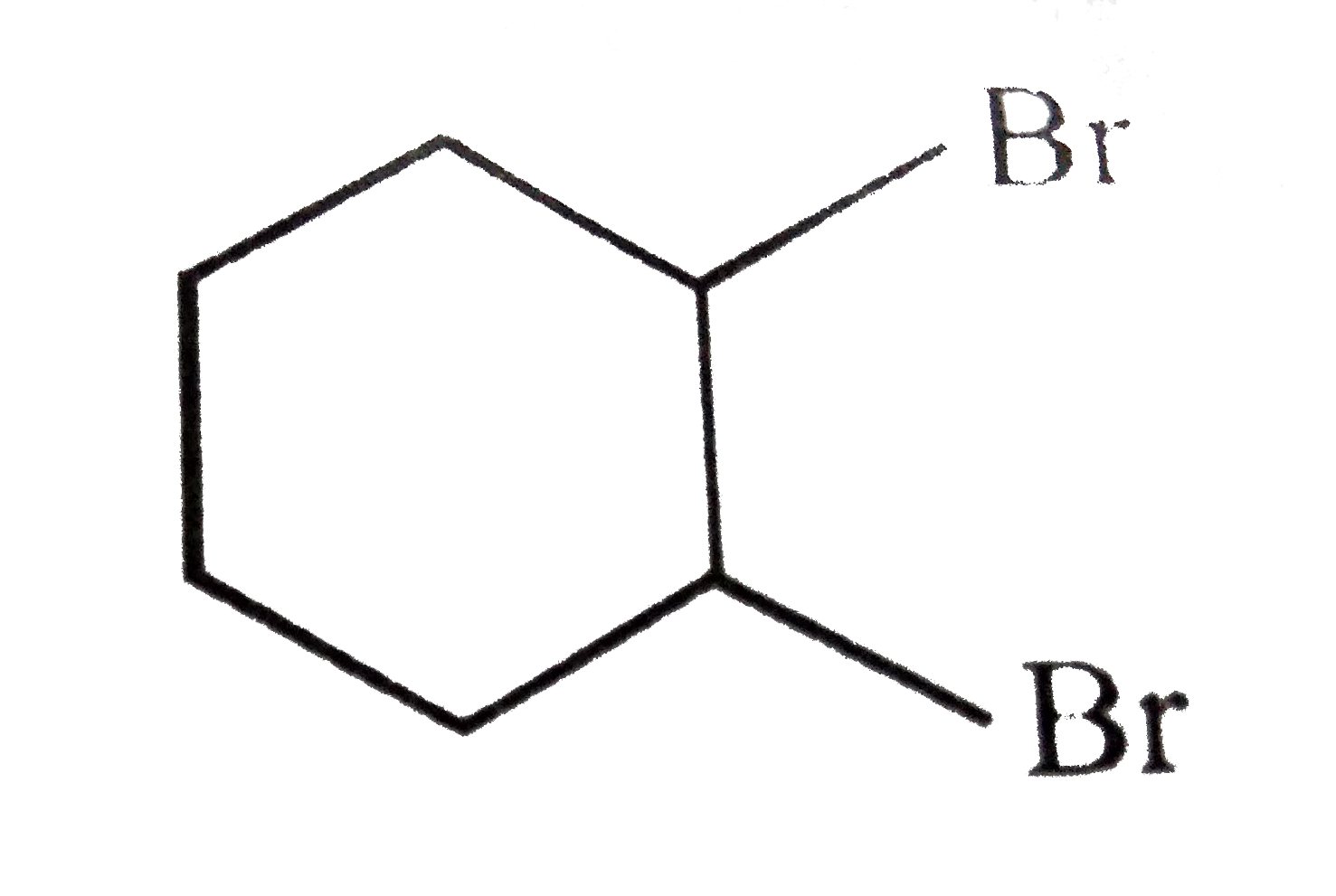
View SolutionHow many stereoisomers are possible in this compound ?
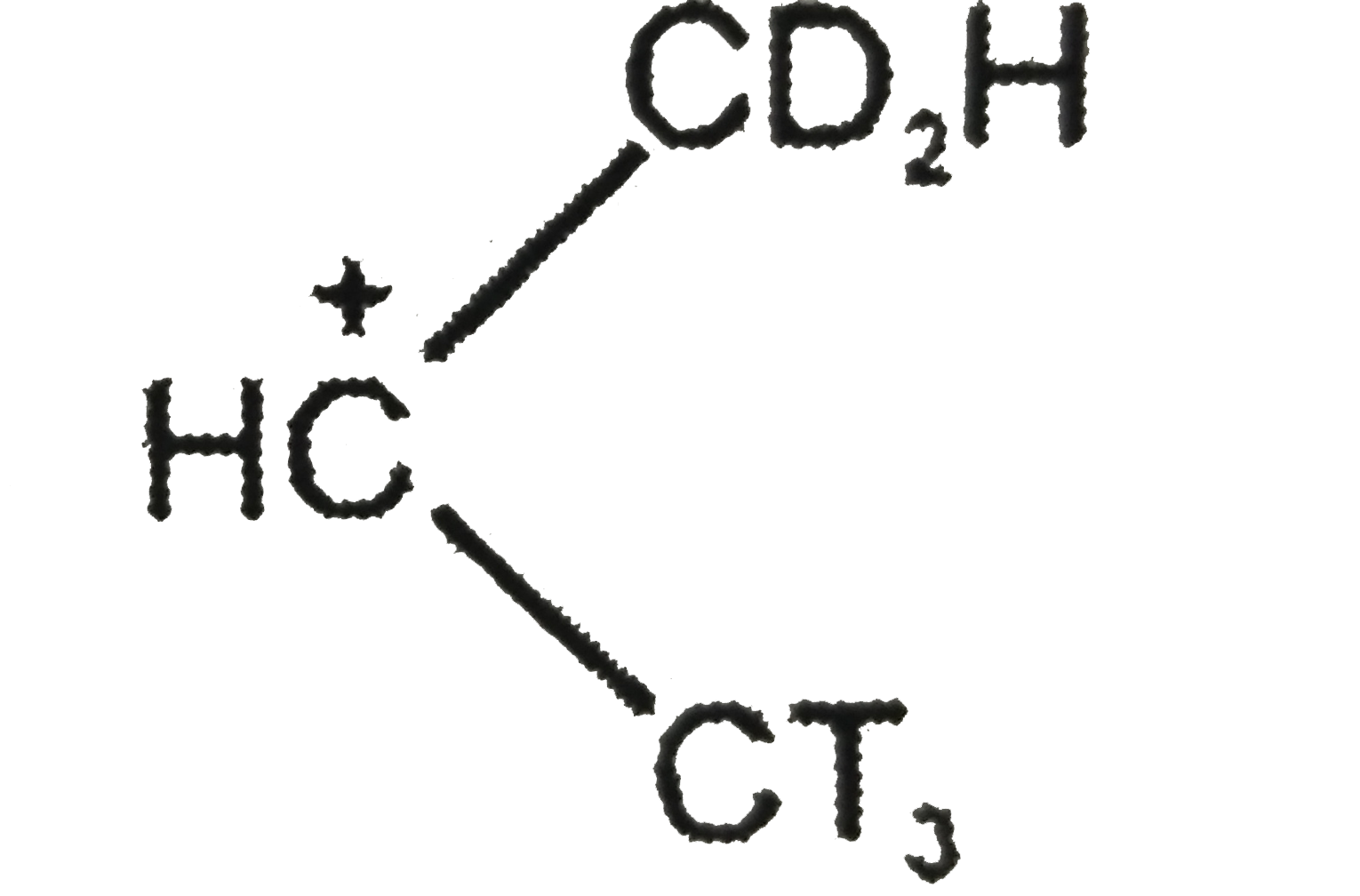
View SolutionHow many hperconjugative structures are possible in the following carbocation ?
View SolutionHow many sawhorse projections of ethane are possible ?
View Solution
