The range of radius ratio (cationic to anionic) for an octahedral arrangement of ions in an ionic solid is
0.155−0.225
0.225−0.414
0.414−0.732
0.732−1.000
The correct Answer is:C
To determine the range of the radius ratio (cationic to anionic) for an octahedral arrangement of ions in an ionic solid, we can follow these steps:
1. Understand the Radius Ratio Rule:
The radius ratio rule relates the size of cations (positively charged ions) to anions (negatively charged ions) in an ionic solid. It is defined as the ratio of the radius of the cation (R⁺) to the radius of the anion (R⁻). This can be expressed as:
Radius Ratio=R+R−
2. Identify the Range for Octahedral Coordination:
For an octahedral arrangement of ions, the radius ratio has specific limits. The critical values for the radius ratio that define the stability of the octahedral voids are:
- The lower limit is approximately 0.414.
- The upper limit is approximately 0.732.
3. Establish the Range:
Therefore, the range of the radius ratio for an octahedral arrangement can be expressed as:
0.414<R+R−<0.732
4. Conclusion:
Based on the above analysis, the range of the radius ratio (cationic to anionic) for an octahedral arrangement in an ionic solid is from 0.414 to 0.732.
Final Answer:
The range of radius ratio (cationic to anionic) for an octahedral arrangement of ions in an ionic solid is 0.414 to 0.732.
---
The radius ratio rule relates the size of cations (positively charged ions) to anions (negatively charged ions) in an ionic solid. It is defined as the ratio of the radius of the cation (R⁺) to the radius of the anion (R⁻). This can be expressed as:
Radius Ratio=R+R−
For an octahedral arrangement of ions, the radius ratio has specific limits. The critical values for the radius ratio that define the stability of the octahedral voids are:
- The lower limit is approximately 0.414.
- The upper limit is approximately 0.732.
Therefore, the range of the radius ratio for an octahedral arrangement can be expressed as:
0.414<R+R−<0.732
Based on the above analysis, the range of the radius ratio (cationic to anionic) for an octahedral arrangement in an ionic solid is from 0.414 to 0.732.
The range of radius ratio (cationic to anionic) for an octahedral arrangement of ions in an ionic solid is 0.414 to 0.732.
Topper's Solved these Questions
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Assertion-Reasoning)
19 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Interger)
9 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercises (Multiple Correct)
39 Videos
- REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSEXERCISE - SUBJECTIVE TYPE
4 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - SOLUTIONSEXERCISE - Ex 2.3 (Objective)
9 Videos
Similar Questions
Knowledge Check
IF the radius ratio of cation to anion is in the range of 0.225 - 0.414 , then the coordination number of cation will be _________.
A3, plane triangularB6,octahedralC4,tetrahedralD8,cubic
Process of separation of ions of an ionic solid in solution
View SolutionThe cation/anion radius ratio for a triangular arrangement of anions in which the cation is in contact with the anions (but does not push them apart) as shown in the figure is
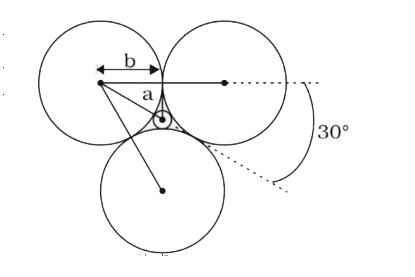
View SolutionIf the value of ionic radius ratio (rcra) is 0.52 in an ionic compound , the geometrical of ions in crystal is
View SolutionIs the conductivity of ionic solids due to the presence of ions ?
View SolutionThe cations and anions are arranged n alternate form in:
View SolutionThe ratio of cationic radius to anionic radius in an ionic crystal is greater than 0.732 its coordination number is
View SolutionCalculate the coordination number in an ionic crystal, when the radius ratio of cation to anion is 0.3
View Solution
