A solution of cane sugar having dextrorotation of inverted by lactic acid to at . The rotations determined are as follows:
Show that the inverison of sugar is a unimolecular reaction.
To show that the inversion of sugar is a unimolecular reaction, we will analyze the given data and calculate the rate constant (k) for the reaction at different time intervals. A unimolecular reaction is characterized by a first-order rate law, which means the rate of reaction depends on the concentration of a single reactant.
1. Identify the Given Data:
- Initial rotation (R₀) = 34.50°
- Final rotation (R∞) = -10.77° (at completion of the reaction)
- Concentration of lactic acid = 0.5 N
- Temperature = 298 K
- Time and rotation data:
| Time (min) | Rotation (°) |
|------------|---------------|
| 0 | 34.50 |
| 14.55 | 31.10 |
| 111.36 | 13.98 |
| ∞ | -10.77 |
2. Calculate the Rate Constant (k) for Each Time Interval:
The formula for the first-order reaction is:
k=2.303tlog(R0−R∞Rt−R∞)
where:
- R0 = initial rotation
- Rt = rotation at time t
- R∞ = final rotation
- t = time in minutes
3. Calculate k for t = 14.55 min:
- Rt=31.10°
k=2.30314.55log(34.50−(−10.77)31.10−(−10.77))
=2.30314.55log(45.2741.87)
=2.30314.55log(1.0805)
=2.30314.55×0.0339
k≈0.0053min−1
4. Calculate k for t = 111.36 min:
- Rt=13.98°
k=2.303111.36log(34.50−(−10.77)13.98−(−10.77))
=2.303111.36log(45.2724.75)
=2.303111.36log(1.826)
=2.303111.36×0.261
k≈0.0053min−1
5. Conclusion:
Since the calculated value of k is consistent (0.0053 min⁻¹) for both time intervals, we can conclude that the reaction follows first-order kinetics. Therefore, the inversion of sugar is a unimolecular reaction.
- Initial rotation (R₀) = 34.50°
- Final rotation (R∞) = -10.77° (at completion of the reaction)
- Concentration of lactic acid = 0.5 N
- Temperature = 298 K
- Time and rotation data:
| Time (min) | Rotation (°) |
|------------|---------------|
| 0 | 34.50 |
| 14.55 | 31.10 |
| 111.36 | 13.98 |
| ∞ | -10.77 |
The formula for the first-order reaction is:
k=2.303tlog(R0−R∞Rt−R∞)
where:
- R0 = initial rotation
- Rt = rotation at time t
- R∞ = final rotation
- t = time in minutes
- Rt=31.10°
k=2.30314.55log(34.50−(−10.77)31.10−(−10.77))
=2.30314.55log(45.2741.87)
=2.30314.55log(1.0805)
=2.30314.55×0.0339
k≈0.0053min−1
- Rt=13.98°
k=2.303111.36log(34.50−(−10.77)13.98−(−10.77))
=2.303111.36log(45.2724.75)
=2.303111.36log(1.826)
=2.303111.36×0.261
k≈0.0053min−1
Since the calculated value of k is consistent (0.0053 min⁻¹) for both time intervals, we can conclude that the reaction follows first-order kinetics. Therefore, the inversion of sugar is a unimolecular reaction.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Solved Example
52 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex 4.1 (Objective)
9 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
Methyl acetate was subjected to hydrolyiss in N−HCl at 298K. 5mL of the mixture is withdraw at different intervals and titrated with constant with about N/8NaOH. The following results were obtained:
∣∣∣Time (min)0254061∞Vol of alkali used (mL)19.2424.2026.6029.3242.03∣∣∣
Show that the reaction is of first order.
View SolutionA 10%(w/w) solution of cane sugar has undergone partial inversion according to the reaction:
sucrose+water→glucose+ fructose. If the boiling point of solution is 100.27∘C.
(a) What is the average mass of the dissolved materials?
(b) What fraction of the sugar has inverted? Kb(H2O)=0.512 K mol−1 kg
View SolutionA student performs an experiment to determine how the range of a ball depends on the velocity with which it is projected. The "range" is the distance between the points where the ball lends and from where it was projected, assuming it lands at the same height from which it was projected. It each trial, the student uses the same baseball, and launches it at the same angle. Table shows the experimental results.
∣∣ ∣ ∣ ∣ ∣ ∣∣TrailLaunch speed(m/s)Range(m)110822031.833070.7440122.5∣∣ ∣ ∣ ∣ ∣ ∣∣
Based on this data, the student then hypothesizes that the range, R, depends on the initial speed v0 according to the following equation : R=Cvn0, where C is a constant and n is another constant.
The student performs another trial in which the ball is launched at speed 5.0m/s. Its range is approximately:
View SolutionThe first order reaction:
Sucrose → Glucose + Fructose takes place at 308K in 0.5NHCl. At time zero the initial total rotation of the mixture is 32.4∘. After 10min, the total rotation is 28.8∘. If the rotation of sucrose per mole is 85∘, that of glucose is 7.4∘, and of fructose is −86.04∘, calculate the half life of the reaction.
View SolutionA 50 mL solution of weak base BOH is titrated with 0.1NHCI solution. The pH of solution is found to be 10.04 and 9.14 after the addition of 5.0mL and 20.0 mL of acid respectively. Find out Kb for weak base.
View SolutionFigure shows a steel rod joined to a brass rod. Each of the rods has length of 31cm and area of cross-section 0.20cm2. The junction is maintained at a constant temperature 50∘C and the two ends are maintained at 100∘C. The amount of heat taken out from the cold junction in 10 minutes after the steady state is reached in n×102J. Find 'n' the thermal conductivities are Ksteel=46W/m−∘C and Kbrass=109W/m−∘C.
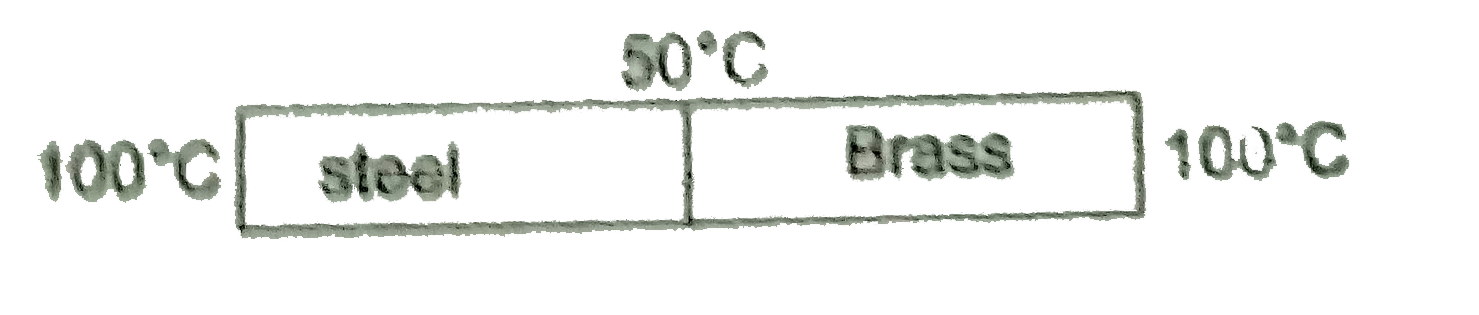
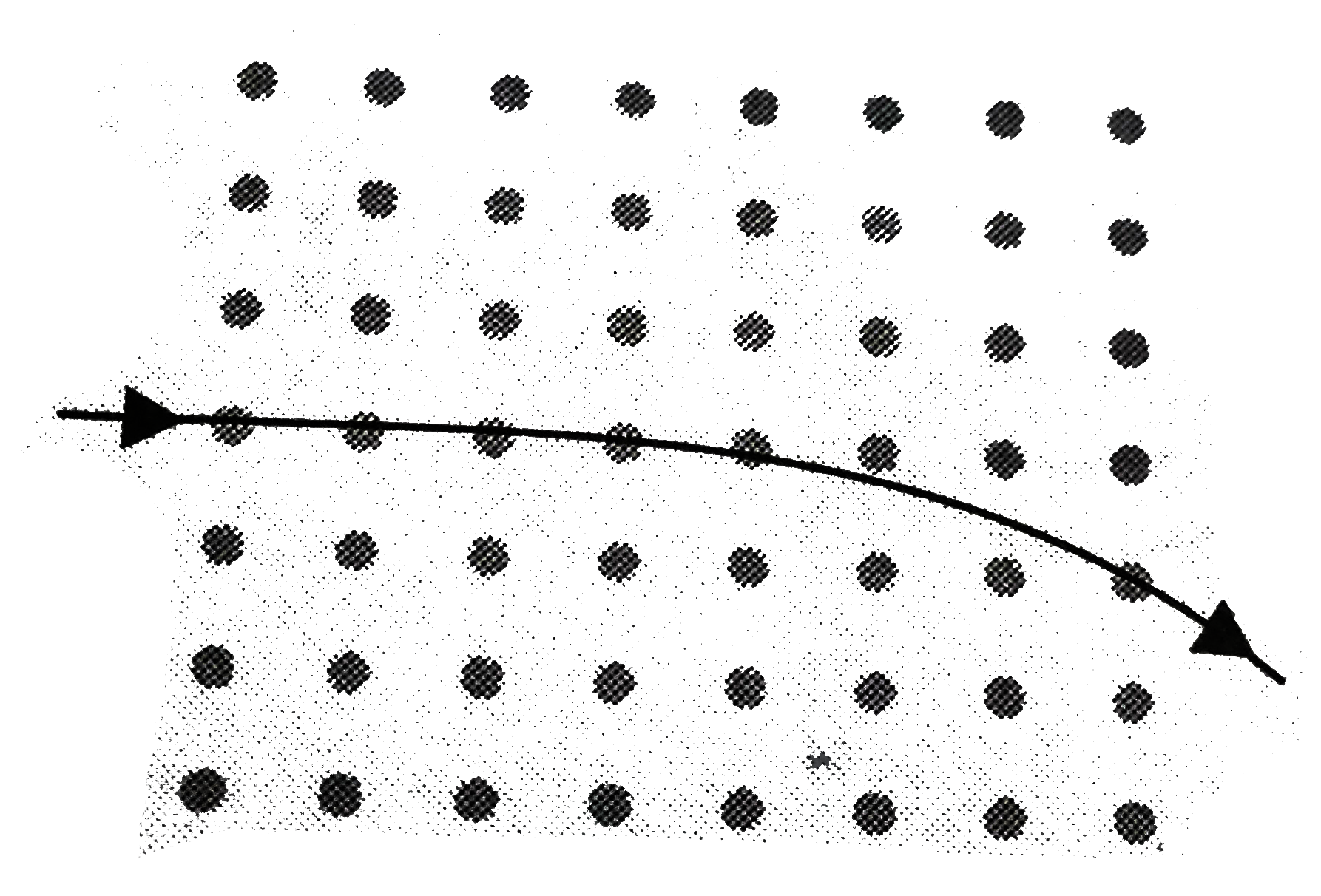
View SolutionProtons having a kinetic energy of 50 eV are moving in the positive x-direction and enter a magnetic field B=0.5mˆkT directed out of the plane of the page and extending from x=0→x=1m as shown in Fig. 1.34.
a. Calculate the y-component of the protons' momentum as they leave the magnetic field.
b. Find the angle ϕ between the initial velocity vector of the proton beam and the velocity vector after the beam emerges from the field. Ignore relativistic effects and note that 1eV=1.60×10−19J.
View SolutionFigure shows water in a container having 2.0−mm thick walls made of a material of thermal conductivity 0.50Wm−1C−1 . The container is kept in a melting-ice bath at 0∘C . The total surface area in contact with water is0.05m2 . A wheel is clamped inside the water and is coupled to a block of mass M as shown in the figure. As the goes down, the wheel rotates. It is found that after some time a steady state is reached in which the block goes down with a constant speed of 10cms−1 and the temperature of the water remains constant at 1.0∘C .Find the mass M of the block. Assume that the heat flow out of the water only through the walls in contact. Take g=10ms−2 .

View SolutionThe initial concentration of N2O5 in the following first order reaction:
N2O5(g)→2NO2(g)+12O2(g)
was 1.24×10−2molL−1 at 318K. The concentration of N2O5 after 60min was 0.20×10−2molL−1. Calculate the rate constant of the reaction at 318K.
View SolutionA 4.00kg block carrying a charge Q=50.0μC is connected to a spring for which k = 100 N/ m. The block lies on a frictionless horizontal track, and the system is immersed in a uniform electric field of magnitude E=5.00×105Vm, directed as shown in figure. If the block is released from rest when the spring is unstretched (at x = 0).
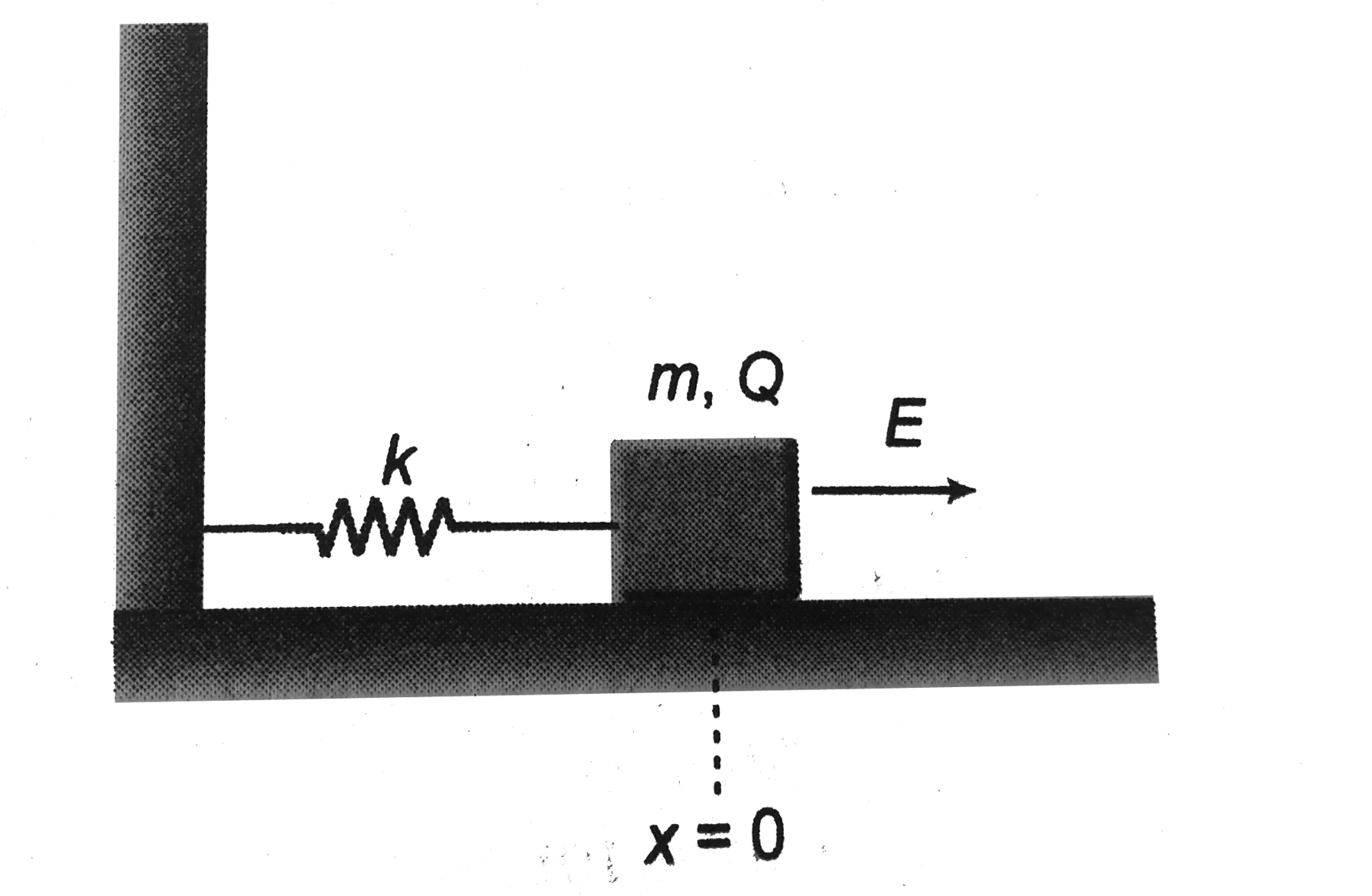
(a) By what maximum amount does the spring expand?
(b) What is the equilibrium position of the block?
(c) Show that the block's motion is simple harmonic and determine its period.
(d) Repeat part (a) if the coefficient of kinetic friction between block and surface is 0.2.
View Solution
